Solving Literal Equations Made Easy

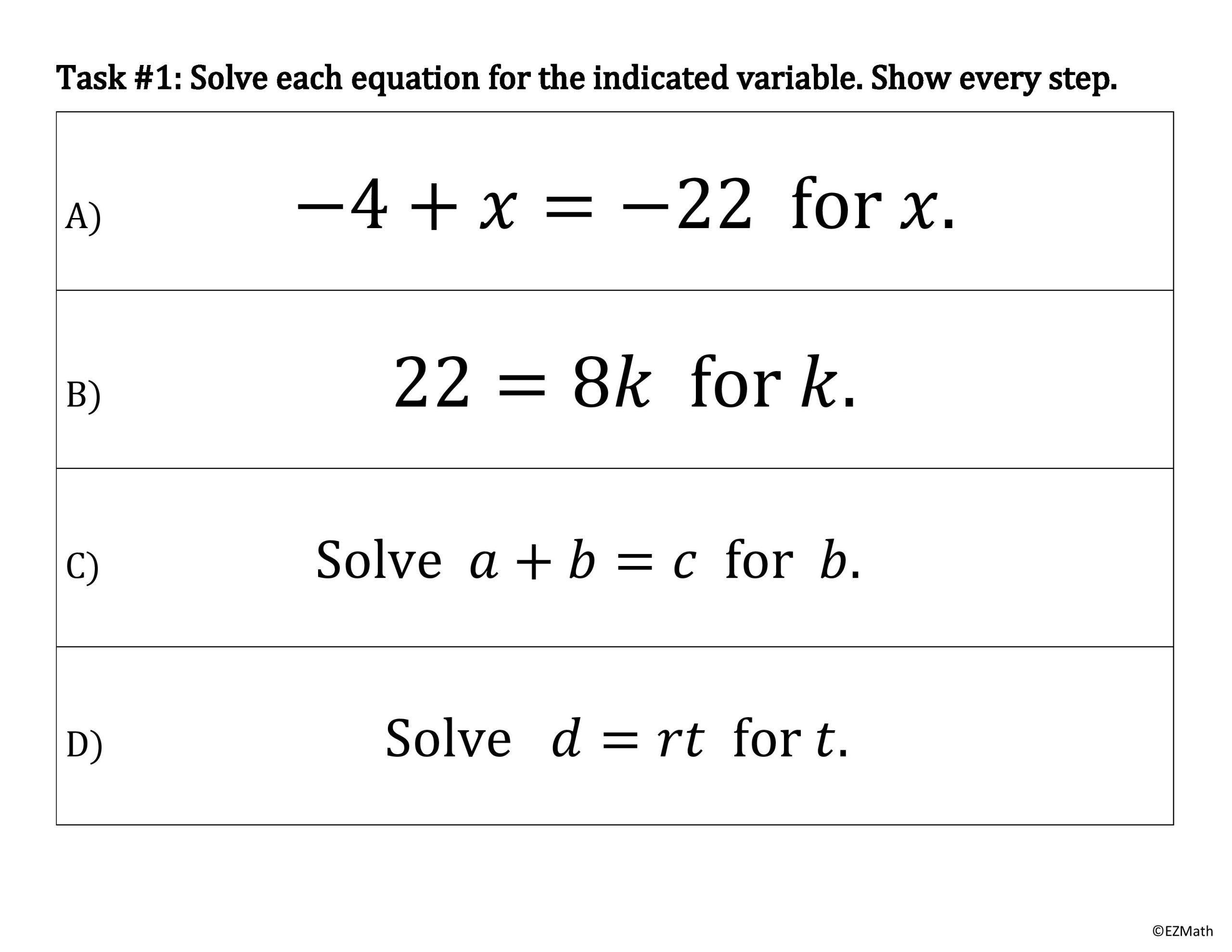
Understanding Literal Equations

Literal equations are mathematical equations that contain variables, constants, and algebraic expressions, where the goal is to solve for a specific variable or expression. These equations are commonly used in various fields, such as physics, engineering, and economics, to model real-world problems. In this article, we will explore the concept of literal equations, provide step-by-step solutions, and offer valuable tips to make solving them easier.

Types of Literal Equations

Literal equations can be classified into two main categories:

• Simple Literal Equations: These equations involve a single variable or expression and can be solved using basic algebraic operations.
• Complex Literal Equations: These equations involve multiple variables or expressions and require more advanced algebraic techniques to solve.

Step-by-Step Solution to Simple Literal Equations

To solve simple literal equations, follow these steps:

1. Read the equation carefully: Identify the variable or expression you need to solve for.
2. Isolate the variable: Use algebraic operations to isolate the variable or expression on one side of the equation.
3. Simplify the equation: Combine like terms and simplify the equation as much as possible.
4. Check your solution: Plug your solution back into the original equation to ensure it is true.

Example: Solving a Simple Literal Equation

Solve for x: 2x + 5 = 11

1. Read the equation carefully: We need to solve for x.
2. Isolate the variable: Subtract 5 from both sides of the equation: 2x = 11 - 5, 2x = 6.
3. Simplify the equation: Divide both sides of the equation by 2: x = ⁶⁄₂, x = 3.
4. Check your solution: Plug x = 3 back into the original equation: 2(3) + 5 = 11, 6 + 5 = 11, 11 = 11 (True).

Step-by-Step Solution to Complex Literal Equations

To solve complex literal equations, follow these steps:

1. Read the equation carefully: Identify the variables or expressions you need to solve for.
2. Use algebraic techniques: Use techniques such as substitution, elimination, or factoring to simplify the equation.
3. Isolate the variable: Use algebraic operations to isolate the variable or expression on one side of the equation.
4. Simplify the equation: Combine like terms and simplify the equation as much as possible.
5. Check your solution: Plug your solution back into the original equation to ensure it is true.

Example: Solving a Complex Literal Equation

Solve for x and y: x + 2y = 7, 3x - 2y = 5

1. Read the equation carefully: We need to solve for x and y.
2. Use algebraic techniques: We can use the elimination method to eliminate the y-variable. Multiply the first equation by 2 and the second equation by 1: 2x + 4y = 14, 3x - 2y = 5 Now, add both equations to eliminate the y-variable: 5x + 2y = 19
3. Isolate the variable: Use algebraic operations to isolate the x-variable: 5x = 19 - 2y, x = (19 - 2y)/5.
4. Simplify the equation: Combine like terms and simplify the equation as much as possible.
5. Check your solution: Plug your solution back into the original equation to ensure it is true.

📝 Note: In complex literal equations, there may be multiple solutions. Always check your solutions to ensure they are true.

Common Mistakes to Avoid

When solving literal equations, avoid these common mistakes:

• Incorrect algebraic operations: Make sure to follow the order of operations (PEMDAS) and use the correct algebraic techniques.
• Incorrect simplification: Combine like terms and simplify the equation as much as possible to avoid errors.
• Not checking solutions: Always check your solutions to ensure they are true.

Conclusion

Solving literal equations requires a deep understanding of algebraic techniques and attention to detail. By following the step-by-step solutions outlined in this article, you can improve your skills in solving both simple and complex literal equations. Remember to always check your solutions to ensure they are true.

Related Terms:

• Literal Equations worksheet answers
• Solving Literal Equations worksheet pdf
• Literal equations worksheet 8th grade
• Hard literal equations worksheet