Solving Linear Equations with Fractions Made Easy

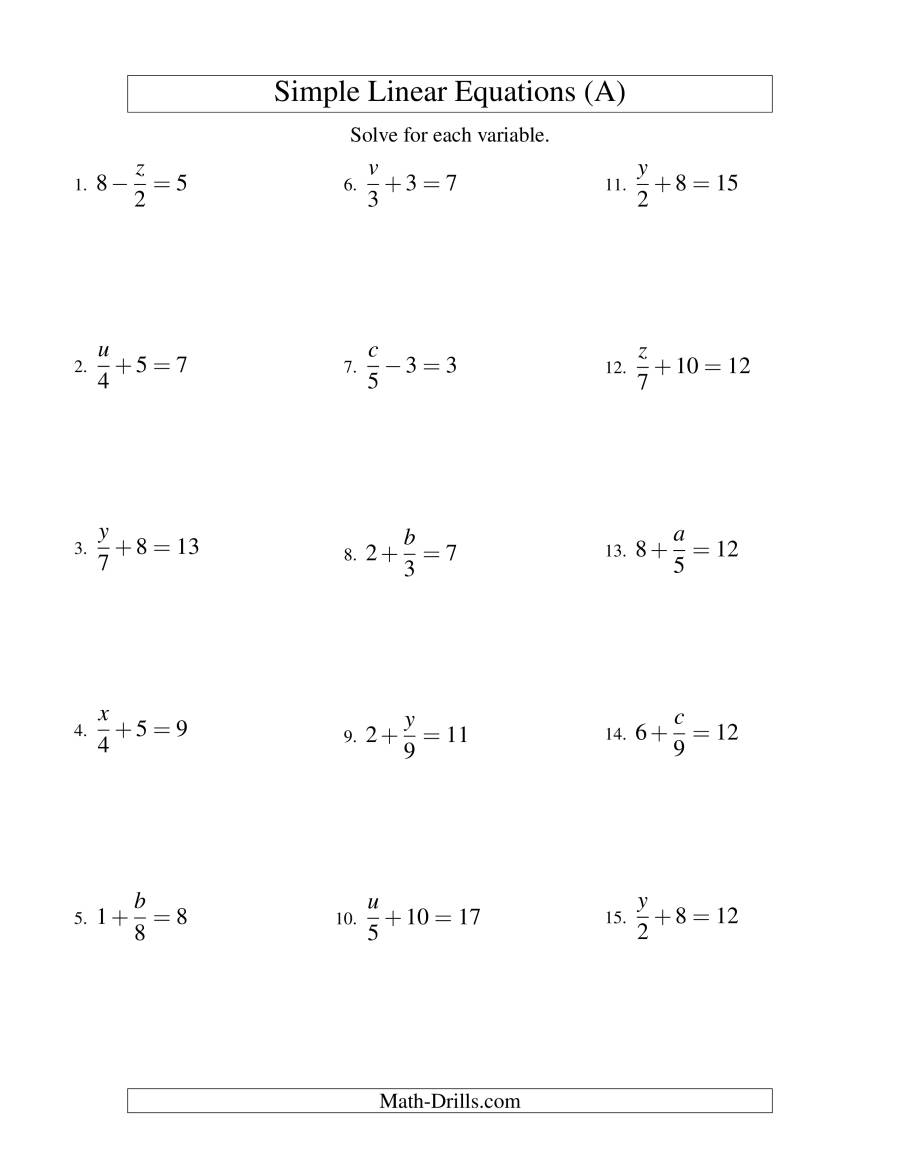
Unlocking the Secrets of Linear Equations with Fractions

Linear equations with fractions can be a daunting task for many students. However, with the right approach and techniques, solving these equations can become a breeze. In this article, we will explore the step-by-step process of solving linear equations with fractions, making it easy and accessible for everyone.

Understanding the Basics

Before diving into the world of linear equations with fractions, it’s essential to understand the basics. A linear equation is an equation in which the highest power of the variable(s) is 1. For example, 2x + 3 = 5 is a linear equation. Fractions, on the other hand, are numbers that represent a part of a whole. When dealing with fractions in linear equations, we need to ensure that we follow the order of operations (PEMDAS) and simplify the fractions correctly.

Step-by-Step Process

To solve linear equations with fractions, follow these steps:

1. Read the equation carefully: Take a moment to read the equation and understand what’s being asked. Identify the variable(s) and the fractions involved.
2. Find a common denominator: If there are multiple fractions in the equation, find a common denominator to simplify the equation. This will make it easier to work with the fractions.
3. Multiply both sides by the common denominator: Once you have the common denominator, multiply both sides of the equation by it. This will eliminate the fractions.
4. Simplify the equation: After multiplying both sides by the common denominator, simplify the equation by combining like terms.
5. Isolate the variable: Use algebraic manipulation to isolate the variable(s) on one side of the equation.
6. Check your solution: Once you’ve solved the equation, plug your solution back into the original equation to ensure it’s true.

🤔 Note: When multiplying both sides of the equation by the common denominator, make sure to distribute the multiplication correctly to avoid errors.

Example Problem 1

Solve the equation: 1/2x + ¹⁄₄ = ³⁄₄

Step 1: Read the equation carefully and identify the variable and fractions.

Step 2: Find a common denominator. The least common multiple (LCM) of 2, 4, and 4 is 4. So, the common denominator is 4.

Step 3: Multiply both sides of the equation by the common denominator (4).

4(1/2x + ¹⁄₄) = 4(³⁄₄)

Step 4: Simplify the equation by combining like terms.

2x + 1 = 3

Step 5: Isolate the variable (x) by subtracting 1 from both sides.

2x = 2

Step 6: Divide both sides by 2 to solve for x.

x = 1

Example Problem 2

Solve the equation: 3/4x - ¹⁄₂ = ¹⁄₄

Step 1: Read the equation carefully and identify the variable and fractions.

Step 2: Find a common denominator. The LCM of 4, 2, and 4 is 4. So, the common denominator is 4.

Step 3: Multiply both sides of the equation by the common denominator (4).

4(3/4x - ¹⁄₂) = 4(¹⁄₄)

Step 4: Simplify the equation by combining like terms.

3x - 2 = 1

Step 5: Isolate the variable (x) by adding 2 to both sides.

3x = 3

Step 6: Divide both sides by 3 to solve for x.

x = 1

Tips and Tricks

• When multiplying both sides of the equation by the common denominator, make sure to distribute the multiplication correctly.
• Use a calculator to check your solutions, especially when dealing with complex fractions.
• Practice, practice, practice! The more you practice solving linear equations with fractions, the more comfortable you’ll become.

Conclusion

Solving linear equations with fractions doesn’t have to be intimidating. By following the step-by-step process outlined in this article, you’ll be able to tackle even the most complex equations with confidence. Remember to take your time, find a common denominator, and simplify the equation correctly. With practice and patience, you’ll become a master of solving linear equations with fractions.