7 Ways to Solve Linear Equation Word Problems
Understanding Linear Equation Word Problems
Linear equation word problems are a common type of math problem that can be solved using algebraic methods. These problems typically involve real-world scenarios, such as calculating the cost of goods, determining the time it takes to travel a certain distance, or finding the area of a room. To solve these problems, we need to first understand the situation and then use mathematical equations to find the solution. In this post, we will explore 7 ways to solve linear equation word problems and provide examples and notes to help you master this skill.
Method 1: Read and Understand the Problem
The first step to solving linear equation word problems is to read and understand the problem carefully. This involves identifying the key elements of the problem, such as the unknown variable, the given information, and the overall objective. To do this effectively, follow these steps:
- Read the problem carefully and identify the key words and phrases.
- Identify the unknown variable and the given information.
- Determine the overall objective of the problem.
- Check for any units or dimensions that may be relevant to the problem.
🤔 Note: Take your time when reading the problem, and make sure you understand what is being asked before proceeding.
Method 2: Translate the Problem into an Equation
Once you have read and understood the problem, the next step is to translate it into an equation. This involves using the information given in the problem to write a mathematical equation that represents the situation. To do this effectively, follow these steps:
- Identify the key elements of the problem, such as the unknown variable, the given information, and the overall objective.
- Use mathematical operations, such as addition, subtraction, multiplication, and division, to represent the relationships between the variables.
- Write the equation in a clear and concise manner, using variables and constants as needed.
Example:
A bakery sells a total of 250 loaves of bread per day. They sell a combination of whole wheat and white bread. If they sell 30 more whole wheat loaves than white bread loaves, and they sell 120 whole wheat loaves, how many white bread loaves do they sell?
Let x be the number of white bread loaves sold. Then, the number of whole wheat loaves sold is x + 30.
We know that the total number of loaves sold is 250, so we can write the equation:
x + (x + 30) = 250
Combine like terms:
2x + 30 = 250
Subtract 30 from both sides:
2x = 220
Divide both sides by 2:
x = 110
Therefore, the bakery sells 110 white bread loaves per day.
Method 3: Solve the Equation
Now that we have written the equation, the next step is to solve it. This involves using mathematical operations to isolate the unknown variable. To do this effectively, follow these steps:
- Add, subtract, multiply, or divide both sides of the equation to isolate the unknown variable.
- Use inverse operations to undo any operations that have been performed on the variable.
- Check your solution by plugging it back into the original equation.
Example:
Solve the equation:
2x + 5 = 11
Subtract 5 from both sides:
2x = 6
Divide both sides by 2:
x = 3
Therefore, the value of x is 3.
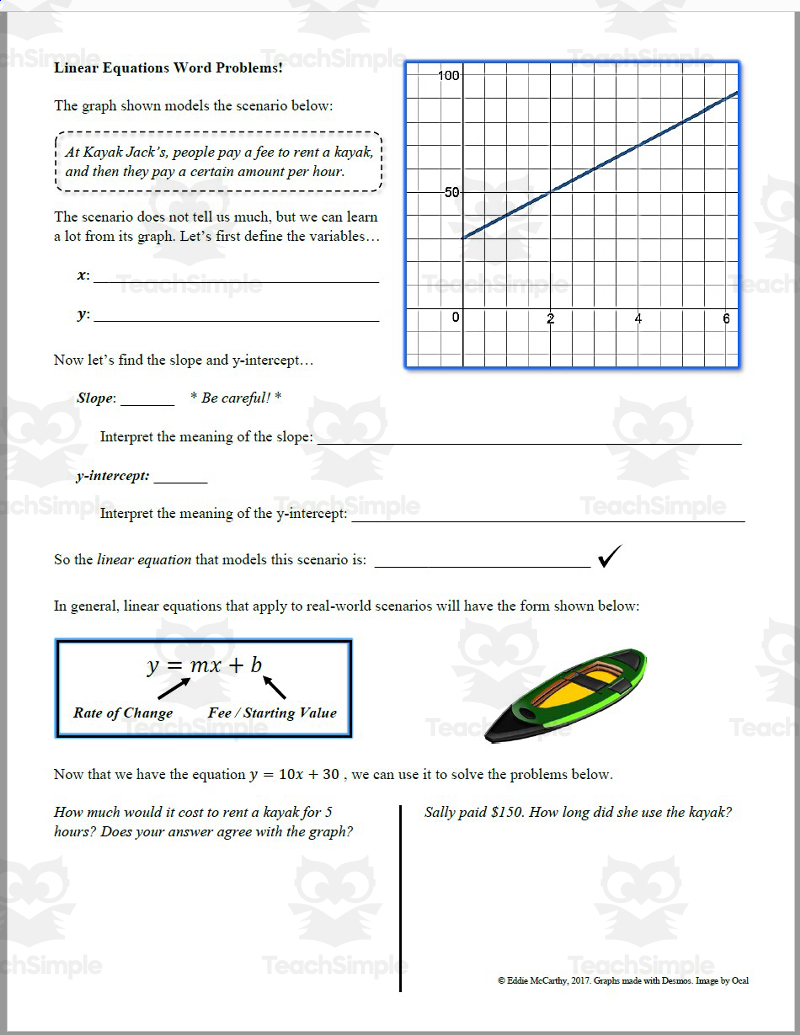
Method 4: Use Graphs to Solve the Equation
Another way to solve linear equation word problems is to use graphs. This involves plotting the equation on a coordinate plane and finding the solution by reading the graph. To do this effectively, follow these steps:
- Plot the equation on a coordinate plane.
- Identify the x-intercept, which represents the solution to the equation.
- Check your solution by plugging it back into the original equation.
Example:
Solve the equation:
x + 2 = 5
Plot the equation on a coordinate plane:
(0, 2), (1, 3), (2, 4), (3, 5), (4, 6)
The x-intercept is (3, 0), which represents the solution to the equation.
Therefore, the value of x is 3.
Method 5: Use Tables to Solve the Equation
Another way to solve linear equation word problems is to use tables. This involves creating a table of values and finding the solution by reading the table. To do this effectively, follow these steps:
- Create a table of values, using the equation to generate the values.
- Identify the value of x that makes the equation true.
- Check your solution by plugging it back into the original equation.
Example:
Solve the equation:
2x + 3 = 7
Create a table of values:

| x | 2x + 3 |
|---|---|
| 0 | 3 |
| 1 | 5 |
| 2 | 7 |
| 3 | 9 |
The value of x that makes the equation true is 2.
Therefore, the value of x is 2.
Method 6: Use Real-World Applications to Solve the Equation
Linear equation word problems often involve real-world applications, such as calculating the cost of goods, determining the time it takes to travel a certain distance, or finding the area of a room. To solve these problems, we can use real-world applications to help us understand the situation and find the solution. To do this effectively, follow these steps:
- Identify the real-world application of the problem.
- Use the application to help you understand the situation and find the solution.
- Check your solution by plugging it back into the original equation.
Example:
A car travels from City A to City B at an average speed of 60 miles per hour. If the distance between the two cities is 180 miles, how long does the trip take?
Let x be the time it takes to make the trip. Then, the distance traveled is 60x.
We know that the distance between the two cities is 180 miles, so we can write the equation:
60x = 180
Divide both sides by 60:
x = 3
Therefore, the trip takes 3 hours.
Method 7: Check Your Solution
Finally, it’s essential to check your solution by plugging it back into the original equation. This ensures that your solution is correct and helps you avoid mistakes. To do this effectively, follow these steps:
- Plug your solution back into the original equation.
- Check that the equation is true.
- Make any necessary adjustments to your solution.
Example:
Solve the equation:
x + 2 = 5
Solution:
x = 3
Check your solution:
3 + 2 = 5
True!
Therefore, the value of x is indeed 3.
Now that we have explored 7 ways to solve linear equation word problems, let’s summarize the key points:
- Read and understand the problem carefully.
- Translate the problem into an equation.
- Solve the equation using mathematical operations.
- Use graphs, tables, and real-world applications to help you understand the situation and find the solution.
- Check your solution by plugging it back into the original equation.
By following these steps and using the methods outlined in this post, you’ll be well on your way to becoming a master of linear equation word problems!
What is the difference between a linear equation and a nonlinear equation?
+A linear equation is an equation in which the highest power of the variable is 1, whereas a nonlinear equation is an equation in which the highest power of the variable is greater than 1.
How do I know which method to use to solve a linear equation word problem?
+The method you choose will depend on the specific problem and your personal preference. However, it’s essential to read and understand the problem carefully and translate it into an equation before choosing a method.
Can I use more than one method to solve a linear equation word problem?
+Yes, you can use more than one method to solve a linear equation word problem. In fact, using multiple methods can help you check your solution and ensure that it’s correct.