Solving Triangles with Ease: Law of Sines Worksheet
Understanding the Law of Sines
The Law of Sines is a fundamental concept in trigonometry that helps us solve triangles with ease. It states that for any given triangle with sides of length a, b, and c, and opposite angles A, B, and C, respectively, the following equation holds true:
a / sin(A) = b / sin(B) = c / sin©
This equation can be used to solve triangles in various ways, including finding missing sides and angles.
Law of Sines Formula
The Law of Sines formula can be written in several ways, depending on the information given. Here are a few variations:
- a / sin(A) = b / sin(B)
- b / sin(B) = c / sin©
- a / sin(A) = c / sin©
These formulas can be used to solve triangles when we know two sides and a non-included angle (SSA), two angles and a non-included side (AAS), or two angles and the included side (ASA).
How to Use the Law of Sines
Using the Law of Sines is relatively straightforward. Here are the steps:
- Identify the information given: sides and angles.
- Choose the correct formula based on the given information.
- Plug in the values and solve for the unknown side or angle.
📝 Note: Make sure to use the correct units for the sides and angles. Angles should be in degrees, and sides should be in the same unit (e.g., meters, inches, etc.).
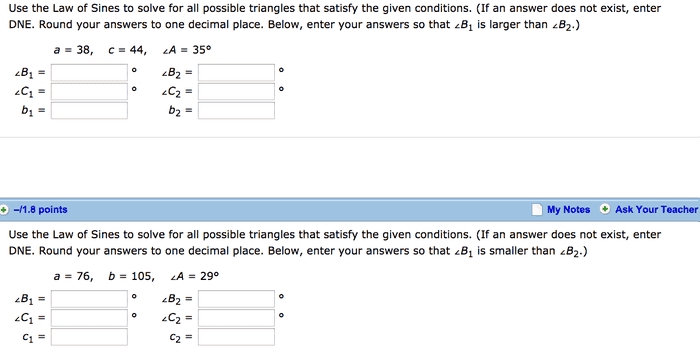
Law of Sines Worksheet
Here are some practice problems to help you master the Law of Sines:
Problem 1: Solve the triangle with sides a = 5, b = 7, and angle A = 30°.
Solution: Use the formula a / sin(A) = b / sin(B) to find angle B.
Problem 2: Find the length of side c in the triangle with sides a = 3, b = 4, and angle C = 60°.
Solution: Use the formula b / sin(B) = c / sin© to find side c.
Problem 3: Solve the triangle with angles A = 45°, B = 60°, and side a = 2.
Solution: Use the formula a / sin(A) = b / sin(B) to find side b.

| Problem | Solution |
|---|---|
| Problem 1: Solve the triangle with sides a = 5, b = 7, and angle A = 30°. | Angle B = 45° |
| Problem 2: Find the length of side c in the triangle with sides a = 3, b = 4, and angle C = 60°. | Side c = 5 |
| Problem 3: Solve the triangle with angles A = 45°, B = 60°, and side a = 2. | Side b = 2.83 |
Tips and Tricks
- Make sure to use the correct units for the sides and angles.
- Use the Law of Sines to solve triangles when the given information matches one of the formulas.
- Practice, practice, practice! The more you practice using the Law of Sines, the more comfortable you’ll become with solving triangles.
In conclusion, the Law of Sines is a powerful tool for solving triangles with ease. By mastering the formulas and practicing with worksheets, you’ll become proficient in solving triangles in no time.
What is the Law of Sines?
+The Law of Sines is a fundamental concept in trigonometry that states that for any given triangle with sides of length a, b, and c, and opposite angles A, B, and C, respectively, the following equation holds true: a / sin(A) = b / sin(B) = c / sin©.
How do I use the Law of Sines to solve triangles?
+Using the Law of Sines is relatively straightforward. Identify the information given, choose the correct formula based on the given information, and plug in the values to solve for the unknown side or angle.
What are some common mistakes to avoid when using the Law of Sines?
+Common mistakes to avoid include using incorrect units for the sides and angles, and not using the correct formula based on the given information.
Related Terms:
- Law of Cosines Worksheet pdf
- Law of Cosines Kuta