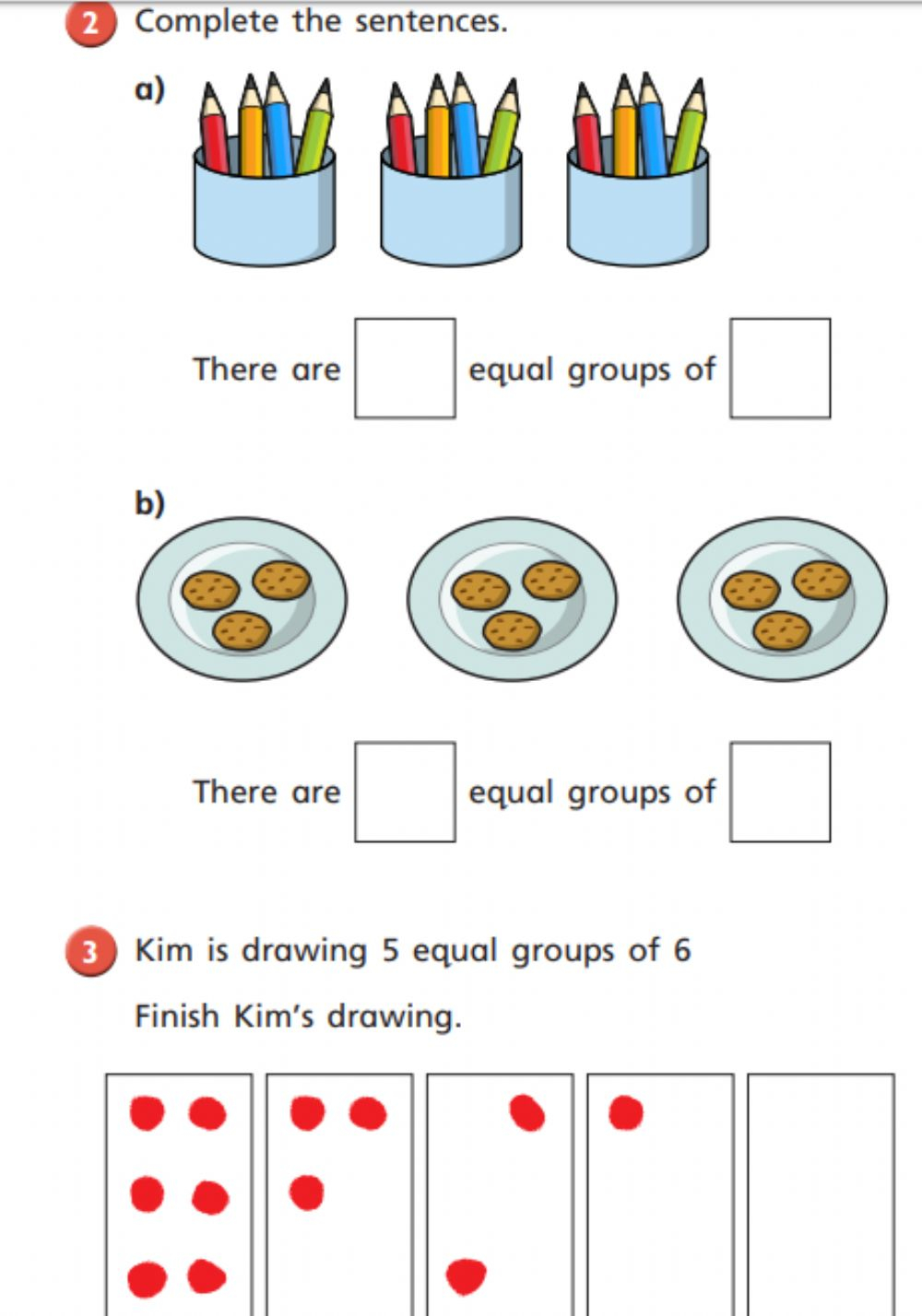
Prime and Composite Numbers Worksheet
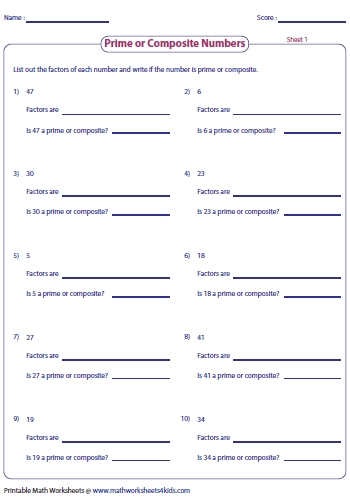
Understanding Prime and Composite Numbers
Prime and composite numbers are fundamental concepts in mathematics, particularly in number theory. These terms are used to describe the properties of positive integers. In this article, we will delve into the definitions, characteristics, and examples of prime and composite numbers, as well as provide a worksheet to help you practice and reinforce your understanding.
What are Prime Numbers?
A prime number is a positive integer greater than 1 that has exactly two distinct factors: 1 and itself. In other words, the only numbers that can divide a prime number are 1 and the number itself. For example, 2, 3, 5, and 7 are prime numbers because they can only be divided evenly by 1 and themselves.
Characteristics of Prime Numbers
Here are some key characteristics of prime numbers:
- They are greater than 1.
- They have exactly two distinct factors: 1 and themselves.
- They cannot be divided evenly by any other number except 1 and themselves.
What are Composite Numbers?
A composite number, on the other hand, is a positive integer greater than 1 that has more than two distinct factors. In other words, a composite number can be divided evenly by numbers other than 1 and itself. For example, 4, 6, 8, and 9 are composite numbers because they can be divided evenly by numbers other than 1 and themselves.
Characteristics of Composite Numbers
Here are some key characteristics of composite numbers:
- They are greater than 1.
- They have more than two distinct factors.
- They can be divided evenly by numbers other than 1 and themselves.
Examples of Prime and Composite Numbers
Here are some examples to illustrate the difference between prime and composite numbers:
| Number | Prime or Composite | Factors |
|---|---|---|
| 2 | Prime | 1, 2 |
| 3 | Prime | 1, 3 |
| 4 | Composite | 1, 2, 4 |
| 5 | Prime | 1, 5 |
| 6 | Composite | 1, 2, 3, 6 |
Worksheet
Now that we have covered the definitions and characteristics of prime and composite numbers, it’s time to practice! Here’s a worksheet to help you reinforce your understanding:
Part 1: Multiple Choice
- Which of the following numbers is a prime number?
- A) 4
- B) 6
- C) 8
- D) 11
- Which of the following numbers is a composite number?
- A) 2
- B) 3
- C) 5
- D) 9
Part 2: Short Answer
- What is the definition of a prime number?
- What is the definition of a composite number?
Part 3: Fill in the Blanks
- The number 7 is a ______________________ number because it can only be divided evenly by 1 and itself.
- The number 12 is a ______________________ number because it can be divided evenly by numbers other than 1 and itself.
Answer Key
Part 1: Multiple Choice
- D) 11
- D) 9
Part 2: Short Answer
- A prime number is a positive integer greater than 1 that has exactly two distinct factors: 1 and itself.
- A composite number is a positive integer greater than 1 that has more than two distinct factors.
Part 3: Fill in the Blanks
- prime
- composite
By completing this worksheet, you should now have a better understanding of prime and composite numbers. Remember, practice makes perfect, so be sure to try more examples on your own to reinforce your knowledge.
In conclusion, prime and composite numbers are fundamental concepts in mathematics, and understanding their definitions and characteristics is crucial for success in number theory and beyond. By practicing with the worksheet provided, you can develop a deeper understanding of these concepts and improve your skills in mathematics.
What is the difference between prime and composite numbers?
+
Prime numbers have exactly two distinct factors: 1 and themselves, while composite numbers have more than two distinct factors.
Can a prime number be divided evenly by any other number?
+
No, a prime number can only be divided evenly by 1 and itself.
What is an example of a composite number?
+
4 is an example of a composite number because it can be divided evenly by numbers other than 1 and itself, such as 2.