Law of Sines and Cosines Worksheet Review Made Easy
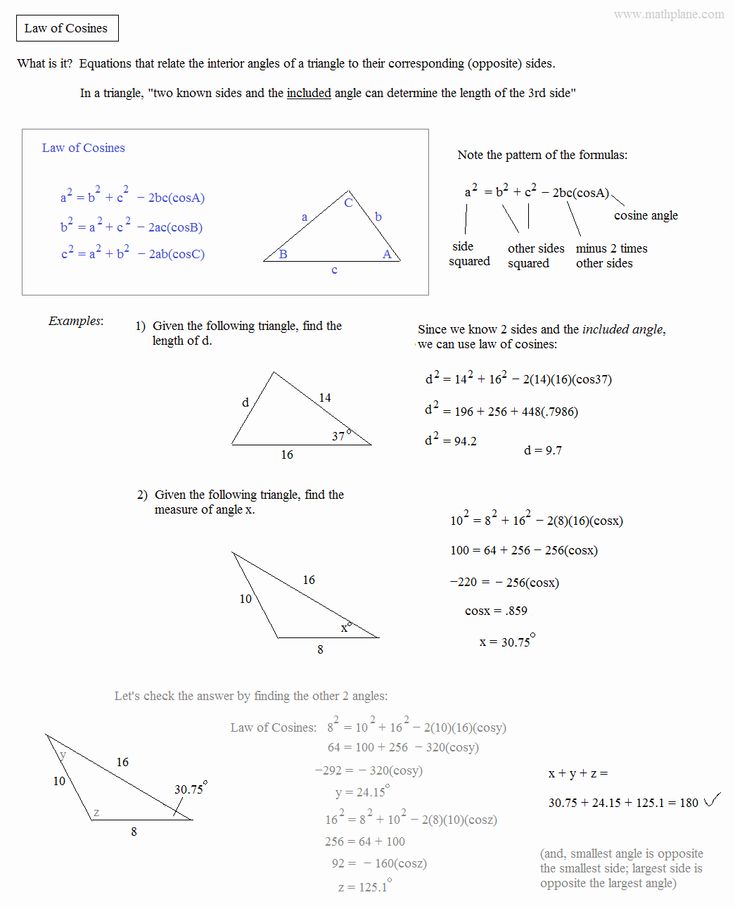
Introduction to the Law of Sines and Cosines
The Law of Sines and Cosines are fundamental concepts in trigonometry that help us solve oblique triangles. An oblique triangle is a triangle that is neither acute nor right-angled. In this post, we will provide a comprehensive review of the Law of Sines and Cosines, including their formulas, applications, and practice problems.
The Law of Sines
The Law of Sines states that for any given triangle with angles A, B, and C, and opposite sides a, b, and c, the following equation holds:
a / sin(A) = b / sin(B) = c / sin©
This formula allows us to find the length of a side of a triangle if we know the length of another side and the measure of two angles.
Example 1: Finding the Length of a Side
In triangle ABC, angle A measures 30°, angle B measures 60°, and side a measures 5 inches. Find the length of side b.
Using the Law of Sines, we can write:
5 / sin(30°) = b / sin(60°)
Solving for b, we get:
b = 5 * sin(60°) / sin(30°) b = 8.66 inches
The Law of Cosines
The Law of Cosines states that for any given triangle with sides a, b, and c, and angle C opposite side c, the following equation holds:
c² = a² + b² - 2ab * cos©
This formula allows us to find the length of a side of a triangle if we know the lengths of the other two sides and the measure of the angle between them.
Example 2: Finding the Length of a Side
In triangle ABC, side a measures 3 inches, side b measures 4 inches, and angle C measures 60°. Find the length of side c.
Using the Law of Cosines, we can write:
c² = 3² + 4² - 2 * 3 * 4 * cos(60°) c² = 9 + 16 - 24 * 0.5 c² = 25 - 12 c² = 13 c = √13 c = 3.61 inches
Applications of the Law of Sines and Cosines
The Law of Sines and Cosines have numerous applications in various fields, including:
- Aviation: Pilots use the Law of Sines and Cosines to calculate distances and angles between landmarks and their aircraft.
- Surveying: Surveyors use the Law of Sines and Cosines to calculate distances and angles between reference points.
- Engineering: Engineers use the Law of Sines and Cosines to design and optimize systems, such as bridges and buildings.
- Computer Science: Computer scientists use the Law of Sines and Cosines to develop algorithms for computer graphics and game development.
Practice Problems
Here are some practice problems to help you review the Law of Sines and Cosines:
- In triangle ABC, angle A measures 45°, angle B measures 60°, and side a measures 6 inches. Find the length of side b.
- In triangle DEF, side d measures 2 inches, side e measures 3 inches, and angle F measures 30°. Find the length of side f.
- In triangle GHI, angle G measures 75°, angle H measures 60°, and side g measures 4 inches. Find the length of side h.
📝 Note: Make sure to show your work and use the formulas to solve the problems.
Conclusion
In conclusion, the Law of Sines and Cosines are powerful tools for solving oblique triangles. By understanding the formulas and applications of these laws, you can become proficient in solving triangle problems. Remember to practice regularly and use online resources to help you review and reinforce your understanding of the Law of Sines and Cosines.
What is the main difference between the Law of Sines and the Law of Cosines?
+The main difference between the Law of Sines and the Law of Cosines is the type of triangle they can solve. The Law of Sines can solve any triangle, while the Law of Cosines is used to solve triangles with two known sides and the angle between them.
Can the Law of Sines be used to solve right triangles?
+No, the Law of Sines is not necessary to solve right triangles. Right triangles can be solved using the Pythagorean theorem or trigonometric ratios.
What are some real-world applications of the Law of Sines and Cosines?
+The Law of Sines and Cosines have numerous applications in various fields, including aviation, surveying, engineering, and computer science.