Grouping Factoring Worksheet: Simplify Equations with Ease

Unlocking the Secrets of Grouping Factoring
Grouping factoring is a powerful technique used to simplify complex equations by factoring out common terms. This method is particularly useful when dealing with quadratic equations, but it can also be applied to other types of equations. In this article, we’ll delve into the world of grouping factoring, explore its benefits, and provide a step-by-step guide on how to simplify equations using this technique.
What is Grouping Factoring?
Grouping factoring is a method of factoring that involves grouping terms together to create a simpler equation. This technique is based on the idea that certain terms can be grouped together to form a new term, which can then be factored out of the equation.
Benefits of Grouping Factoring
Grouping factoring offers several benefits, including:
- Simplifies complex equations: Grouping factoring can help simplify complex equations by breaking them down into more manageable parts.
- Reduces errors: By grouping terms together, you can reduce the likelihood of errors and make it easier to identify mistakes.
- Increases efficiency: Grouping factoring can save time and effort by allowing you to factor out common terms quickly and easily.
Step-by-Step Guide to Grouping Factoring
Here’s a step-by-step guide to grouping factoring:
- Identify the common term: Look for the greatest common factor (GCF) of the terms in the equation.
- Group the terms: Group the terms that have the common factor together.
- Factor out the common term: Factor out the common term from the grouped terms.
- Simplify the equation: Simplify the equation by combining like terms.
📝 Note: Make sure to check if the equation can be factored further after grouping the terms.
Example 1: Simplifying a Quadratic Equation
Let’s consider the following quadratic equation:
x^2 + 5x + 6 = 0
Using grouping factoring, we can simplify the equation as follows:
- Identify the common term: The GCF of the terms is 1.
- Group the terms: Group the terms x^2 and 5x together.
- Factor out the common term: Factor out the common term x from the grouped terms.
- Simplify the equation: Simplify the equation by combining like terms.
x(x + 5) + 6 = 0
x(x + 5) = -6
Example 2: Simplifying a Linear Equation
Let’s consider the following linear equation:
2x + 6 = 10
Using grouping factoring, we can simplify the equation as follows:
- Identify the common term: The GCF of the terms is 2.
- Group the terms: Group the terms 2x and 6 together.
- Factor out the common term: Factor out the common term 2 from the grouped terms.
- Simplify the equation: Simplify the equation by combining like terms.
2(x + 3) = 10
x + 3 = 5
Grouping Factoring Worksheet
Practice makes perfect! Here’s a worksheet to help you practice grouping factoring:
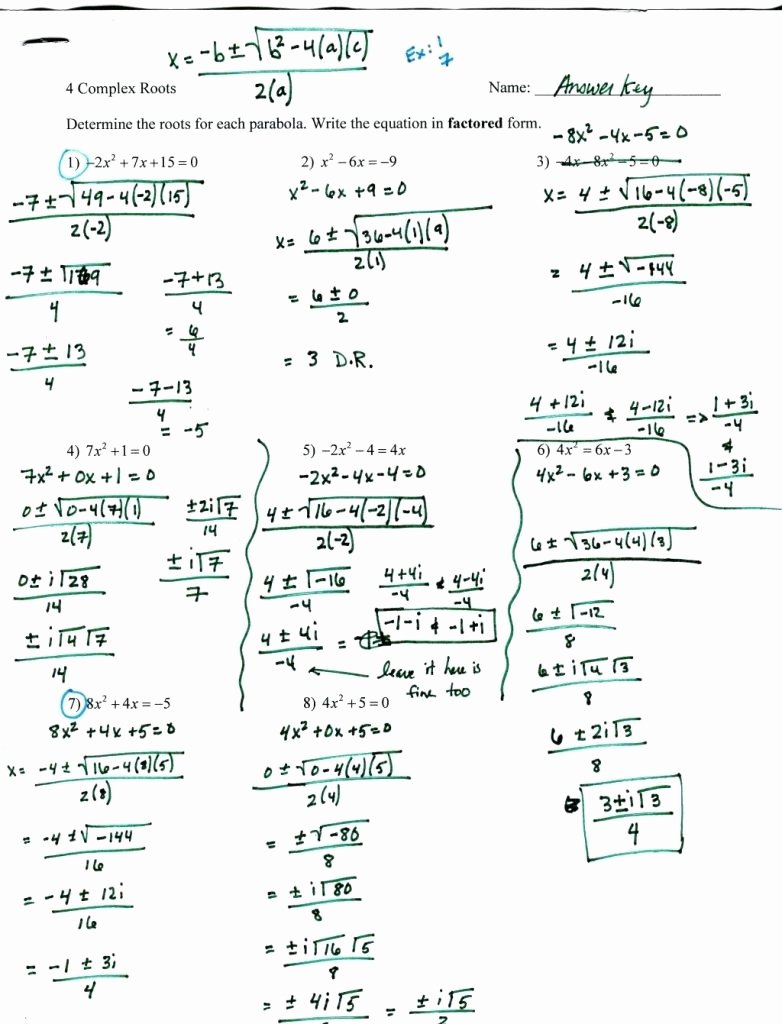
| Equation | Solution |
|---|---|
| x^2 + 3x + 2 = 0 | |
| 2x + 8 = 10 | |
| x^2 + 2x + 1 = 0 | |
| 3x + 9 = 12 | |
| x^2 + 4x + 4 = 0 |
📝 Note: Try to solve the equations on your own before checking the solutions.
Common Mistakes to Avoid
When using grouping factoring, make sure to avoid the following common mistakes:
- Forgetting to check for further factoring: Always check if the equation can be factored further after grouping the terms.
- Incorrectly identifying the common term: Make sure to identify the correct common term before grouping the terms.
- Failing to simplify the equation: Don’t forget to simplify the equation by combining like terms after factoring out the common term.
What is the main benefit of grouping factoring?
+The main benefit of grouping factoring is that it simplifies complex equations by breaking them down into more manageable parts.
How do I identify the common term in an equation?
+To identify the common term, look for the greatest common factor (GCF) of the terms in the equation.
Can grouping factoring be used for all types of equations?
+Grouping factoring can be used for quadratic equations and some linear equations, but it may not be applicable to all types of equations.
In conclusion, grouping factoring is a powerful technique that can simplify complex equations by factoring out common terms. By following the step-by-step guide and practicing with the worksheet, you can master this technique and become more efficient in solving equations.