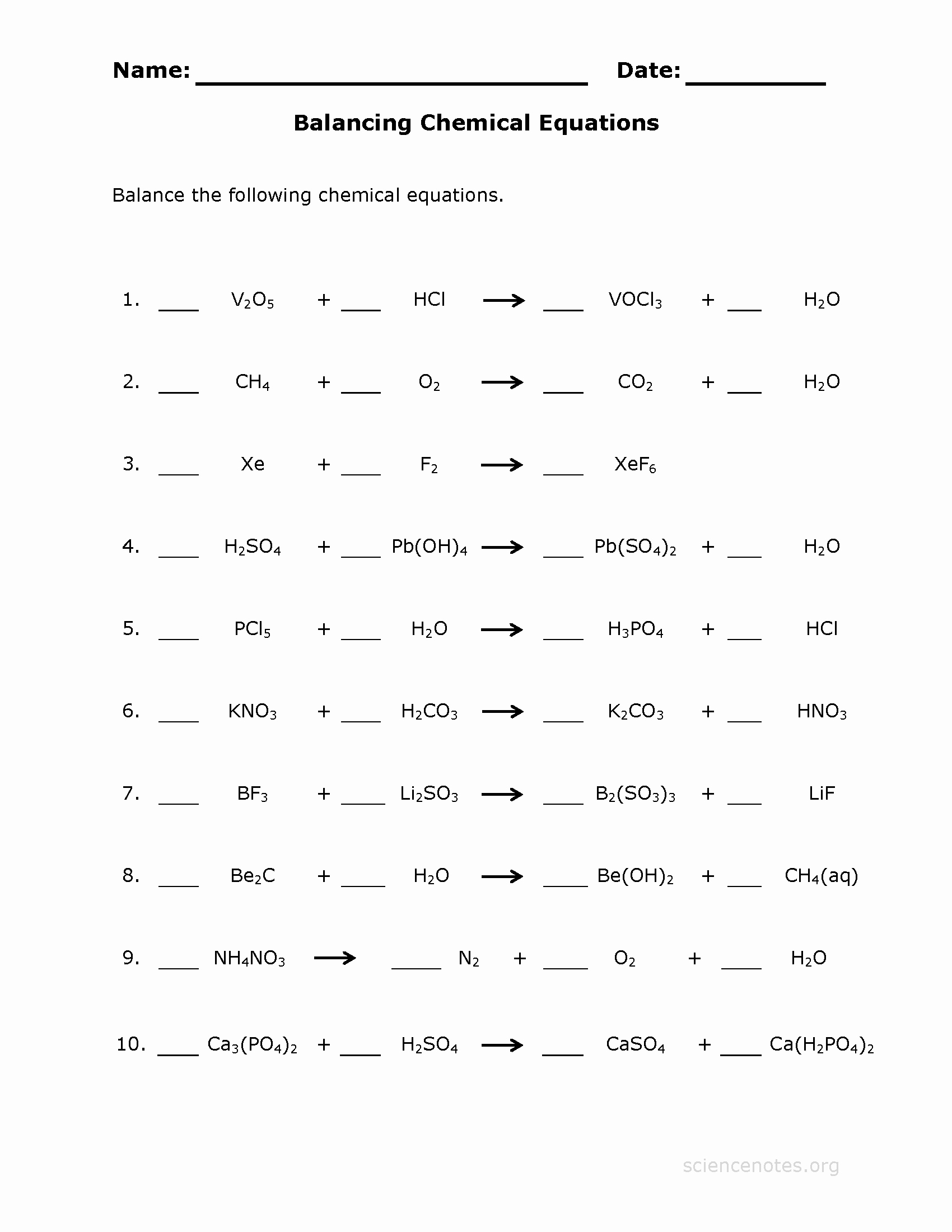
6th Grade GCF Worksheets with 20 Practice Exercises
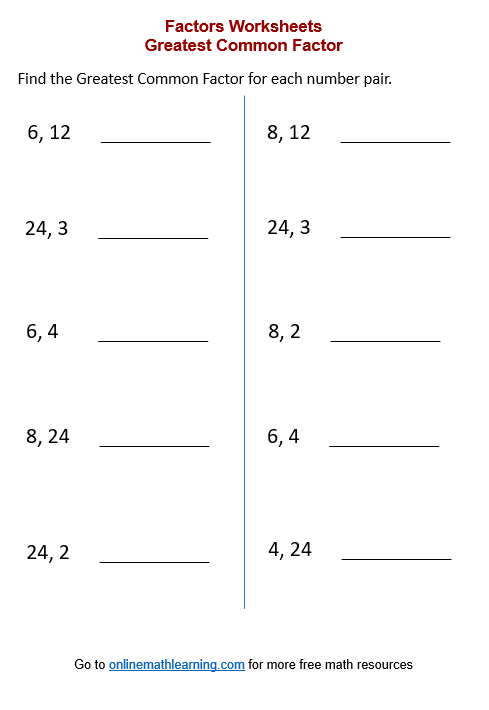
Understanding the Greatest Common Factor (GCF) Concept
The Greatest Common Factor (GCF) is a fundamental concept in mathematics that helps students understand the relationship between numbers. It is the largest positive integer that divides each of the numbers in a set of numbers without leaving a remainder. In this article, we will delve into the world of 6th grade GCF worksheets, providing 20 practice exercises to help students grasp this concept.
What is the Greatest Common Factor (GCF)?
The GCF is the largest number that divides two or more numbers without leaving a remainder. For example, the GCF of 12 and 18 is 6, because 6 is the largest number that divides both 12 and 18 without leaving a remainder.
How to Find the GCF
There are several methods to find the GCF, including:
- Listing Factors: List all the factors of each number and find the greatest common factor.
- Prime Factorization: Break down each number into its prime factors and find the common factors.
- Using a Venn Diagram: Use a Venn diagram to find the overlapping factors of two numbers.
6th Grade GCF Worksheets with 20 Practice Exercises
Here are 20 practice exercises to help 6th grade students master the concept of GCF:
Exercises 1-5: Listing Factors
- Find the GCF of 12 and 15.
- Find the GCF of 24 and 30.
- Find the GCF of 18 and 24.
- Find the GCF of 9 and 12.
- Find the GCF of 20 and 25.
Exercises 6-10: Prime Factorization
- Find the GCF of 48 and 60 using prime factorization.
- Find the GCF of 36 and 48 using prime factorization.
- Find the GCF of 24 and 36 using prime factorization.
- Find the GCF of 18 and 24 using prime factorization.
- Find the GCF of 12 and 18 using prime factorization.
Exercises 11-15: Using a Venn Diagram
- Find the GCF of 20 and 30 using a Venn diagram.
- Find the GCF of 24 and 36 using a Venn diagram.
- Find the GCF of 18 and 24 using a Venn diagram.
- Find the GCF of 12 and 15 using a Venn diagram.
- Find the GCF of 9 and 12 using a Venn diagram.
Exercises 16-20: Word Problems
- Tom has 18 pencils and 24 pens. What is the greatest number of sets of 6 pencils and 6 pens that Tom can make?
- A bookshelf has 12 shelves, and each shelf can hold 15 books. What is the greatest number of sets of 3 shelves with 3 books on each shelf?
- A bakery has 24 cupcakes and 30 cookies. What is the greatest number of sets of 6 cupcakes and 6 cookies that the bakery can make?
- A group of friends want to share some candy equally. If they have 20 pieces of candy and want to divide it among 4 friends, what is the greatest number of pieces of candy each friend can get?
- A store has 18 shirts and 24 pants. What is the greatest number of sets of 6 shirts and 6 pants that the store can make?
📝 Note: Encourage students to use different methods to find the GCF, such as listing factors, prime factorization, and using a Venn diagram.
These 20 practice exercises will help 6th grade students develop a deep understanding of the GCF concept and its applications in real-world problems.
What is the purpose of finding the GCF?
+The GCF is used to simplify fractions, find the least common multiple (LCM), and solve problems involving equivalent ratios.
How can I find the GCF of three or more numbers?
+To find the GCF of three or more numbers, find the GCF of two numbers first, and then find the GCF of the result and the third number.
Can I use a calculator to find the GCF?
+While calculators can be used to find the GCF, it is recommended to practice finding the GCF manually to develop a deeper understanding of the concept.
Related Terms:
- GCF worksheets Grade 6 pdf
- Greatest common factor grade 6
- Greatest common factor worksheets PDF
- LCM 6th grade worksheets
- LCM worksheets Grade 6 pdf