5 Ways to Master Greatest Common Factor Worksheets

Unlocking the Secrets of Greatest Common Factor Worksheets
Mastering greatest common factor (GCF) worksheets can be a challenging task for students, but with the right approach, it can become a breeze. In this article, we will explore five ways to help you master GCF worksheets and become a math whiz.
Understanding the Concept of GCF
Before we dive into the strategies, it’s essential to understand what GCF is all about. The greatest common factor is the largest positive integer that divides two or more numbers without leaving a remainder. For example, the GCF of 12 and 18 is 6, because 6 is the largest number that divides both 12 and 18 without leaving a remainder.
Strategy 1: Using the Listing Method
The listing method is a simple and effective way to find the GCF of two numbers. Here’s how it works:
- List all the factors of each number.
- Identify the common factors.
- Choose the largest common factor.
For example, let’s find the GCF of 12 and 18 using the listing method:
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 18: 1, 2, 3, 6, 9, 18
- Common factors: 1, 2, 3, 6
- GCF: 6
📝 Note: The listing method can be time-consuming for larger numbers. However, it's a great way to develop your understanding of GCF.
Strategy 2: Using the Prime Factorization Method
The prime factorization method is another way to find the GCF of two numbers. Here’s how it works:
- Find the prime factors of each number.
- Identify the common prime factors.
- Multiply the common prime factors.
For example, let’s find the GCF of 12 and 18 using the prime factorization method:
- Prime factors of 12: 2 × 2 × 3
- Prime factors of 18: 2 × 3 × 3
- Common prime factors: 2 × 3
- GCF: 6
Strategy 3: Using the Euclidean Algorithm
The Euclidean algorithm is a more efficient way to find the GCF of two numbers. Here’s how it works:
- Divide the larger number by the smaller number.
- Take the remainder and divide it into the smaller number.
- Repeat the process until the remainder is 0.
- The last non-zero remainder is the GCF.
For example, let’s find the GCF of 12 and 18 using the Euclidean algorithm:
- 18 ÷ 12 = 1 remainder 6
- 12 ÷ 6 = 2 remainder 0
- GCF: 6
Strategy 4: Using the Ladder Method
The ladder method is a visual way to find the GCF of two numbers. Here’s how it works:
- Draw a ladder with the numbers on either side.
- Find the common factors and mark them on the ladder.
- The highest common factor is the GCF.
For example, let’s find the GCF of 12 and 18 using the ladder method:
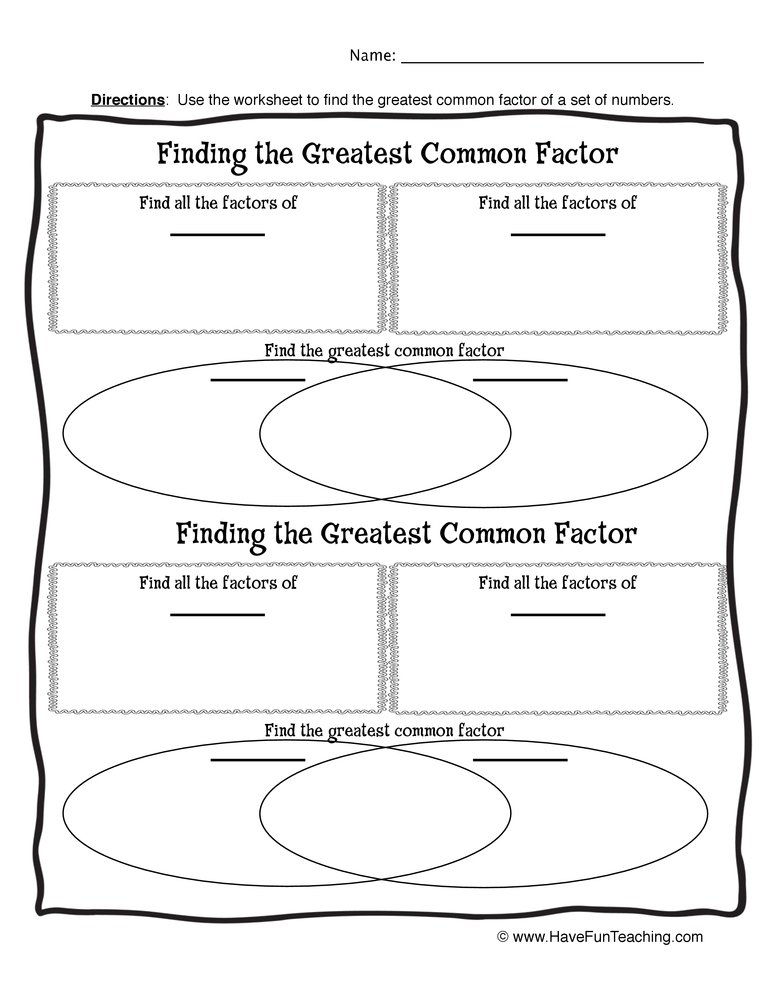
| 12 | 18 |
|---|---|
| 1 | 1 |
| 2 | 2 |
| 3 | 3 |
| 4 | 6 |
| 6 | 9 |
| 12 | 18 |
GCF: 6
Strategy 5: Practicing with Worksheets
Practice makes perfect! The best way to master GCF worksheets is to practice with different types of problems. Here are some tips to help you practice effectively:
- Start with simple problems and gradually move on to more challenging ones.
- Use a variety of strategies to find the GCF.
- Check your answers to ensure accuracy.
- Practice regularly to build your confidence and speed.
By following these five strategies, you’ll become a master of GCF worksheets in no time. Remember to practice regularly and use a variety of strategies to find the GCF. With time and practice, you’ll be able to solve GCF problems with ease and confidence.
The key to mastering GCF worksheets is to understand the concept of GCF and to practice regularly using different strategies. By following the strategies outlined in this article, you’ll be well on your way to becoming a math whiz.
What is the greatest common factor (GCF)?
+The greatest common factor is the largest positive integer that divides two or more numbers without leaving a remainder.
What are the different strategies to find the GCF?
+There are several strategies to find the GCF, including the listing method, prime factorization method, Euclidean algorithm, ladder method, and practicing with worksheets.
Why is it important to practice with GCF worksheets?
+Practicing with GCF worksheets helps to build confidence and speed in finding the GCF, and it also helps to develop problem-solving skills.