5 Ways to Master Greater Than Less Than Equal to
Understanding the Basics of Greater Than, Less Than, and Equal To
Greater than, less than, and equal to are fundamental concepts in mathematics that are used to compare numbers. These symbols are essential in various mathematical operations, including algebra, geometry, and calculus. Mastering these concepts is crucial for problem-solving and critical thinking. In this article, we will explore five ways to help you master greater than, less than, and equal to.
1. Visualize the Number Line
One effective way to understand greater than, less than, and equal to is to visualize the number line. Imagine a horizontal line with numbers marked on it. The number line can help you compare numbers and determine which one is greater or lesser.
- To the left of a number, the numbers are smaller (less than).
- To the right of a number, the numbers are larger (greater than).
- When two numbers are on the same point on the number line, they are equal.
📝 Note: The number line is an essential tool for visualizing and comparing numbers.
2. Practice with Real-World Examples
Practicing with real-world examples can help you apply the concepts of greater than, less than, and equal to in everyday situations. Here are a few examples:
- Compare the prices of two products: If a shirt costs 20 and a pair of pants costs 30, the shirt is less expensive (less than) than the pants.
- Compare the heights of two people: If John is 5’8” and Emily is 5’9”, Emily is taller (greater than) than John.
- Compare the scores of two students: If Sarah scored 85 on a test and Tom scored 85 on the same test, their scores are equal.
3. Use Symbolic Representations
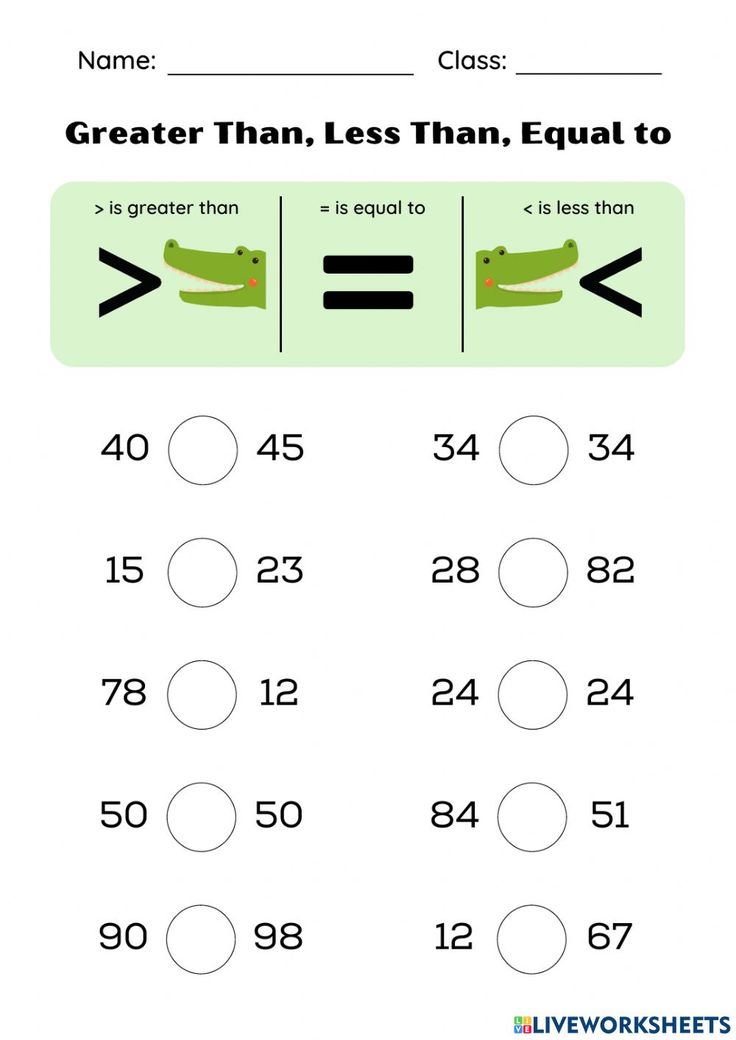
Using symbolic representations can help you write equations and inequalities that involve greater than, less than, and equal to. Here are the symbols and their meanings:
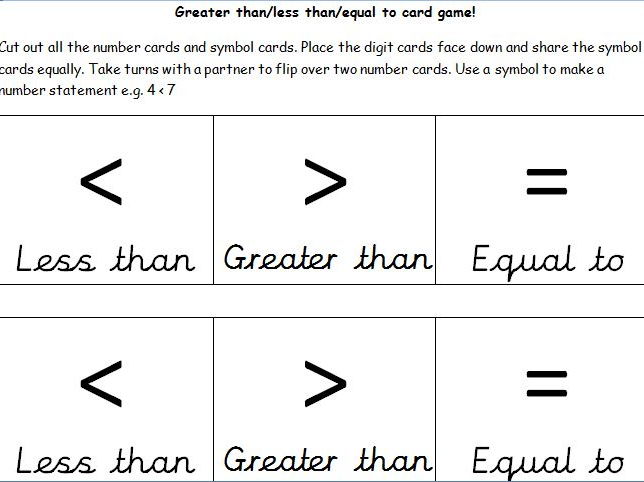
| Symbol | Meaning |
|---|---|
| > | Greater than |
| < | Less than |
| = | Equal to |
| ≥ | Greater than or equal |
| ≤ | Less than or equal |
For example:
- 2 > 1 means 2 is greater than 1.
- 3 < 4 means 3 is less than 4.
- 5 = 5 means 5 is equal to 5.
4. Solve Inequalities
Solving inequalities involves using greater than, less than, and equal to symbols to compare expressions. Here’s an example:
Solve for x: 2x + 3 > 5
To solve this inequality, you need to isolate the variable x.
- Subtract 3 from both sides: 2x > 2
- Divide both sides by 2: x > 1
Therefore, the solution to the inequality is x > 1.
5. Play Online Games and Activities
Playing online games and activities can make learning greater than, less than, and equal to fun and engaging. Here are a few examples:
- “Greater Than, Less Than” game: This game involves comparing numbers and symbols to determine which one is greater or lesser.
- “Math Bingo”: This game involves solving math problems, including inequalities, to mark numbers on a bingo card.
Summarizing key points:
Mastering greater than, less than, and equal to requires practice, visualization, and real-world applications. By using the number line, practicing with real-world examples, using symbolic representations, solving inequalities, and playing online games and activities, you can become proficient in these fundamental math concepts.
What is the difference between greater than and less than?
+
Greater than (>) means a number is larger than another number, while less than (<) means a number is smaller than another number.
How do I solve an inequality?
+
To solve an inequality, you need to isolate the variable by performing algebraic operations, such as addition, subtraction, multiplication, and division.
What are some real-world applications of greater than, less than, and equal to?
+
Greater than, less than, and equal to are used in various real-world applications, such as comparing prices, heights, scores, and temperatures.