Geometry Basics: Segment Addition Postulate Made Easy
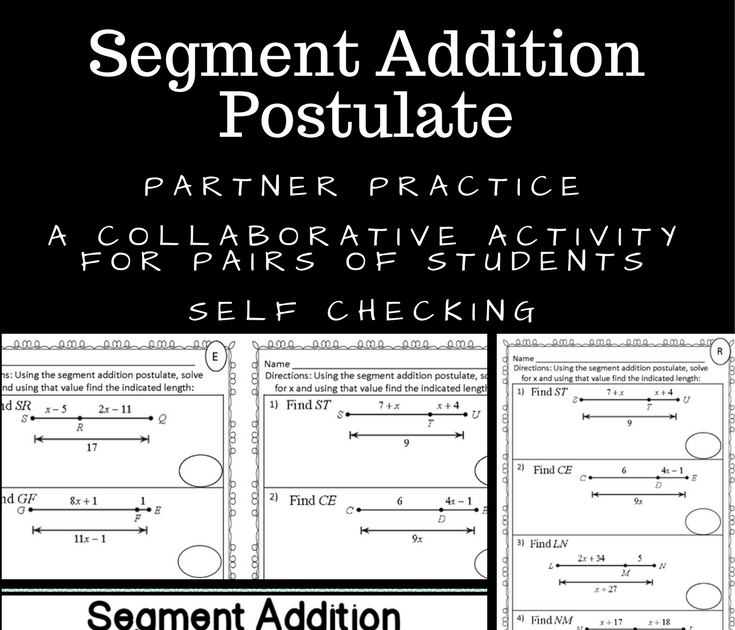
Unlocking the Secrets of Geometry: The Segment Addition Postulate
Geometry, the branch of mathematics that deals with shapes and sizes, can be intimidating for many students. However, understanding the basics of geometry is crucial for problem-solving and critical thinking. One of the fundamental concepts in geometry is the Segment Addition Postulate. In this blog post, we will explore the Segment Addition Postulate in detail, making it easy for you to grasp and apply.
What is the Segment Addition Postulate?
The Segment Addition Postulate states that if two segments share a common endpoint, then the length of the third segment formed by combining the two segments is equal to the sum of the lengths of the individual segments. Mathematically, this can be expressed as:
a + b = c
where a and b are the lengths of the two segments, and c is the length of the third segment formed by combining them.
Understanding the Concept
To illustrate the Segment Addition Postulate, let’s consider an example. Suppose we have two segments, AB and BC, with lengths 3 cm and 4 cm, respectively. If we combine these two segments to form a third segment AC, the length of AC would be the sum of the lengths of AB and BC, which is 7 cm.
| Segment | Length (cm) |
|---|---|
| AB | 3 |
| BC | 4 |
| AC | 7 |
Key Points to Remember
When applying the Segment Addition Postulate, keep the following points in mind:
- The two segments must share a common endpoint.
- The length of the third segment is the sum of the lengths of the individual segments.
- The postulate only applies to collinear segments (segments that lie on the same line).
📝 Note: The Segment Addition Postulate is a fundamental concept in geometry, and understanding it will help you solve problems involving segment lengths and midpoints.
Real-World Applications
The Segment Addition Postulate has numerous applications in real-world scenarios, such as:
- Construction: When building a house, architects and engineers need to calculate the length of walls, roofs, and other structural elements. The Segment Addition Postulate helps them do so accurately.
- Surveying: Surveyors use the postulate to calculate distances between landmarks and boundaries.
- Computer-Aided Design (CAD): CAD software relies on the Segment Addition Postulate to create accurate models and designs.
Common Misconceptions
When working with the Segment Addition Postulate, be aware of the following common misconceptions:
- Assuming non-collinear segments: Make sure the segments share a common endpoint and lie on the same line.
- Forgetting to include units: Always include units (such as cm, m, or km) when working with segment lengths.
Conclusion
In conclusion, the Segment Addition Postulate is a fundamental concept in geometry that helps you understand and work with segment lengths. By grasping this concept, you’ll be able to solve problems and apply your knowledge to real-world scenarios. Remember to keep the key points in mind, and avoid common misconceptions to ensure accuracy in your calculations.
What is the Segment Addition Postulate?
+The Segment Addition Postulate states that if two segments share a common endpoint, then the length of the third segment formed by combining the two segments is equal to the sum of the lengths of the individual segments.
What are some real-world applications of the Segment Addition Postulate?
+The Segment Addition Postulate has applications in construction, surveying, and computer-aided design (CAD).
What is a common misconception when working with the Segment Addition Postulate?
+One common misconception is assuming non-collinear segments, which can lead to incorrect calculations.