5 Ways to Master Fractions of Whole Numbers
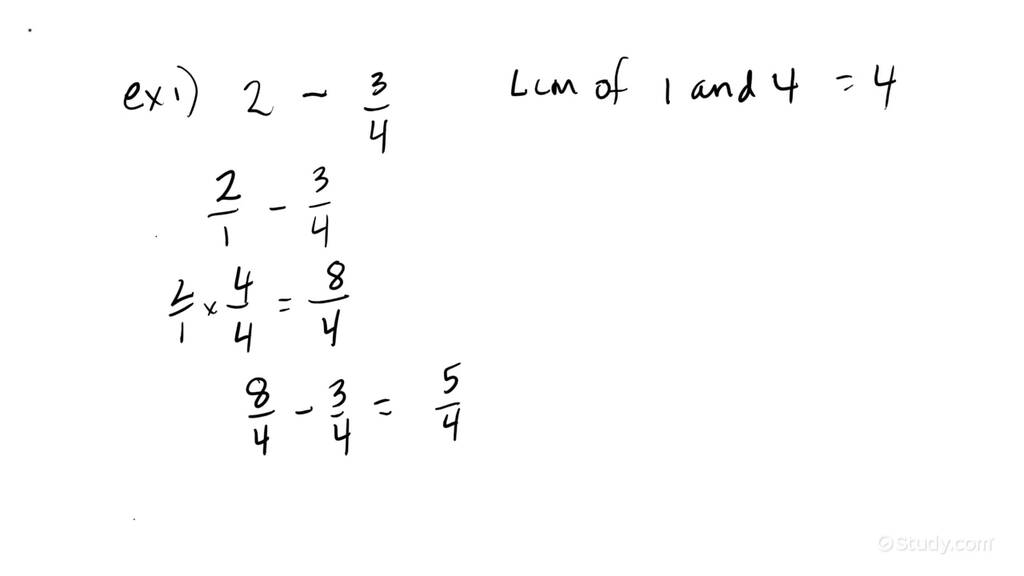
Understanding Fractions of Whole Numbers
Fractions of whole numbers are a fundamental concept in mathematics, and mastering them is essential for problem-solving and critical thinking. A fraction of a whole number represents a part of the whole, and it’s denoted by a numerator (the top number) and a denominator (the bottom number). In this article, we’ll explore five ways to master fractions of whole numbers, making it easier for you to work with them in various mathematical contexts.
1. Visualize Fractions Using Real-World Examples
Visualizing fractions can help you understand the concept better. Imagine you have a pizza that’s divided into 8 slices. If you eat 2 slices, you’ve consumed 2⁄8 of the pizza. This real-world example illustrates the concept of a fraction, where the numerator (2) represents the number of slices eaten, and the denominator (8) represents the total number of slices.
Visualizing Fractions Tips:
- Use everyday objects, such as fruits, vegetables, or toys, to demonstrate fractions.
- Draw diagrams or charts to represent fractions and make them more tangible.
- Practice identifying fractions in real-world scenarios, like measuring ingredients for a recipe.
2. Simplify Fractions to Their Lowest Terms
Simplifying fractions is an essential skill to master. When you simplify a fraction, you reduce it to its lowest terms, making it easier to work with. For example, the fraction 4⁄8 can be simplified to 1⁄2 by dividing both the numerator and denominator by 4.
Simplifying Fractions Tips:
- Look for common factors between the numerator and denominator.
- Divide both numbers by the greatest common factor (GCF).
- Check if the fraction can be simplified further.
3. Add and Subtract Fractions with Like Denominators
Adding and subtracting fractions with like denominators is a fundamental operation in mathematics. When you have fractions with the same denominator, you can simply add or subtract the numerators.
Adding and Subtracting Fractions Tips:
- Make sure the denominators are the same.
- Add or subtract the numerators, keeping the denominator unchanged.
- Simplify the resulting fraction, if possible.

| Fraction 1 | Fraction 2 | Result |
|---|---|---|
| 1⁄8 | 2⁄8 | 3⁄8 |
| 3⁄8 | 1⁄8 | 4⁄8 |
4. Multiply Fractions by Whole Numbers
Multiplying fractions by whole numbers is another crucial operation. When you multiply a fraction by a whole number, you multiply the numerator by that number, keeping the denominator unchanged.
Multiplying Fractions Tips:
- Multiply the numerator by the whole number.
- Keep the denominator unchanged.
- Simplify the resulting fraction, if possible.
Example:
Multiply 3⁄4 by 2:
(3 × 2) / 4 = 6⁄4 = 1 1⁄2
5. Convert Between Fractions and Mixed Numbers
Converting between fractions and mixed numbers is an essential skill. A mixed number consists of a whole number and a fraction, like 2 1⁄2. To convert a mixed number to a fraction, multiply the whole number by the denominator, then add the numerator.
Converting Between Fractions and Mixed Numbers Tips:
- Multiply the whole number by the denominator.
- Add the numerator to the result.
- Simplify the resulting fraction, if possible.
Example:
Convert 2 1⁄2 to a fraction:
(2 × 2) + 1 = 5
Result: 5⁄2
📝 Note: When converting from a fraction to a mixed number, divide the numerator by the denominator, and the remainder will be the new numerator.
In conclusion, mastering fractions of whole numbers requires practice, patience, and a solid understanding of the concepts. By visualizing fractions, simplifying them, adding and subtracting with like denominators, multiplying by whole numbers, and converting between fractions and mixed numbers, you’ll become proficient in working with fractions in no time. Remember to practice regularly and apply these concepts to real-world problems to reinforce your understanding.
What is a fraction of a whole number?
+A fraction of a whole number represents a part of the whole, denoted by a numerator (the top number) and a denominator (the bottom number).
How do I simplify a fraction?
+To simplify a fraction, look for common factors between the numerator and denominator, and divide both numbers by the greatest common factor (GCF). Check if the fraction can be simplified further.
Can I add and subtract fractions with different denominators?
+No, you can only add and subtract fractions with like denominators. If the denominators are different, you need to find the least common multiple (LCM) of the denominators and convert both fractions to have the LCM as the denominator.