5 Ways to Master Fraction Multiplication

Unlocking the Secrets of Fraction Multiplication
Fraction multiplication is a fundamental concept in mathematics that can be a bit tricky to grasp at first, but with practice and the right strategies, anyone can master it. In this article, we will explore five ways to help you become a pro at multiplying fractions.
Understanding the Basics
Before we dive into the advanced techniques, let’s review the basics of fraction multiplication. To multiply two fractions, you simply multiply the numerators (the numbers on top) and multiply the denominators (the numbers on the bottom). The resulting fraction is the product of the two fractions.
🤔 Note: When multiplying fractions, you don't need to find the least common multiple (LCM) of the denominators, unlike when adding or subtracting fractions.
1. The Traditional Method
The traditional method of multiplying fractions involves multiplying the numerators and denominators separately and then simplifying the resulting fraction.
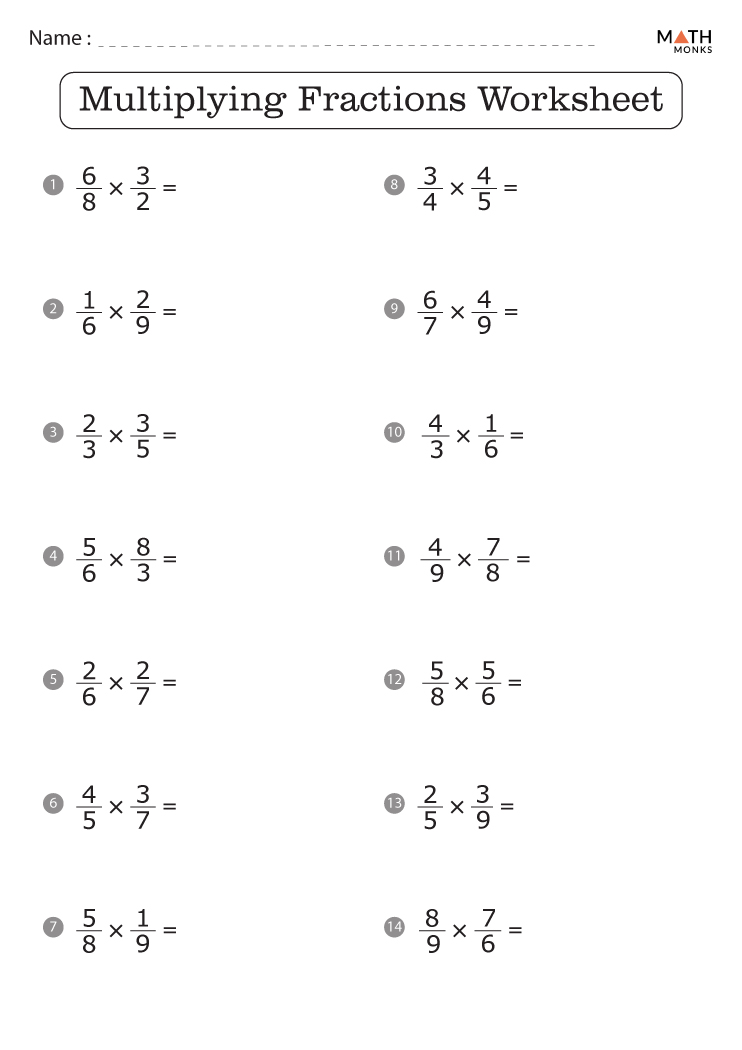
| Fraction 1 | Fraction 2 | Product |
|---|---|---|
| 1/2 | 3/4 | (1 x 3) / (2 x 4) = 3/8 |
| 2/3 | 5/6 | (2 x 5) / (3 x 6) = 10/18 = 5/9 |
2. The Area Model
The area model is a visual representation of fraction multiplication that can help you understand the concept better. Imagine two rectangles, each representing one of the fractions. The area of the overlapping region represents the product of the two fractions.

3. The Number Line Method
The number line method involves representing the fractions on a number line and then multiplying them by counting the units.

4. The Grid Method
The grid method involves creating a grid with the numerators and denominators of the fractions and then multiplying them by counting the units.

5. The Real-World Application Method
The real-world application method involves using everyday objects to demonstrate fraction multiplication. For example, if you have 1⁄2 cup of flour and you want to triple it, you can multiply 1⁄2 by 3 to get 3⁄2 cups.
📝 Note: Using real-world applications can help make fraction multiplication more meaningful and interesting.
In conclusion, mastering fraction multiplication requires practice and patience, but with the right strategies, anyone can become a pro. By understanding the basics and using techniques such as the traditional method, area model, number line method, grid method, and real-world application method, you can confidently multiply fractions and tackle more complex math problems.
What is the most common mistake when multiplying fractions?
+One of the most common mistakes when multiplying fractions is to add or subtract the numerators and denominators instead of multiplying them.
Can I multiply fractions with different denominators?
+Yes, you can multiply fractions with different denominators. Simply multiply the numerators and denominators separately and then simplify the resulting fraction.
How can I simplify a fraction after multiplying?
+To simplify a fraction after multiplying, divide the numerator and denominator by their greatest common divisor (GCD).