Mastering Fractions: 5 Ways to Convert Decimals & Percents

Understanding Fractions and Their Importance

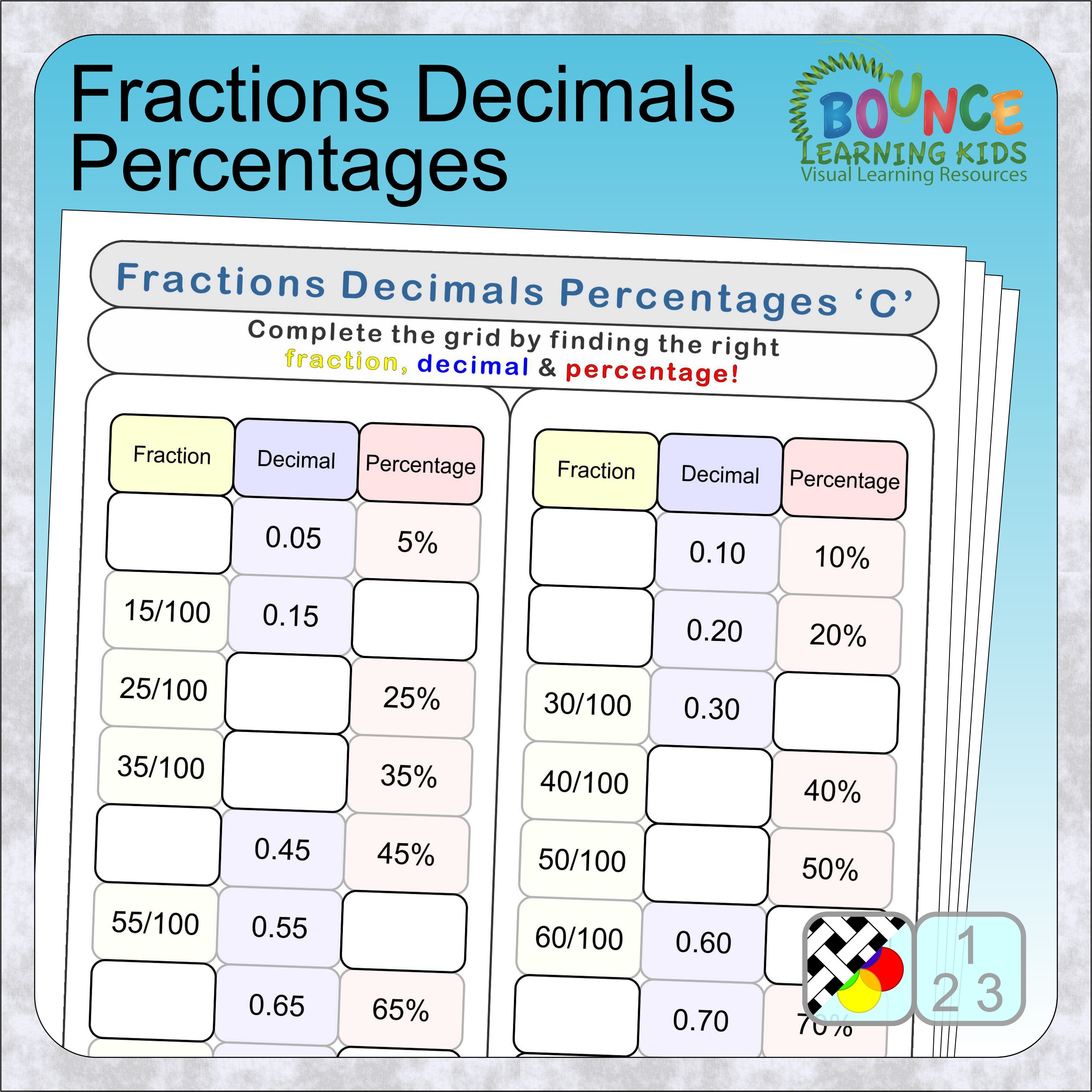
Fractions, decimals, and percents are essential components of mathematics, and mastering them is crucial for problem-solving in various areas of life, including science, finance, and cooking. While fractions might seem daunting at first, they can be easily understood and manipulated with practice. In this article, we will explore the basics of fractions, their relationship with decimals and percents, and provide step-by-step instructions on how to convert between these different forms.

What are Fractions?

A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into. For example, in the fraction ³⁄₄, the numerator is 3, and the denominator is 4.

Relationship between Fractions, Decimals, and Percents

Fractions, decimals, and percents are interconnected and can be easily converted into one another.

• Fractions to Decimals: To convert a fraction to a decimal, divide the numerator by the denominator. For example, ³⁄₄ can be converted to a decimal by dividing 3 by 4, which equals 0.75.
• Decimals to Percents: To convert a decimal to a percent, multiply the decimal by 100. For instance, 0.75 can be converted to a percent by multiplying it by 100, resulting in 75%.
• Percents to Fractions: To convert a percent to a fraction, divide the percent by 100 and simplify the resulting fraction. For example, 75% can be converted to a fraction by dividing 75 by 100, resulting in ³⁄₄.

5 Ways to Convert Decimals and Percents

1. Converting Decimals to Fractions

To convert decimals to fractions, we can use the following steps:

• Step 1: Write the decimal as a fraction with the decimal as the numerator and 1 as the denominator. For example, 0.75 can be written as ⁷⁵⁄₁.
• Step 2: Determine the place value of the last digit in the decimal. In this case, the last digit (5) is in the hundredths place.
• Step 3: Write the place value as the denominator. Since the last digit is in the hundredths place, the denominator will be 100.
• Step 4: Simplify the fraction, if possible. In this case, ⁷⁵⁄₁₀₀ can be simplified to ³⁄₄.

📝 Note: When converting decimals to fractions, make sure to simplify the fraction to its simplest form.

2. Converting Percents to Decimals

To convert percents to decimals, we can use the following steps:

• Step 1: Divide the percent by 100. For example, 75% can be converted to a decimal by dividing 75 by 100.
• Step 2: Write the result as a decimal. In this case, 75 ÷ 100 = 0.75.

📝 Note: When converting percents to decimals, make sure to divide the percent by 100.

3. Converting Fractions to Percents

To convert fractions to percents, we can use the following steps:

• Step 1: Convert the fraction to a decimal by dividing the numerator by the denominator. For example, ³⁄₄ can be converted to a decimal by dividing 3 by 4.
• Step 2: Multiply the decimal by 100. In this case, 0.75 can be converted to a percent by multiplying it by 100.
• Step 3: Write the result as a percent. In this case, 0.75 × 100 = 75%.

📝 Note: When converting fractions to percents, make sure to multiply the decimal by 100.

4. Converting Decimals to Percents

To convert decimals to percents, we can use the following steps:

• Step 1: Multiply the decimal by 100. For example, 0.75 can be converted to a percent by multiplying it by 100.
• Step 2: Write the result as a percent. In this case, 0.75 × 100 = 75%.

📝 Note: When converting decimals to percents, make sure to multiply the decimal by 100.

5. Converting Percents to Fractions

To convert percents to fractions, we can use the following steps:

• Step 1: Divide the percent by 100. For example, 75% can be converted to a fraction by dividing 75 by 100.
• Step 2: Write the result as a fraction. In this case, 75 ÷ 100 = ³⁄₄.

📝 Note: When converting percents to fractions, make sure to simplify the fraction to its simplest form.

Common Applications of Fractions, Decimals, and Percents

Fractions, decimals, and percents have numerous applications in real-life scenarios, including:

• Cooking: Recipes often involve fractions, decimals, and percents when measuring ingredients.
• Finance: Interest rates, discounts, and investments involve percents and decimals.
• Science: Fractions and decimals are used to express measurements and quantities in various scientific fields.

In conclusion, mastering fractions, decimals, and percents is crucial for problem-solving in various areas of life. By understanding the relationship between these different forms and practicing conversions, you can become proficient in working with fractions, decimals, and percents.