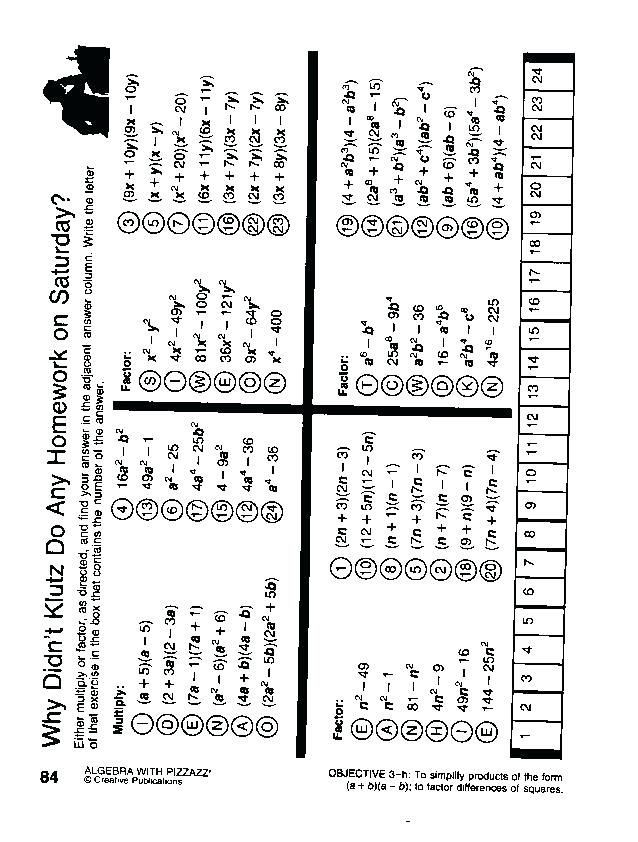
5 Ways to Master Factorization Tree Worksheets
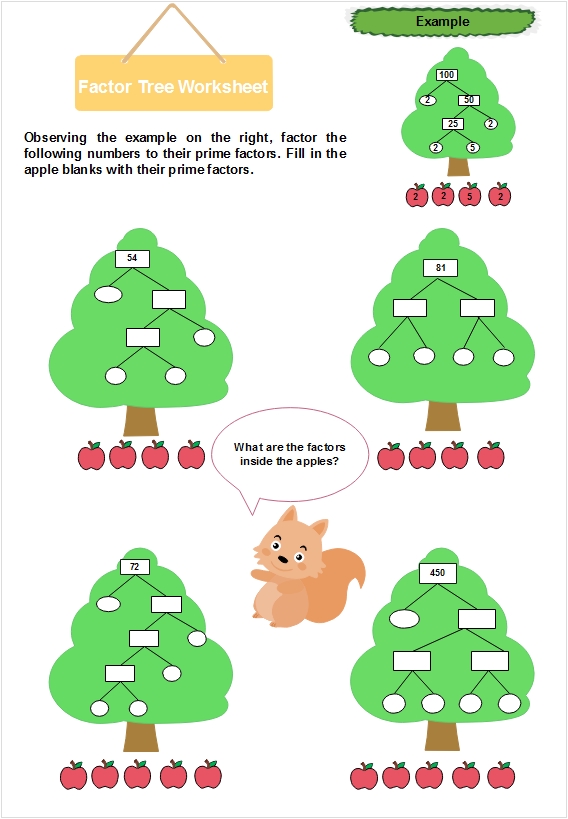
Unlocking the Secrets of Factorization Tree Worksheets
Factorization tree worksheets can be a daunting task for many students, but with the right strategies and practice, anyone can master them. In this article, we’ll explore five effective ways to tackle factorization tree worksheets and become a pro in no time.
Understanding the Basics of Factorization Trees
Before diving into the strategies, it’s essential to understand what factorization trees are and how they work. A factorization tree is a visual representation of the factors of a number, using a tree-like structure. Each branch of the tree represents a factor, and the leaves represent the prime factors. Factorization trees are an excellent tool for finding the prime factorization of a number.
1. Start with Simple Factorization Trees
Begin with simple factorization trees, using small numbers like 12, 18, or 24. These numbers have fewer factors, making it easier to visualize the tree. Practice building the tree by finding the factors of the number, and then branching out to the prime factors.
Example: Factorization tree for 12
| Factor | Prime Factors |
|---|---|
| 12 | 2 x 2 x 3 |
| 6 | 2 x 3 |
| 4 | 2 x 2 |
| 3 | 3 |
📝 Note: Start with numbers that have fewer factors to build your confidence and understanding of factorization trees.
2. Use the "Branching Out" Method
The “branching out” method involves finding the factors of a number and then branching out to the prime factors. Start by finding the factors of the number, and then divide each factor into its prime factors.
Example: Factorization tree for 24
| Factor | Prime Factors |
|---|---|
| 24 | 2 x 2 x 2 x 3 |
| 12 | 2 x 2 x 3 |
| 8 | 2 x 2 x 2 |
| 6 | 2 x 3 |
| 4 | 2 x 2 |
| 3 | 3 |
🔍 Note: Use the "branching out" method to visualize the factorization tree and find the prime factors of a number.
3. Practice with Different Types of Numbers
Practice building factorization trees with different types of numbers, such as odd numbers, even numbers, and numbers with multiple factors. This will help you develop a deeper understanding of factorization trees and improve your problem-solving skills.
Examples:
- Factorization tree for 27 (odd number)
- Factorization tree for 36 (even number)
- Factorization tree for 48 (number with multiple factors)
4. Use Real-World Applications
Using real-world applications can make factorization trees more interesting and relevant. For example, you can use factorization trees to find the greatest common divisor (GCD) of two numbers or to simplify fractions.
Example: Finding the GCD of 12 and 18 using factorization trees
| Factor | Prime Factors |
|---|---|
| 12 | 2 x 2 x 3 |
| 18 | 2 x 3 x 3 |
| GCD | 2 x 3 |
📊 Note: Use real-world applications to make factorization trees more engaging and relevant.
5. Create Your Own Factorization Tree Worksheets
Creating your own factorization tree worksheets can help you develop a deeper understanding of the concept. Choose a number and create a factorization tree, and then try to solve it. You can also create worksheets for your friends or classmates to practice.
Tips:
- Choose numbers with multiple factors to make it more challenging.
- Use different types of numbers, such as odd numbers, even numbers, and numbers with multiple factors.
- Create worksheets with different levels of difficulty to suit different skill levels.
By following these five strategies, you’ll become a master of factorization tree worksheets in no time. Remember to start with simple factorization trees, use the “branching out” method, practice with different types of numbers, use real-world applications, and create your own worksheets.
As you become more confident and proficient in building factorization trees, you’ll develop a deeper understanding of the concept and improve your problem-solving skills. Happy practicing!
What is a factorization tree?
+
A factorization tree is a visual representation of the factors of a number, using a tree-like structure. Each branch of the tree represents a factor, and the leaves represent the prime factors.
How do I build a factorization tree?
+
To build a factorization tree, start by finding the factors of a number, and then branch out to the prime factors. Use the “branching out” method to visualize the tree.
What are some real-world applications of factorization trees?
+
Factorization trees can be used to find the greatest common divisor (GCD) of two numbers or to simplify fractions. They can also be used in cryptography and coding theory.