Factor Quadratic Expressions with Ease

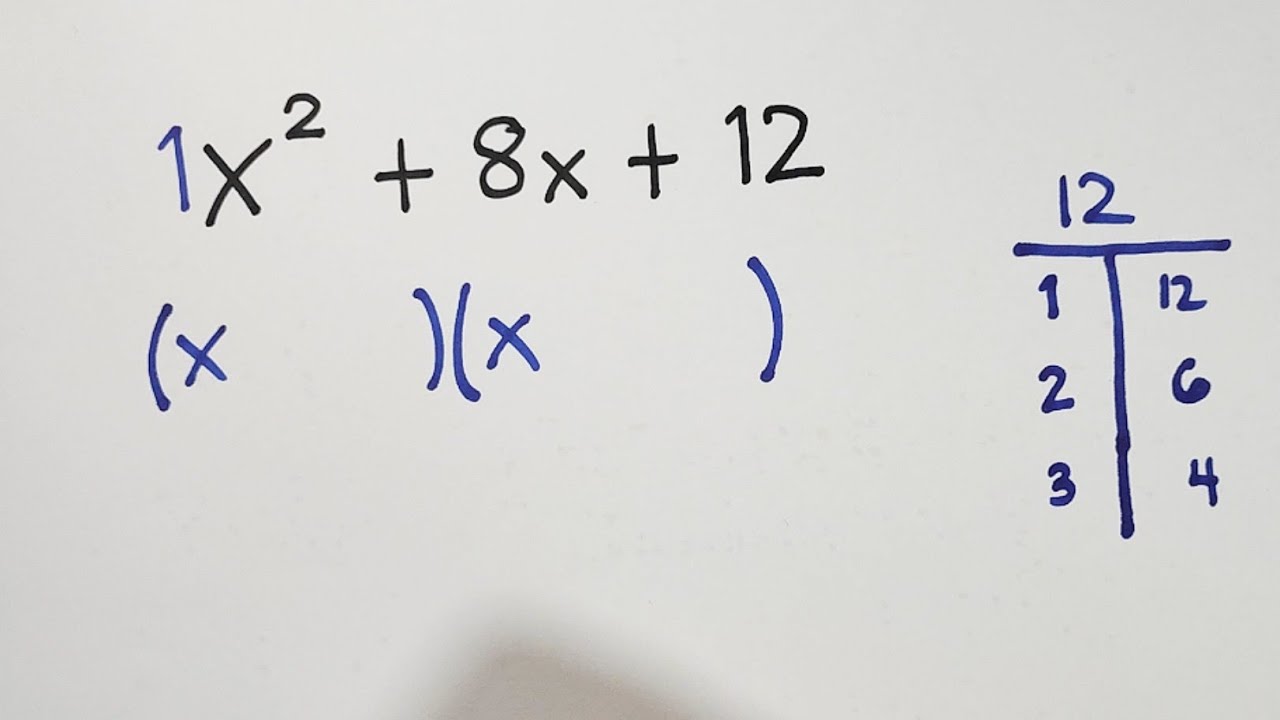
Understanding Quadratic Expressions

Quadratic expressions are polynomial expressions that involve a squared variable, typically in the form of ax^2 + bx + c, where a, b, and c are constants. These expressions are used to model a wide range of real-world phenomena, from the trajectory of projectiles to the growth of populations. However, working with quadratic expressions can be challenging, especially when it comes to factoring.

The Importance of Factoring Quadratic Expressions

Factoring quadratic expressions is a crucial skill in algebra, as it allows us to solve equations, simplify expressions, and even find the roots of quadratic functions. By factoring, we can break down complex expressions into simpler components, making it easier to work with them.

Methods for Factoring Quadratic Expressions

There are several methods for factoring quadratic expressions, each with its own strengths and weaknesses. Here are some of the most common methods:

1. Factoring by Grouping

Factoring by grouping involves grouping the terms of the quadratic expression into pairs, and then factoring out the greatest common factor (GCF) from each pair.

Example: Factor the quadratic expression x^2 + 5x + 6

• Group the terms: (x^2 + 3x) + (2x + 6)
• Factor out the GCF: x(x + 3) + 2(x + 3)
• Factor out the common binomial factor: (x + 2)(x + 3)

2. Factoring by Using the Quadratic Formula

The quadratic formula can be used to factor quadratic expressions by finding the roots of the quadratic function.

Example: Factor the quadratic expression x^2 + 4x + 4

• Use the quadratic formula: x = (-b ± √(b^2 - 4ac)) / 2a
• Simplify the expression: x = (-4 ± √(16 - 16)) / 2
• Factor the expression: (x + 2)(x + 2)

3. Factoring by Finding the Greatest Common Factor

Factoring by finding the greatest common factor (GCF) involves finding the largest factor that divides all the terms of the quadratic expression.

Example: Factor the quadratic expression 2x^2 + 6x + 4

• Find the GCF: 2
• Factor out the GCF: 2(x^2 + 3x + 2)

Common Mistakes to Avoid When Factoring Quadratic Expressions

When factoring quadratic expressions, there are several common mistakes to avoid:

• Forgetting to factor out the GCF: Make sure to factor out the greatest common factor from all the terms.
• Not checking for common binomial factors: Look for common binomial factors, such as (x + a), that can be factored out.
• Using the wrong method: Choose the correct method for factoring, depending on the type of quadratic expression.

📝 Note: Factoring quadratic expressions requires practice and patience. Start with simple expressions and gradually move on to more complex ones.

Real-World Applications of Factoring Quadratic Expressions

Factoring quadratic expressions has numerous real-world applications, from physics and engineering to economics and computer science. Here are a few examples:

• Projectile Motion: Quadratic expressions can be used to model the trajectory of projectiles, taking into account factors such as gravity and air resistance.
• Electrical Circuits: Quadratic expressions can be used to analyze electrical circuits, including resistors, capacitors, and inductors.
• Data Analysis: Quadratic expressions can be used to model and analyze data, including population growth and financial trends.

Conclusion

Factoring quadratic expressions is a fundamental skill in algebra, with numerous real-world applications. By mastering the different methods for factoring, including factoring by grouping, using the quadratic formula, and finding the greatest common factor, you can simplify complex expressions and solve equations with ease. Remember to avoid common mistakes and practice regularly to become proficient in factoring quadratic expressions.