5 Easy Ways to Master Factoring Problems
Understanding Factoring
Factoring is a fundamental concept in mathematics, particularly in algebra, that involves expressing an algebraic expression as a product of simpler expressions, called factors. Mastering factoring is essential for solving various types of equations and problems in mathematics. However, factoring can be a challenging topic for many students. In this article, we will explore five easy ways to master factoring problems.
Method 1: Greatest Common Factor (GCF)
The greatest common factor (GCF) method is one of the simplest and most effective ways to factor algebraic expressions. This method involves finding the greatest common factor of the terms in the expression and factoring it out.
Example: Factor the expression 12x + 18
- Find the GCF of 12 and 18, which is 6
- Factor out the GCF: 6(2x + 3)
📝 Note: When factoring out the GCF, make sure to divide each term by the GCF to obtain the correct factors.
Method 2: Difference of Squares
The difference of squares method is used to factor expressions of the form a^2 - b^2. This method involves applying the formula (a - b)(a + b) to the expression.
Example: Factor the expression x^2 - 16
- Identify the expression as a difference of squares: x^2 - 4^2
- Apply the formula: (x - 4)(x + 4)
Method 3: Sum and Difference of Cubes
The sum and difference of cubes method is used to factor expressions of the form a^3 + b^3 or a^3 - b^3. This method involves applying the formulas (a + b)(a^2 - ab + b^2) and (a - b)(a^2 + ab + b^2) respectively.
Example: Factor the expression x^3 + 8
- Identify the expression as a sum of cubes: x^3 + 2^3
- Apply the formula: (x + 2)(x^2 - 2x + 4)
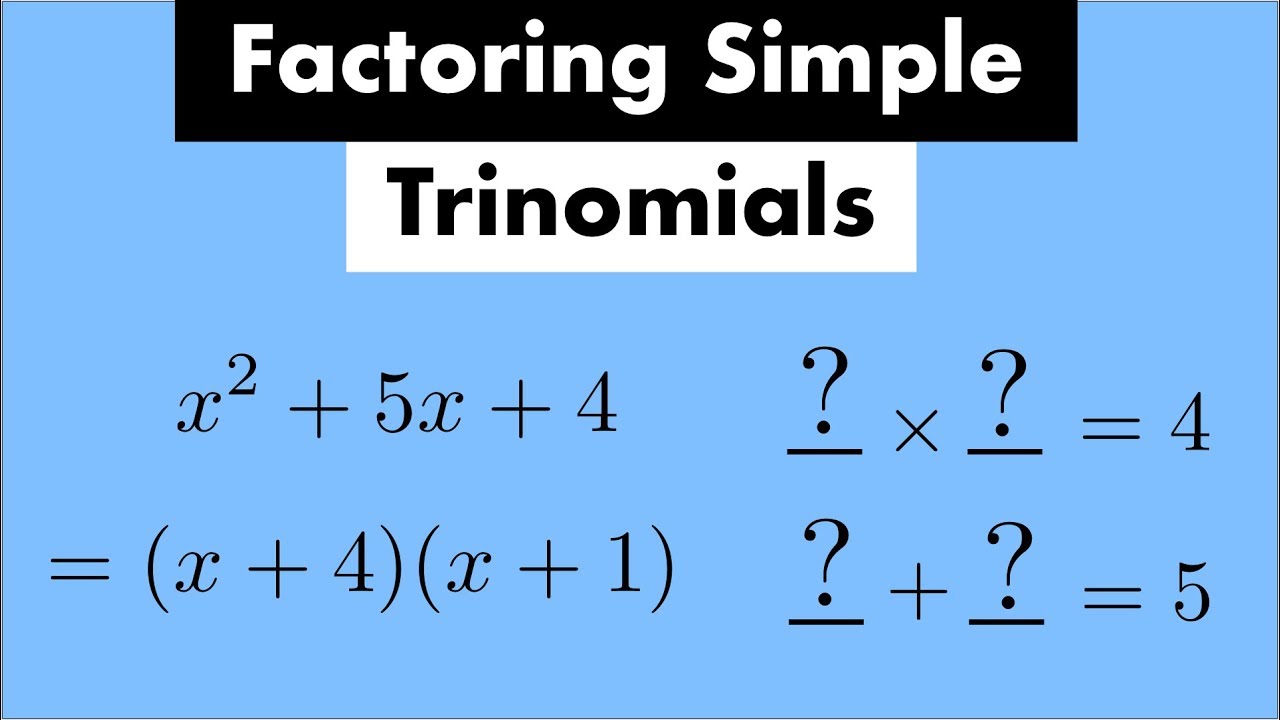
Method 4: Factoring Quadratic Expressions
Factoring quadratic expressions involves expressing a quadratic expression in the form ax^2 + bx + c as a product of two binomial factors. This method involves finding two numbers whose product is ac and whose sum is b.
Example: Factor the expression x^2 + 5x + 6
- Find two numbers whose product is 6 and whose sum is 5: 2 and 3
- Write the factored form: (x + 2)(x + 3)
Method 5: Factoring by Grouping
Factoring by grouping involves factoring expressions by grouping terms that have common factors. This method involves factoring out the greatest common factor of each group and then factoring out the common factor from the groups.
Example: Factor the expression x^2 + 3x + 2x + 6
- Group the terms: (x^2 + 3x) + (2x + 6)
- Factor out the GCF of each group: x(x + 3) + 2(x + 3)
- Factor out the common factor: (x + 2)(x + 3)
Mastering factoring problems requires practice and patience. By applying these five easy methods, you can simplify and solve various types of equations and problems in mathematics.
What is the difference between factoring and solving equations?
+
Factoring involves expressing an algebraic expression as a product of simpler expressions, while solving equations involves finding the value of the variable that satisfies the equation.
How do I know which factoring method to use?
+
The choice of factoring method depends on the type of expression and the numbers involved. For example, if the expression is a difference of squares, use the difference of squares method.
Can I use factoring to solve quadratic equations?
+
Yes, factoring can be used to solve quadratic equations. If the quadratic expression can be factored, then the equation can be solved by setting each factor equal to zero and solving for the variable.