5 Easy Ways to Factor Perfect Squares
Understanding Perfect Squares
Perfect squares are numbers that can be expressed as the square of an integer, i.e., a number that can be obtained by squaring an integer. For example, 4, 9, 16, and 25 are perfect squares because they can be expressed as the square of integers 2, 3, 4, and 5, respectively. Factoring perfect squares is an essential concept in algebra, and it can be used to simplify expressions, solve equations, and manipulate algebraic expressions.
Why Factor Perfect Squares?
Factoring perfect squares is crucial in various mathematical operations, such as:
- Simplifying expressions: Factoring perfect squares can help simplify complex expressions and make them easier to work with.
- Solving equations: Factoring perfect squares can be used to solve quadratic equations and other types of equations.
- Manipulating algebraic expressions: Factoring perfect squares can be used to manipulate algebraic expressions and rewrite them in a more convenient form.
5 Easy Ways to Factor Perfect Squares
Factoring perfect squares can be done in several ways, and here are five easy methods:
1. Recognition Method
This method involves recognizing perfect squares and factoring them directly. For example, 16 can be factored as 4 × 4 or 2 × 2 × 2 × 2.
📝 Note: This method requires a good understanding of perfect squares and their factors.
2. Using the Formula
The formula for factoring perfect squares is:
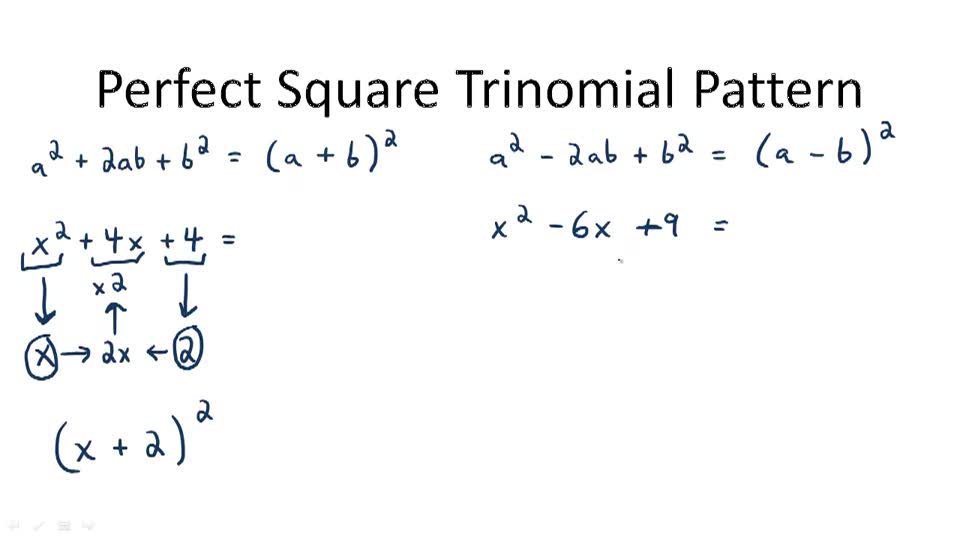
a^2 + 2ab + b^2 = (a + b)^2
Using this formula, we can factor perfect squares by identifying the values of a and b. For example, x^2 + 6x + 9 can be factored as (x + 3)^2.
3. Factoring by Grouping
This method involves grouping the terms of the perfect square and factoring them separately. For example, x^2 + 5x + 6 can be factored by grouping the terms as (x^2 + 5x) + 6, and then factoring the expression as (x + 2)(x + 3).
📝 Note: This method requires a good understanding of grouping and factoring techniques.
4. Using the Square Root Method
This method involves finding the square root of the perfect square and using it to factor the expression. For example, √(x^2 + 9) = x + 3.
5. Using Algebraic Manipulation
This method involves manipulating the perfect square using algebraic techniques, such as expanding or factoring expressions. For example, (x + 2)^2 can be expanded as x^2 + 4x + 4, and then factored as (x + 2)^2.
| Method | Description |
|---|---|
| Recognition Method | Recognizing perfect squares and factoring them directly |
| Using the Formula | Using the formula a^2 + 2ab + b^2 = (a + b)^2 to factor perfect squares |
| Factoring by Grouping | Grouping the terms of the perfect square and factoring them separately |
| Using the Square Root Method | Finding the square root of the perfect square and using it to factor the expression |
| Using Algebraic Manipulation | Manipulating the perfect square using algebraic techniques, such as expanding or factoring expressions |
In conclusion, factoring perfect squares is an essential concept in algebra, and there are several easy ways to do it. By understanding the different methods and techniques, you can simplify expressions, solve equations, and manipulate algebraic expressions with ease.
What is a perfect square?
+A perfect square is a number that can be expressed as the square of an integer, i.e., a number that can be obtained by squaring an integer.
Why is factoring perfect squares important?
+Factoring perfect squares is important because it can be used to simplify expressions, solve equations, and manipulate algebraic expressions.
What are some common methods for factoring perfect squares?
+Some common methods for factoring perfect squares include the recognition method, using the formula, factoring by grouping, using the square root method, and using algebraic manipulation.