Exponents Rules Worksheet: Simplify Expressions with Ease
Mastering Exponents Rules: A Comprehensive Guide to Simplifying Expressions
Exponents are a fundamental concept in mathematics, and understanding the rules that govern their behavior is essential for simplifying expressions and solving equations. In this article, we will delve into the world of exponents, exploring the key rules and principles that will help you simplify expressions with ease.
What are Exponents?
Exponents are shorthand notation for repeated multiplication. For example, the expression 2^3 represents 2 multiplied by itself three times: 2 × 2 × 2 = 8. Exponents can be used to represent a wide range of mathematical operations, from simple multiplication to complex algebraic equations.
Exponents Rules
There are several key rules that govern the behavior of exponents. These rules are essential for simplifying expressions and solving equations.
Rule 1: Product of Powers
When multiplying two powers with the same base, add the exponents.
Example: 2^2 × 2^3 = 2^(2+3) = 2^5
Rule 2: Power of a Power
When raising a power to another power, multiply the exponents.
Example: (2^2)^3 = 2^(2×3) = 2^6
Rule 3: Power of a Product
When raising a product to a power, raise each factor to that power.
Example: (2 × 3)^2 = 2^2 × 3^2
Rule 4: Zero Exponent
Any number raised to the power of zero is equal to 1.
Example: 2^0 = 1
Rule 5: Negative Exponent
A negative exponent represents the reciprocal of the base raised to the positive exponent.
Example: 2^(-3) = 1 / 2^3
Simplifying Expressions with Exponents
Now that we have explored the key rules that govern exponents, let’s put them into practice by simplifying some expressions.
Example 1: Simplify the expression 2^2 × 2^3.
Using the product of powers rule, we add the exponents: 2^2 × 2^3 = 2^(2+3) = 2^5.
Example 2: Simplify the expression (2^2)^3.
Using the power of a power rule, we multiply the exponents: (2^2)^3 = 2^(2×3) = 2^6.
Example 3: Simplify the expression (2 × 3)^2.
Using the power of a product rule, we raise each factor to the power: (2 × 3)^2 = 2^2 × 3^2.
Common Mistakes to Avoid
When working with exponents, there are several common mistakes to avoid.
[😊] Note: When multiplying powers with the same base, do not multiply the exponents. Instead, add the exponents.
[🤔] Note: When raising a power to another power, do not add the exponents. Instead, multiply the exponents.
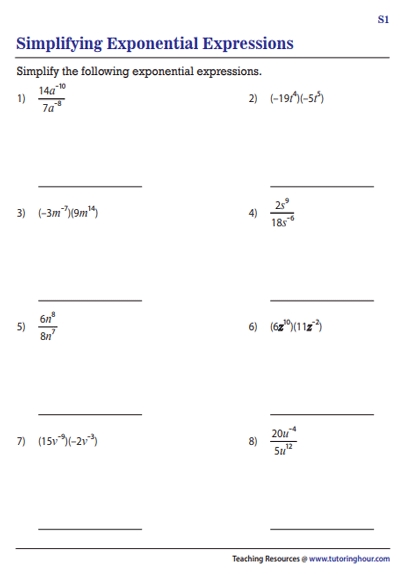
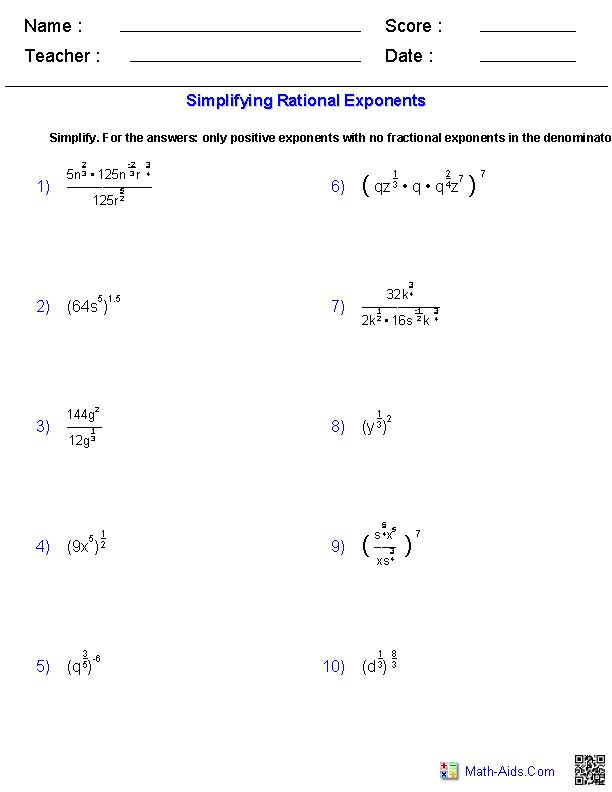
Exponents Rules Worksheet
Practice makes perfect! To help you master the exponents rules, we have put together a worksheet with some examples to try.
| Expression | Simplified Expression |
|---|---|
| 2^2 × 2^3 | 2^5 |
| (2^2)^3 | 2^6 |
| (2 × 3)^2 | 2^2 × 3^2 |
| 2^0 | 1 |
| 2^(-3) | 1 / 2^3 |
Conclusion
In conclusion, mastering the exponents rules is essential for simplifying expressions and solving equations. By understanding the key rules that govern exponents, you can simplify complex expressions with ease. Remember to avoid common mistakes, such as multiplying exponents instead of adding them, and practice regularly to become proficient in working with exponents.
What is the product of powers rule?
+The product of powers rule states that when multiplying two powers with the same base, add the exponents.
What is the power of a power rule?
+The power of a power rule states that when raising a power to another power, multiply the exponents.
What is the zero exponent rule?
+The zero exponent rule states that any number raised to the power of zero is equal to 1.
Related Terms:
- Exponent Worksheet
- Exponent exercises pdf
- Exponents worksheets PDF with answers
- Exponent pdf
- Exponent rule