5 Ways to Master Exponential Growth and Decay
Understanding Exponential Growth and Decay
Exponential growth and decay are fundamental concepts in mathematics, finance, and science. These phenomena occur when a quantity changes at a rate proportional to its current value, resulting in a rapid increase or decrease over time. Mastering exponential growth and decay is crucial for analyzing and modeling various real-world situations, from population growth and compound interest to radioactive decay and chemical reactions. In this article, we will delve into the world of exponential growth and decay, exploring five ways to master these essential concepts.
1. Learn the Basics: Exponential Functions and Formulas
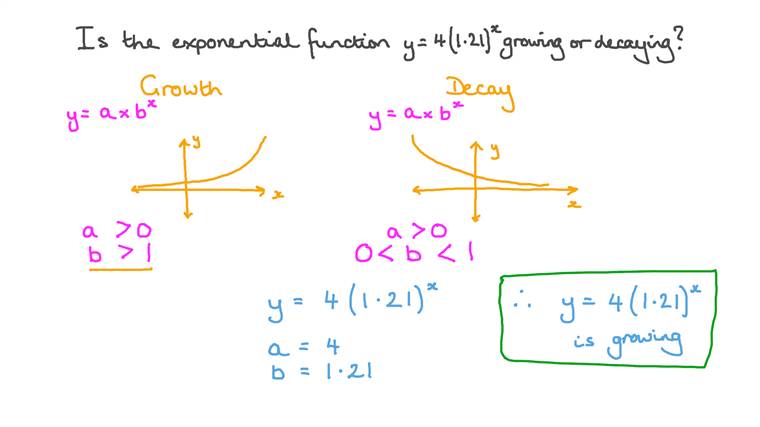
To grasp exponential growth and decay, it’s essential to understand the underlying mathematics. Exponential functions are defined by the formula:
f(x) = ab^x
where:
- a is the initial value or starting point
- b is the growth or decay factor (b > 0 for growth, 0 < b < 1 for decay)
- x is the independent variable (usually time)
Familiarize yourself with the basic properties of exponential functions, including:
- The exponential function is continuous and differentiable
- The function increases or decreases at a rate proportional to its current value
- The function can be represented graphically, with a characteristic curve shape
2. Visualize Exponential Growth and Decay with Graphs
Graphical representations are an excellent way to visualize exponential growth and decay. By plotting the function f(x) = ab^x, you can observe the characteristic curve shapes:
- Exponential growth: a > 1, the curve increases rapidly, with an accelerating rate of change
- Exponential decay: 0 < a < 1, the curve decreases rapidly, with a decelerating rate of change
Use graphing tools or software to explore different scenarios, adjusting the values of a, b, and x to see how the curve changes.
3. Practice with Real-World Examples and Applications
Exponential growth and decay have numerous real-world applications. Practice solving problems and analyzing scenarios in various fields, such as:
- Population growth: modeling the growth of a population, considering factors like birth rates and resource limitations
- Finance: calculating compound interest, investment returns, and loan repayments
- Science: modeling chemical reactions, radioactive decay, and epidemiology
4. Develop Your Skills with Interactive Tools and Resources
Take advantage of interactive tools and resources to deepen your understanding of exponential growth and decay. Utilize:
- Online calculators: explore exponential functions and visualize graph shapes
- Simulations: model real-world scenarios, adjusting parameters to observe the effects of exponential growth and decay
- Interactive lessons: engage with video tutorials, interactive exercises, and quizzes to reinforce your knowledge
📝 Note: Utilize online resources, such as Khan Academy, Mathway, or Wolfram Alpha, to supplement your learning.
5. Challenge Yourself with Advanced Topics and Word Problems
To truly master exponential growth and decay, challenge yourself with advanced topics and word problems. Explore:
- Differential equations: model exponential growth and decay using differential equations, and solve problems involving rates of change
- Word problems: tackle complex, real-world scenarios, applying exponential growth and decay concepts to solve problems in fields like economics, biology, and physics
By following these five steps, you’ll be well on your way to mastering exponential growth and decay. Remember to practice regularly, explore real-world applications, and challenge yourself with advanced topics to reinforce your understanding.
What is the difference between exponential growth and decay?
+Exponential growth occurs when a quantity increases at a rate proportional to its current value, resulting in rapid growth. Exponential decay occurs when a quantity decreases at a rate proportional to its current value, resulting in rapid decline.
How are exponential functions used in real-world applications?
+Exponential functions are used to model population growth, calculate compound interest, and analyze chemical reactions, among other applications.
What are some common pitfalls to avoid when working with exponential growth and decay?
+Common pitfalls include misunderstanding the concept of proportional growth or decay, failing to consider the initial value or starting point, and neglecting to adjust for changes in the growth or decay factor over time.
Related Terms:
- Graphing Exponential Functions Worksheet
- Exponential questions and answers pdf
- Exponential function pdf