Even and Odd Functions Worksheet Exercises

Understanding Even and Odd Functions
In mathematics, a function is considered even if it satisfies the condition f(x) = f(-x) for all x in its domain. On the other hand, a function is considered odd if it satisfies the condition f(-x) = -f(x) for all x in its domain. This distinction is crucial in various mathematical operations and applications. Here, we will explore the characteristics of even and odd functions, provide examples, and offer exercises to help solidify your understanding.
Characteristics of Even Functions
Even functions have symmetry about the y-axis. This means that if you were to fold the graph of an even function along the y-axis, the two halves would match perfectly. Some key properties of even functions include:
- f(x) = f(-x) for all x in the domain of the function.
- The graph of an even function is symmetric with respect to the y-axis.
- The integral of an even function from -a to a is equal to 2 times the integral of the function from 0 to a.
💡 Note: Even functions can be added and multiplied together to produce another even function.
Characteristics of Odd Functions
Odd functions have rotational symmetry of 180 degrees about the origin. This means that if you were to rotate the graph of an odd function by 180 degrees about the origin, the resulting graph would be identical to the original graph. Some key properties of odd functions include:
- f(-x) = -f(x) for all x in the domain of the function.
- The graph of an odd function is symmetric with respect to the origin.
- The integral of an odd function from -a to a is equal to 0.
🔄 Note: Odd functions can be added and multiplied together to produce another odd function.
Examples of Even and Odd Functions
Even Functions:
- f(x) = x^2
- f(x) = cos(x)
- f(x) = |x|
Odd Functions:
- f(x) = x^3
- f(x) = sin(x)
- f(x) = x
Exercises
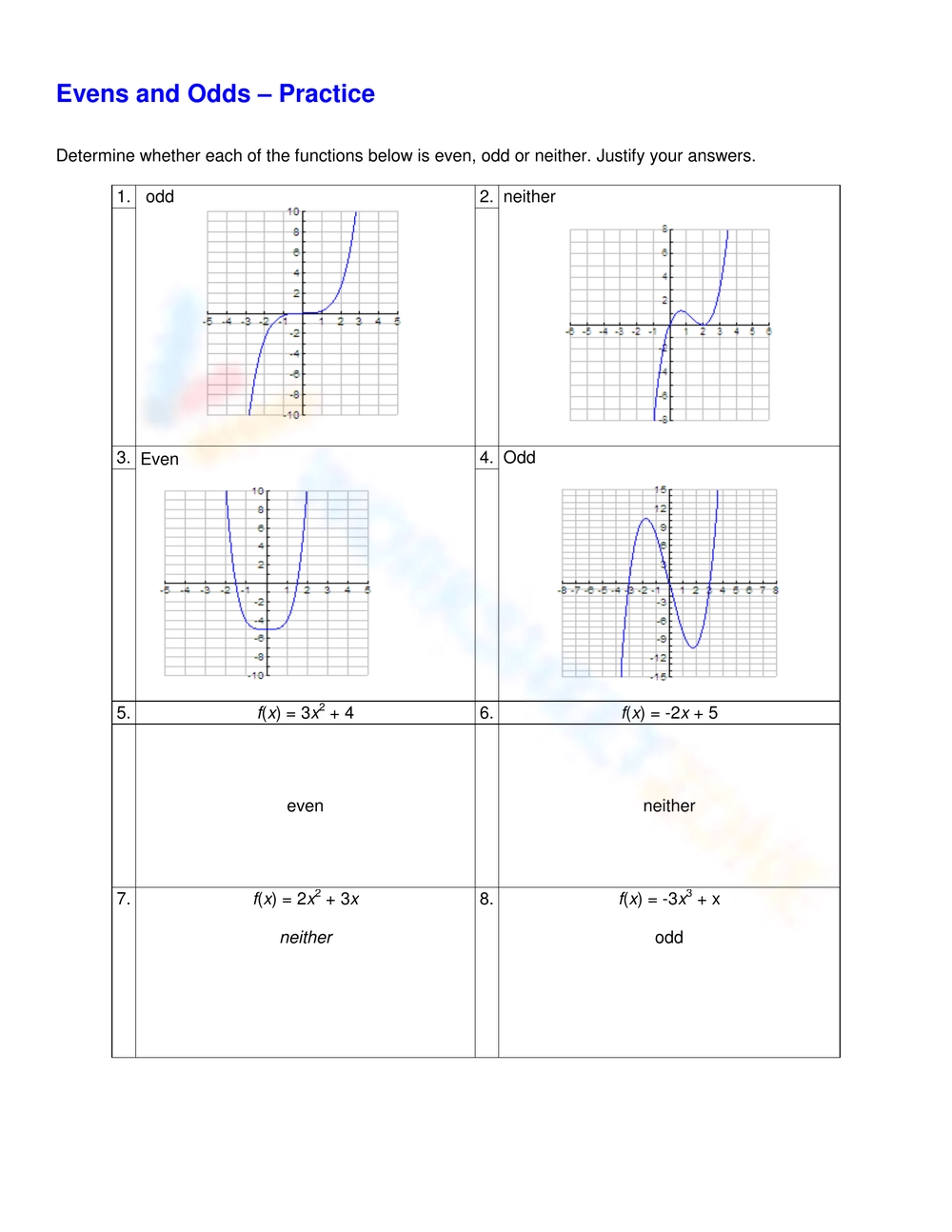
Determine whether each function is even, odd, or neither:
- f(x) = 2x^2 + 3x
- f(x) = x^3 - 2x
- f(x) = |x| + 1
- f(x) = sin(x) + cos(x)
- f(x) = x^4 - 2x^2
Solutions:
| Function | Even, Odd, or Neither |
|---|---|
| f(x) = 2x^2 + 3x | Neither |
| f(x) = x^3 - 2x | Odd |
| f(x) = | x |
| f(x) = sin(x) + cos(x) | Neither |
| f(x) = x^4 - 2x^2 | Even |
Graphical Analysis
Create graphs for the following functions to visualize their symmetry:
- f(x) = x^2
- f(x) = x^3
- f(x) = |x|
Observe the symmetry of each graph to determine whether it is even, odd, or neither.
Real-World Applications
Even and odd functions have numerous applications in various fields, including physics, engineering, and computer science. For example:
- In physics, the motion of a particle can be described using even and odd functions.
- In engineering, the design of electronic circuits often involves even and odd functions.
- In computer science, even and odd functions are used in algorithms for data compression and encryption.
As you can see, even and odd functions play a crucial role in many areas of mathematics and science.
Even and odd functions are fundamental concepts in mathematics, and understanding their properties and characteristics is essential for various applications. By recognizing the symmetry of functions, you can simplify mathematical operations and solve problems more efficiently.
Related Terms:
- 4 2 Even and odd Functions
- Even and odd functions activity