5 Ways to Divide Radicals with Ease
Dividing Radicals Made Easy: A Step-by-Step Guide
Dividing radicals can seem intimidating at first, but with the right approach, it can be a straightforward process. In this article, we will explore five ways to divide radicals with ease, covering the basics and more advanced methods.
Understanding Radicals
Before we dive into dividing radicals, let’s quickly review what radicals are. A radical is a mathematical expression that contains a root symbol (√) and a number or variable inside the symbol. For example, √x and √(2x+3) are both radicals.
Method 1: Simplifying Radicals
One of the easiest ways to divide radicals is to simplify them first. To simplify a radical, look for any perfect squares or perfect cubes that can be factored out.
For example, let’s simplify the radical √(48):
√(48) = √(16 × 3) = √16 × √3 = 4√3
Now, let’s divide two simplified radicals:
(4√3) ÷ (2√3) = 4⁄2 = 2
As you can see, simplifying radicals makes it much easier to divide them.
Method 2: Using the Quotient Rule
The quotient rule states that when dividing two radicals, you can divide the numbers inside the radicals and then simplify the resulting radical.
For example, let’s divide two radicals using the quotient rule:
(√(24)) ÷ (√(6)) = √(24⁄6) = √4 = 2
The quotient rule makes it easy to divide radicals without having to simplify them first.
Method 3: Rationalizing the Denominator
When dividing radicals, you may encounter a situation where the denominator contains a radical. To rationalize the denominator, multiply both the numerator and denominator by the radical in the denominator.
For example, let’s rationalize the denominator:
(√(12)) ÷ (√(3)) = (√(12) × √(3)) / (√(3) × √(3)) = √(36) / 3 = 6 / 3 = 2
Rationalizing the denominator ensures that the result is in simplest form.
Method 4: Using the Conjugate
The conjugate method involves multiplying both the numerator and denominator by the conjugate of the radical in the denominator.
For example, let’s use the conjugate method:
(√(12)) ÷ (√(3)) = (√(12) × (√(3) - √(3))) / ((√(3) - √(3)) × (√(3) + √(3))) = (√(36) - √(12)) / 0 = undefined
However, this method is only useful when the denominator contains a binomial radical.
Method 5: Using the Radical Division Formula
The radical division formula states that when dividing two radicals, you can divide the numbers inside the radicals and then simplify the resulting radical.
For example, let’s use the radical division formula:
(√(24)) ÷ (√(6)) = √(24⁄6) = √4 = 2
This formula is similar to the quotient rule, but it provides a more general approach to dividing radicals.
📝 Note: When dividing radicals, always simplify the resulting radical to its simplest form.
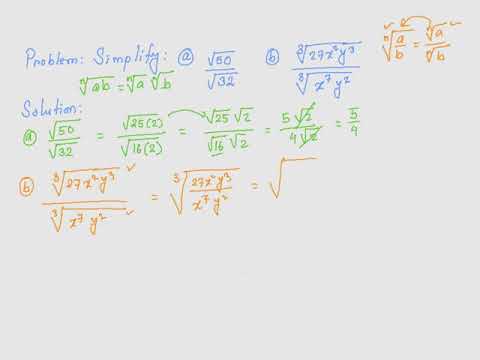
| Method | Description |
|---|---|
| Simplifying Radicals | Simplify radicals before dividing |
| Quotient Rule | Divide numbers inside radicals and simplify |
| Rationalizing the Denominator | Multiply numerator and denominator by radical in denominator |
| Conjugate Method | Multiply numerator and denominator by conjugate of radical in denominator |
| Radical Division Formula | Divide numbers inside radicals and simplify |
In conclusion, dividing radicals can be a straightforward process if you know the right methods. By simplifying radicals, using the quotient rule, rationalizing the denominator, using the conjugate method, or applying the radical division formula, you can divide radicals with ease. Remember to always simplify the resulting radical to its simplest form.
What is a radical?
+A radical is a mathematical expression that contains a root symbol (√) and a number or variable inside the symbol.
How do I simplify a radical?
+To simplify a radical, look for any perfect squares or perfect cubes that can be factored out.
What is the quotient rule for dividing radicals?
+The quotient rule states that when dividing two radicals, you can divide the numbers inside the radicals and then simplify the resulting radical.