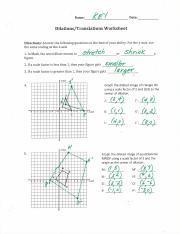
Dilation Translation Worksheet Answer Key
Dilation and Translation: Understanding Geometric Transformations
Geometry is a branch of mathematics that deals with the study of shapes, sizes, and positions of objects. Two fundamental concepts in geometry are dilation and translation. In this article, we will explore the concepts of dilation and translation, provide examples, and offer a worksheet with answers to help you understand these transformations better.
What is Dilation?
Dilation is a transformation that changes the size of a figure. In a dilation, the preimage (the original figure) is enlarged or reduced to create a new image. The scale factor determines how much the image is enlarged or reduced. A scale factor greater than 1 indicates an enlargement, while a scale factor less than 1 indicates a reduction.
Types of Dilation:
There are two types of dilation:
- Enlargement: When the scale factor is greater than 1, the image is larger than the preimage.
- Reduction: When the scale factor is less than 1, the image is smaller than the preimage.
What is Translation?
Translation is a transformation that moves a figure from one position to another without changing its size or shape. In a translation, the preimage is moved horizontally, vertically, or both, to create a new image.
Types of Translation:
There are three types of translation:
- Horizontal Translation: When the preimage is moved horizontally, the image is shifted left or right.
- Vertical Translation: When the preimage is moved vertically, the image is shifted up or down.
- Diagonal Translation: When the preimage is moved both horizontally and vertically, the image is shifted diagonally.
Dilation Translation Worksheet Answer Key
Here are some examples of dilation and translation problems, along with their solutions:
Dilation Examples

| Preimage | Scale Factor | Image |
|---|---|---|
| A triangle with a side length of 4 | 2 | A triangle with a side length of 8 |
| A circle with a radius of 3 | 1⁄2 | A circle with a radius of 1.5 |
| A rectangle with a width of 6 and a height of 4 | 3 | A rectangle with a width of 18 and a height of 12 |
Translation Examples
| Preimage | Translation | Image |
|---|---|---|
| A triangle with vertices (0, 0), (3, 0), and (0, 4) | (h, k) = (2, 3) | A triangle with vertices (2, 3), (5, 3), and (2, 7) |
| A circle with a center (2, 2) and a radius of 3 | (h, k) = (-1, 4) | A circle with a center (1, 6) and a radius of 3 |
| A rectangle with vertices (1, 1), (4, 1), (1, 3), and (4, 3) | (h, k) = (2, -1) | A rectangle with vertices (3, 0), (6, 0), (3, 2), and (6, 2) |
Combined Dilation and Translation Examples
| Preimage | Dilation Scale Factor | Translation | Image |
|---|---|---|---|
| A triangle with a side length of 4 | 2 | (h, k) = (1, 2) | A triangle with a side length of 8 and vertices (1, 2), (5, 2), and (1, 10) |
| A circle with a radius of 3 | 1⁄2 | (h, k) = (-2, 3) | A circle with a radius of 1.5 and a center (-2, 3) |
| A rectangle with a width of 6 and a height of 4 | 3 | (h, k) = (2, -1) | A rectangle with a width of 18 and a height of 12 and vertices (2, -1), (20, -1), (2, 11), and (20, 11) |
🔍 Note: The translation (h, k) = (a, b) means that the preimage is moved a units horizontally and b units vertically.
Dilation Translation Worksheet Answer Key Table
| Problem Number | Preimage | Dilation Scale Factor | Translation | Image |
|---|---|---|---|---|
| 1 | A triangle with a side length of 4 | 2 | (h, k) = (1, 2) | A triangle with a side length of 8 and vertices (1, 2), (5, 2), and (1, 10) |
| 2 | A circle with a radius of 3 | 1⁄2 | (h, k) = (-2, 3) | A circle with a radius of 1.5 and a center (-2, 3) |
| 3 | A rectangle with a width of 6 and a height of 4 | 3 | (h, k) = (2, -1) | A rectangle with a width of 18 and a height of 12 and vertices (2, -1), (20, -1), (2, 11), and (20, 11) |
| 4 | A triangle with vertices (0, 0), (3, 0), and (0, 4) | 1 | (h, k) = (2, 3) | A triangle with vertices (2, 3), (5, 3), and (2, 7) |
| 5 | A circle with a center (2, 2) and a radius of 3 | 1 | (h, k) = (-1, 4) | A circle with a center (1, 6) and a radius of 3 |
📝 Note: This worksheet is designed to help you practice dilation and translation transformations. You can use the answer key to check your work and understand the concepts better.
By practicing dilation and translation transformations, you can develop a deeper understanding of geometric transformations and improve your problem-solving skills. Remember to always check your work and use the answer key to ensure that you are on the right track.
What is the difference between dilation and translation?
+Dilation is a transformation that changes the size of a figure, while translation is a transformation that moves a figure from one position to another without changing its size or shape.
How do you perform a dilation transformation?
+To perform a dilation transformation, you need to multiply the coordinates of the preimage by the scale factor.
How do you perform a translation transformation?
+To perform a translation transformation, you need to add the translation values to the coordinates of the preimage.