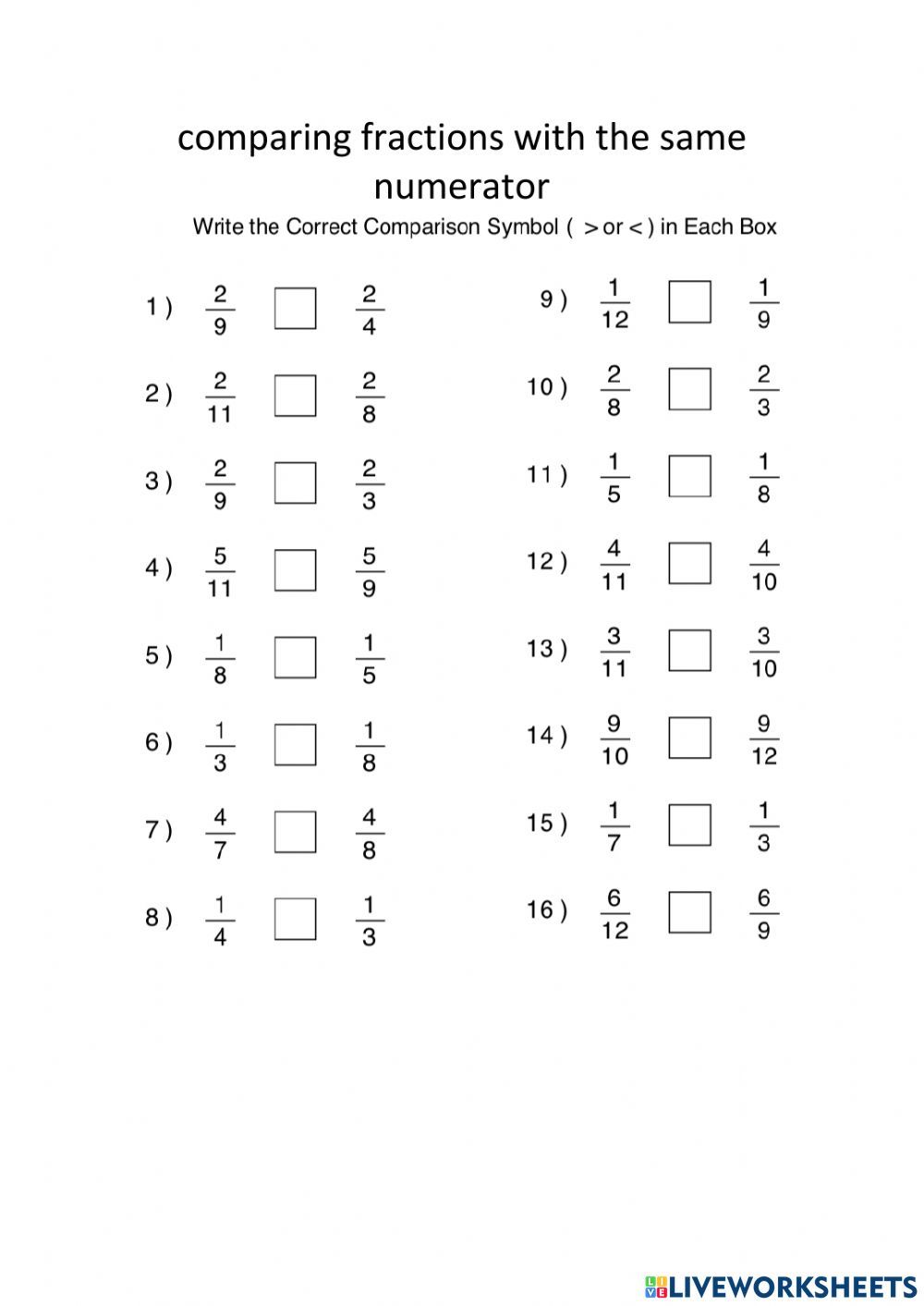
Comparing Fractions With Same Numerator Made Easy

Understanding Fractions

Fractions are a way to represent a part of a whole. They consist of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into. For example, in the fraction ³⁄₄, the numerator is 3 and the denominator is 4.

The Concept of Equivalent Fractions

Equivalent fractions are fractions that have the same value, but different numbers. For instance, ¹⁄₂ is equivalent to ²⁄₄, ³⁄₆, and so on. These fractions all represent the same part of a whole, but with different numerators and denominators.

Comparing Fractions with the Same Numerator

When comparing fractions with the same numerator, we need to look at the denominators. The fraction with the smaller denominator is larger. This is because the denominator tells us how many parts the whole is divided into, so if the denominator is smaller, each part is larger.

For example, let’s compare the fractions ³⁄₄ and ³⁄₆. Both fractions have the same numerator (3), but the denominators are different. Since 4 is less than 6, the fraction ³⁄₄ is larger.

Why Does This Work?

To understand why this works, let’s think about what the denominators represent. If we have a fraction with a numerator of 3 and a denominator of 4, it means we have 3 parts out of a total of 4 equal parts. If we have another fraction with the same numerator (3) but a denominator of 6, it means we have 3 parts out of a total of 6 equal parts. Since the parts are smaller when the denominator is larger, the fraction with the smaller denominator (³⁄₄) is larger.

Examples and Applications

Let’s look at some examples to illustrate this concept:

• Compare the fractions ²⁄₃ and ²⁄₅. Since the numerators are the same (2), we look at the denominators. 3 is less than 5, so the fraction ²⁄₃ is larger.
• Compare the fractions ⁴⁄₅ and ⁴⁄₇. Again, the numerators are the same (4), so we look at the denominators. 5 is less than 7, so the fraction ⁴⁄₅ is larger.

This concept is useful in real-life situations, such as:

• Measuring ingredients for a recipe. If you need ²⁄₃ cup of flour and you only have a ¹⁄₅ cup measuring cup, you’ll need to use more cups to get the right amount.
• Dividing a pizza among friends. If you have ²⁄₃ of a pizza left and you want to divide it among 4 people, you’ll need to cut it into smaller slices to make sure everyone gets an equal share.

Notes

📝 Note: When comparing fractions with the same numerator, it's essential to remember that the fraction with the smaller denominator is larger.

Conclusion

Comparing fractions with the same numerator is a straightforward process. By looking at the denominators, we can determine which fraction is larger. This concept is crucial in various real-life situations, such as cooking and sharing. By mastering this concept, you’ll become more confident in your ability to work with fractions.

Related Terms:

• Comparing fractions same denominator Worksheet
• Comparing fractions with like numerators
• Comparing fractions Worksheet with answers
• Comparing fractions grade 2 worksheets