5 Ways to Compare Decimals

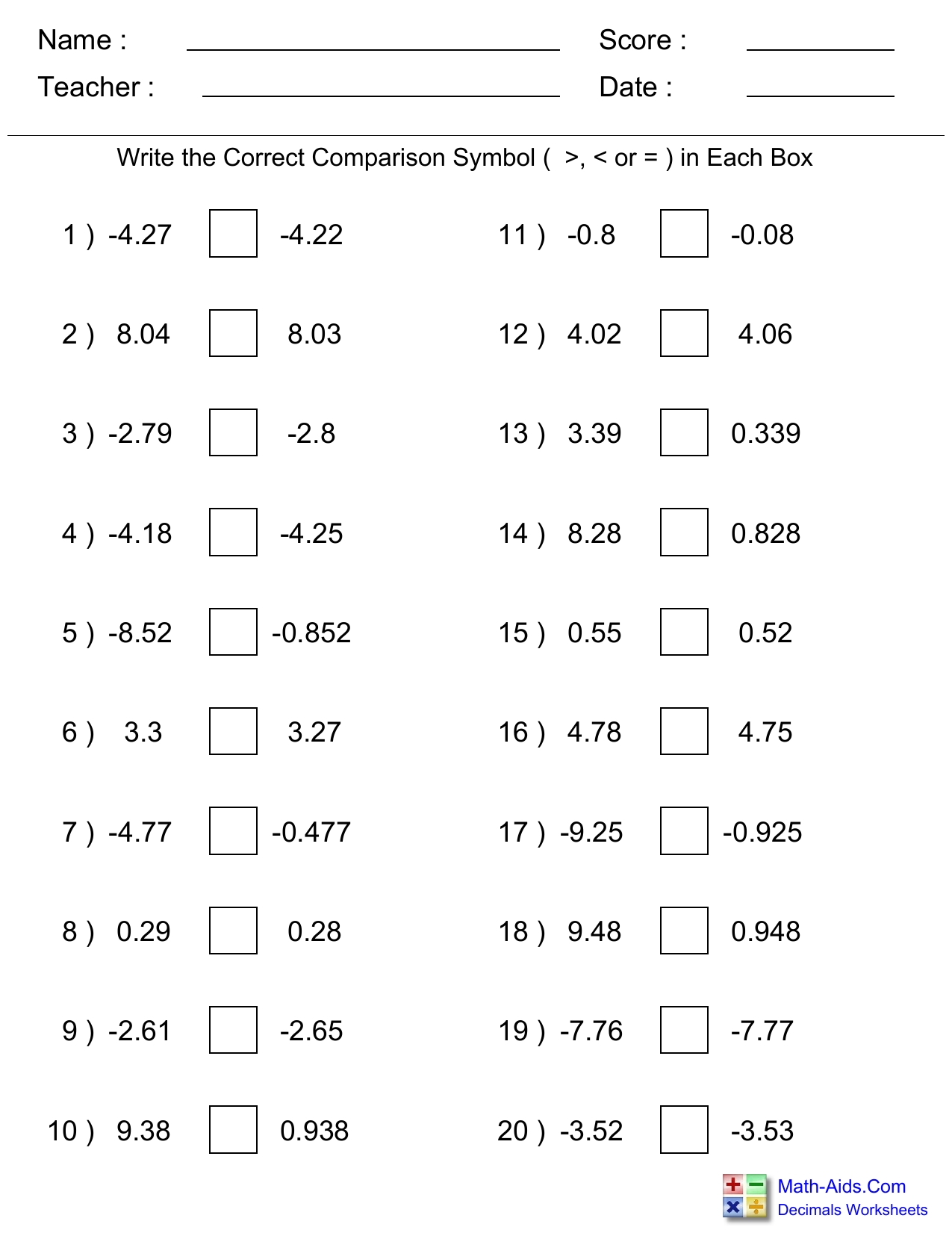
Understanding Decimals and Their Comparisons

Decimals are a fundamental concept in mathematics, representing a way to express fractions in a more readable and manageable form. When dealing with decimals, one of the essential skills is comparing them to determine which is larger or smaller. This comparison is crucial in various mathematical operations, problem-solving, and even in real-world applications such as finance, science, and engineering. In this article, we will explore five methods to compare decimals effectively.

Method 1: Comparing Decimals by Counting Places

One of the simplest ways to compare decimals is by looking at the number of places after the decimal point and then comparing the digits in each place. This method is straightforward and works well for decimals with the same number of places.

📝 Note: When comparing decimals, make sure they have the same number of decimal places. If not, add zeros to the right of the decimal to make them equal in length.

For example, to compare 4.25 and 4.26, we can look at the digits after the decimal point. Both have two places, so we compare the digits in each place:

• 4.25: 2 (in the hundredths place) vs. 4.26: 6 (in the hundredths place)
• Since 6 is greater than 2, 4.26 is larger than 4.25.

Method 2: Using Number Lines

Another visual method to compare decimals is by using a number line. A number line is a straight line with numbers marked at intervals, which helps in understanding the relative position of numbers.

To compare decimals using a number line:

1. Draw a number line with intervals marked (e.g., by tenths or hundredths).
2. Place the decimals on the number line according to their value.
3. Compare the position of the decimals on the number line to determine which is larger.

For instance, to compare 2.5 and 2.7 on a number line marked by tenths:

• 2.5 would be placed halfway between 2 and 3.
• 2.7 would be placed further to the right, closer to 3.
• Since 2.7 is to the right of 2.5 on the number line, 2.7 is larger.

Method 3: Converting Decimals to Fractions

Decimals can be converted into fractions, which sometimes makes comparison easier, especially when dealing with familiar fractions.

To compare decimals by converting them to fractions:

1. Convert each decimal into a fraction.
2. Compare the fractions.

For example, to compare 0.5 and 0.25:

• 0.5 converts to ¹⁄₂.
• 0.25 converts to ¹⁄₄.
• Since ¹⁄₂ is larger than ¹⁄₄, 0.5 is larger than 0.25.

Method 4: Using Inequalities

Inequalities can also be used to compare decimals. This method involves setting up an inequality and solving it to determine the relationship between the decimals.

For example, to compare 3.14 and 3.15 using inequalities:

• If we want to know if 3.14 is less than 3.15, we set up the inequality: 3.14 < 3.15.
• Since 3.14 is indeed less than 3.15, the statement is true, indicating 3.14 is less than 3.15.

Method 5: Technology and Calculators

In today’s digital age, technology and calculators offer a quick and efficient way to compare decimals.

To compare decimals using a calculator:

1. Enter the first decimal into the calculator.
2. Enter the second decimal into the calculator.
3. Use the comparison function (e.g., =, <, >) to compare the decimals.
4. The calculator will display the result of the comparison.

For instance, to compare 2.56 and 2.57 using a calculator:

• Enter 2.56 and 2.57 into the calculator with the comparison function.
• The calculator will indicate if one is larger, smaller, or equal to the other.

Summary of comparing decimals involves various methods including comparing places, using number lines, converting to fractions, inequalities, and technology. Each method has its own advantages and can be used in different situations to determine which decimal is larger or smaller.