Compare Fractions Made Easy With This Worksheet Guide
Mastering Fractions: A Comprehensive Guide
Comparing fractions can be a daunting task for many students, but with the right tools and techniques, it can be made easy. In this guide, we will walk you through a step-by-step approach to comparing fractions using worksheets as a learning aid.
Understanding Fractions
Before we dive into comparing fractions, it’s essential to understand the basics. A fraction is a way of expressing a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). For example, in the fraction 3⁄4, the numerator is 3, and the denominator is 4.
Types of Fractions
There are three main types of fractions:
- Proper fractions: These are fractions where the numerator is less than the denominator. Examples include 1⁄2, 3⁄4, and 2⁄3.
- Improper fractions: These are fractions where the numerator is greater than or equal to the denominator. Examples include 3⁄2, 5⁄4, and 7⁄6.
- Mixed numbers: These are fractions that include a whole number and a proper fraction. Examples include 2 1⁄2, 3 3⁄4, and 1 1⁄3.
Comparing Fractions: A Step-by-Step Approach
Comparing fractions involves determining which fraction is larger or smaller than the other. Here’s a step-by-step guide to comparing fractions:
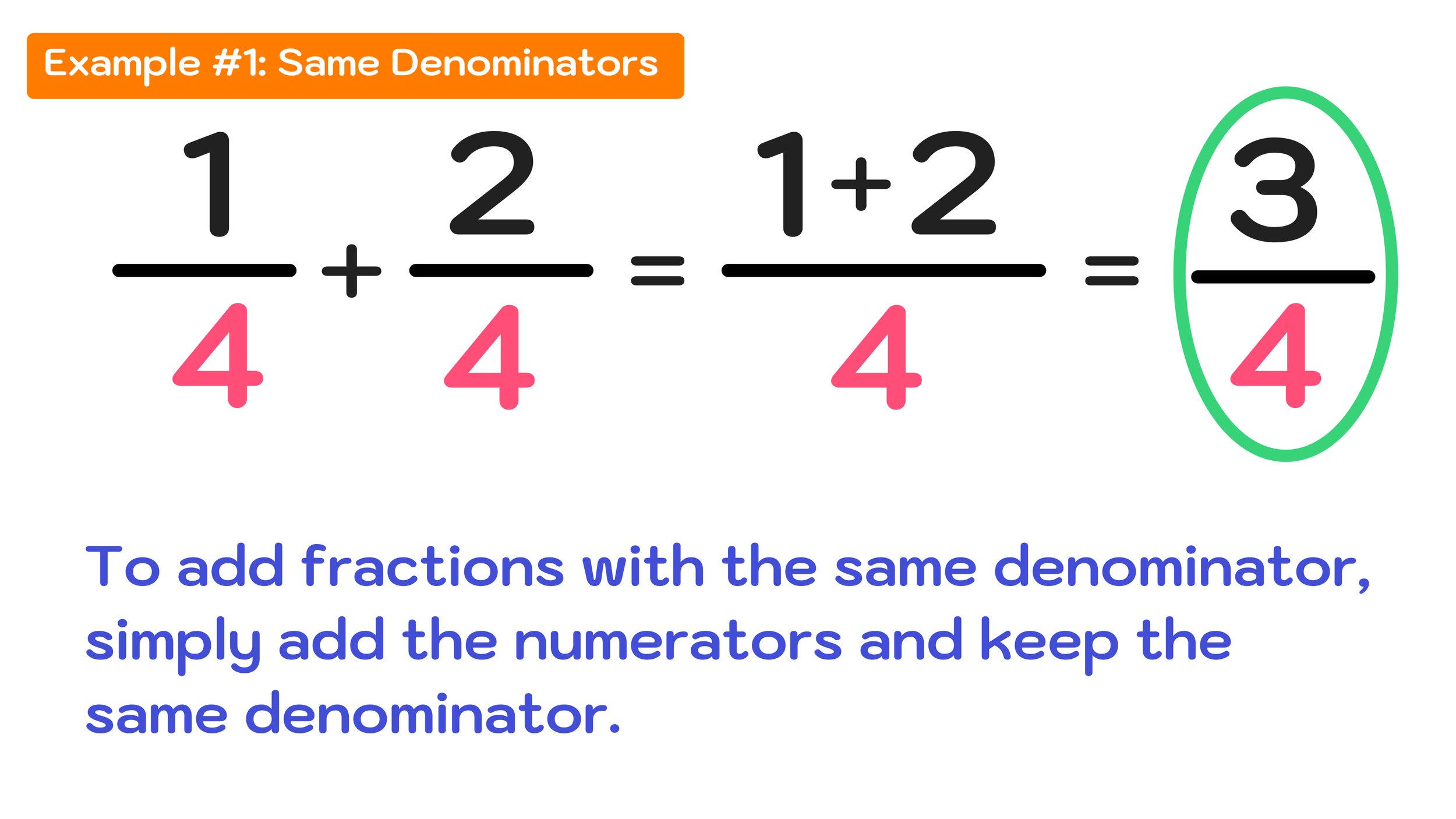
- Check the denominators: If the denominators are the same, compare the numerators. The fraction with the larger numerator is the larger fraction.
- Find the least common multiple (LCM): If the denominators are different, find the least common multiple (LCM) of the two denominators.
- Convert both fractions to have the LCM as the denominator: Once you have the LCM, convert both fractions to have the LCM as the denominator.
- Compare the numerators: Now that both fractions have the same denominator, compare the numerators. The fraction with the larger numerator is the larger fraction.
Example: Comparing Fractions with Different Denominators
Let’s compare the fractions 1⁄2 and 3⁄4.
- Check the denominators: The denominators are different (2 and 4).
- Find the LCM: The LCM of 2 and 4 is 4.
- Convert both fractions to have the LCM as the denominator: Convert 1⁄2 to 2⁄4 and leave 3⁄4 as it is.
- Compare the numerators: Now that both fractions have the same denominator (4), compare the numerators. Since 3 is greater than 2, 3⁄4 is the larger fraction.
Using Worksheets to Practice Comparing Fractions
Worksheets are an excellent way to practice comparing fractions. Here are some tips for using worksheets effectively:
- Start with simple problems: Begin with simple problems that involve comparing fractions with the same denominators.
- Gradually increase the difficulty: As you become more confident, move on to problems that involve comparing fractions with different denominators.
- Use visual aids: Visual aids such as number lines and fraction strips can help you understand the relationships between fractions.
Example Worksheet: Comparing Fractions with the Same Denominator
| Fraction 1 | Fraction 2 | Which fraction is larger? |
|---|---|---|
| 1⁄4 | 2⁄4 | |
| 3⁄6 | 5⁄6 | |
| 2⁄8 | 5⁄8 |
Example Worksheet: Comparing Fractions with Different Denominators
| Fraction 1 | Fraction 2 | Which fraction is larger? |
|---|---|---|
| 1⁄2 | 3⁄4 | |
| 2⁄3 | 3⁄5 | |
| 1⁄4 | 2⁄6 |
📝 Note: You can create your own worksheets using the steps outlined above or use pre-made worksheets from reputable sources.
Conclusion
Comparing fractions can be a challenging task, but with practice and the right tools, it can be made easy. By following the step-by-step approach outlined in this guide and using worksheets to practice, you’ll become a pro at comparing fractions in no time.
What is the difference between a proper fraction and an improper fraction?
+
A proper fraction is a fraction where the numerator is less than the denominator, while an improper fraction is a fraction where the numerator is greater than or equal to the denominator.
How do I find the least common multiple (LCM) of two numbers?
+
The LCM of two numbers can be found by listing the multiples of each number and identifying the smallest multiple that is common to both.
What are some common mistakes to avoid when comparing fractions?
+
Some common mistakes to avoid when comparing fractions include not checking the denominators, not finding the LCM, and not converting both fractions to have the same denominator.