Convert Improper Fractions to Mixed Numbers Easily
Understanding Fractions: Improper and Mixed Numbers
Fractions are a fundamental concept in mathematics, representing a part of a whole. There are three main types of fractions: proper fractions, improper fractions, and mixed numbers. In this blog post, we will focus on converting improper fractions to mixed numbers, a crucial skill in mathematics and real-world applications.
What are Improper Fractions?
An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). For example:
- 5⁄4
- 3⁄2
- 7⁄3
These fractions are called “improper” because they do not represent a part of a whole, but rather a whole or more.
What are Mixed Numbers?
A mixed number is a combination of a whole number and a proper fraction. For example:
- 2 1⁄2
- 3 3⁄4
- 1 1⁄3
Mixed numbers represent a whole number plus a fraction of a whole.
Converting Improper Fractions to Mixed Numbers
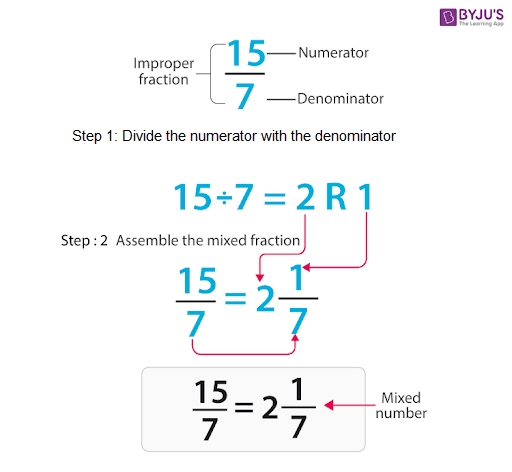
To convert an improper fraction to a mixed number, you need to divide the numerator by the denominator. The quotient (result of division) becomes the whole number, and the remainder becomes the new numerator. The denominator remains the same.
Let’s use the example of 5⁄4:
- Divide the numerator (5) by the denominator (4): 5 ÷ 4 = 1 with a remainder of 1.
- Write the quotient (1) as the whole number.
- Write the remainder (1) as the new numerator.
- Keep the denominator (4) the same.
The result is: 1 1⁄4
Here are a few more examples:
- 3⁄2 = 1 1⁄2
- 7⁄3 = 2 1⁄3
- 9⁄5 = 1 4⁄5
Steps to Convert Improper Fractions to Mixed Numbers
Here are the step-by-step instructions to convert an improper fraction to a mixed number:
- Divide the numerator by the denominator.
- Write the quotient as the whole number.
- Write the remainder as the new numerator.
- Keep the denominator the same.
- Write the result as a mixed number.
Example Problems
Let’s practice converting improper fractions to mixed numbers with some example problems:
- 11⁄6 =?
- 23⁄8 =?
- 17⁄9 =?
Answers:
- 11⁄6 = 1 5⁄6
- 23⁄8 = 2 7⁄8
- 17⁄9 = 1 8⁄9
Table of Conversion Examples
| Improper Fraction | Mixed Number |
|---|---|
| 5/4 | 1 1/4 |
| 3/2 | 1 1/2 |
| 7/3 | 2 1/3 |
| 9/5 | 1 4/5 |
| 11/6 | 1 5/6 |
| 23/8 | 2 7/8 |
| 17/9 | 1 8/9 |
Notes
💡 Note: When converting an improper fraction to a mixed number, make sure to simplify the fraction first, if possible.
Real-World Applications
Converting improper fractions to mixed numbers is a useful skill in various real-world applications, such as:
- Measuring ingredients for cooking and baking
- Calculating distances and speeds in physics and engineering
- Understanding time and schedules in everyday life
In conclusion, converting improper fractions to mixed numbers is a simple process that requires division and rewriting the result in a specific format. By following the steps outlined in this blog post, you can easily convert improper fractions to mixed numbers and apply this skill in various real-world situations.
What is the difference between an improper fraction and a mixed number?
+An improper fraction is a fraction where the numerator is greater than or equal to the denominator, while a mixed number is a combination of a whole number and a proper fraction.
How do I convert an improper fraction to a mixed number?
+To convert an improper fraction to a mixed number, divide the numerator by the denominator, write the quotient as the whole number, and write the remainder as the new numerator.
What is the importance of converting improper fractions to mixed numbers in real-world applications?
+Converting improper fractions to mixed numbers is important in various real-world applications, such as measuring ingredients, calculating distances and speeds, and understanding time and schedules.