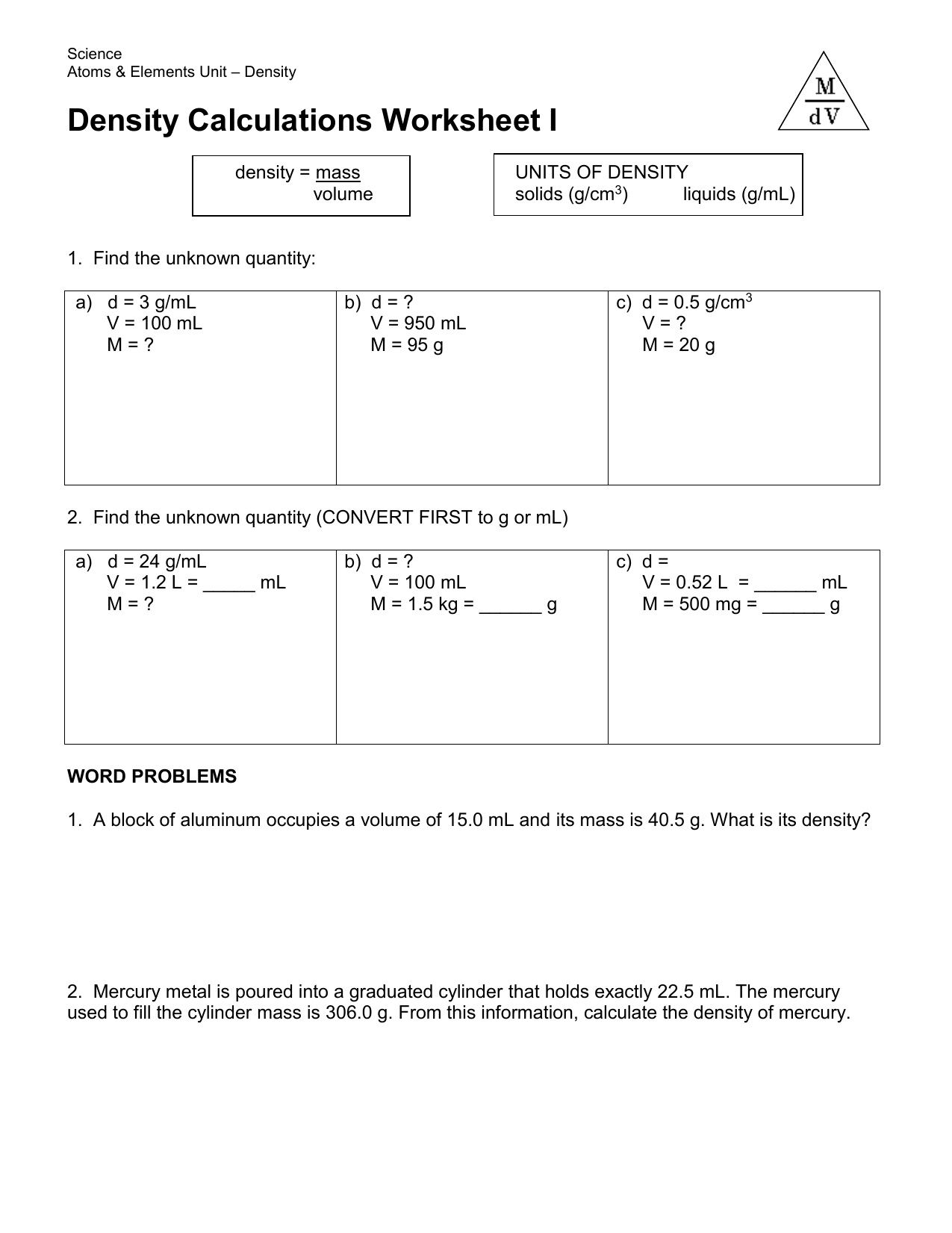
5 Ways to Find Centers of Triangles

Introduction to Triangle Centers

Triangles are one of the most basic shapes in geometry, yet they hold a wealth of interesting properties and characteristics. One of the most fascinating aspects of triangles is their centers. A triangle center is a point that has some special property or relationship with the triangle, such as being equidistant from the vertices or being the intersection of certain lines. In this article, we will explore five ways to find the centers of triangles.

1. Finding the Centroid

The centroid of a triangle is the point of intersection of the medians, which are the lines from each vertex to the midpoint of the opposite side. To find the centroid, you can use the following steps:

• Draw a triangle on a piece of paper.
• Find the midpoint of each side by measuring the length of the side and dividing it by two.
• Draw a line from each vertex to the midpoint of the opposite side.
• The point where the three lines intersect is the centroid.

📝 Note: The centroid divides each median into two segments, one of which is twice the length of the other.

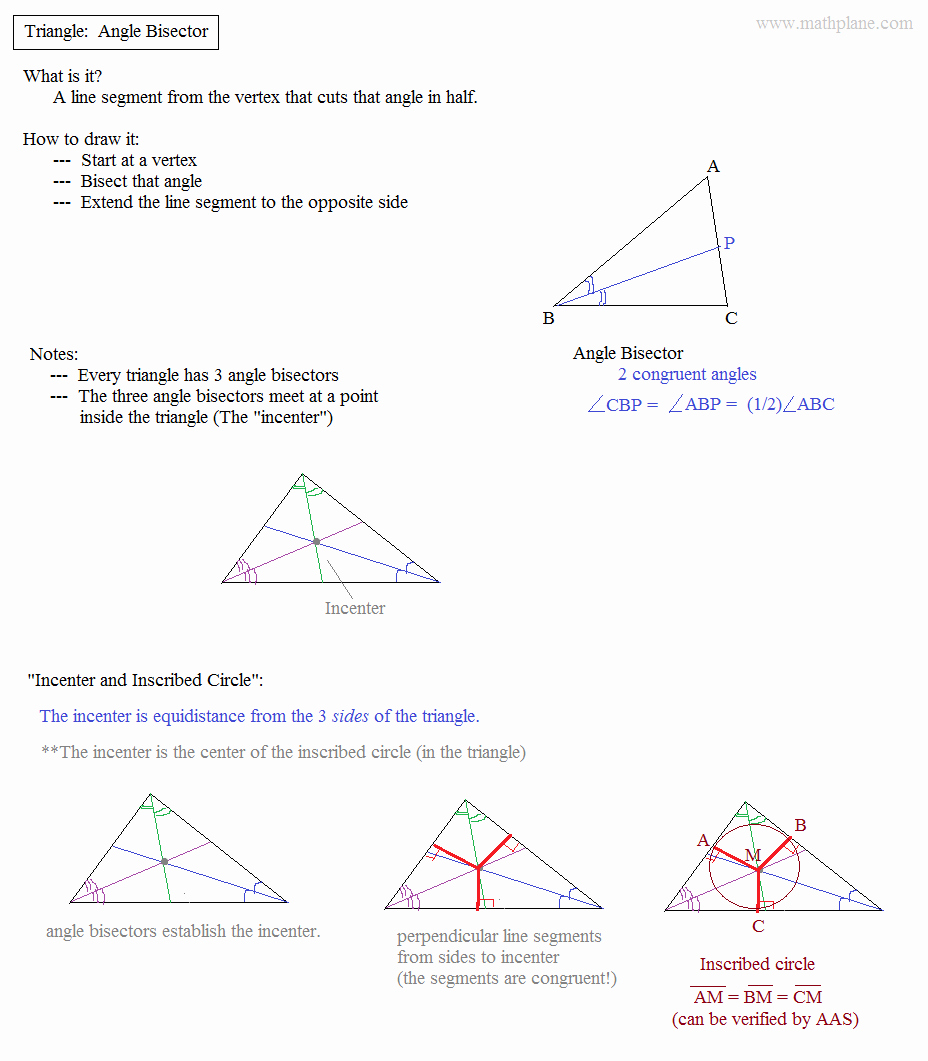
2. Finding the Incenter

The incenter of a triangle is the point of intersection of the angle bisectors, which are the lines that divide each angle into two equal parts. To find the incenter, you can use the following steps:

• Draw a triangle on a piece of paper.
• Find the angle bisectors by drawing a line from each vertex that divides the angle into two equal parts.
• The point where the three lines intersect is the incenter.

📝 Note: The incenter is equidistant from all three sides of the triangle.

3. Finding the Circumcenter

The circumcenter of a triangle is the point of intersection of the perpendicular bisectors, which are the lines that pass through the midpoint of each side and are perpendicular to that side. To find the circumcenter, you can use the following steps:

• Draw a triangle on a piece of paper.
• Find the midpoint of each side by measuring the length of the side and dividing it by two.
• Draw a line from each midpoint that is perpendicular to the side.
• The point where the three lines intersect is the circumcenter.

📝 Note: The circumcenter is equidistant from all three vertices of the triangle.

4. Finding the Orthocenter

The orthocenter of a triangle is the point of intersection of the altitudes, which are the lines that pass through each vertex and are perpendicular to the opposite side. To find the orthocenter, you can use the following steps:

• Draw a triangle on a piece of paper.
• Find the altitude from each vertex by drawing a line that is perpendicular to the opposite side.
• The point where the three lines intersect is the orthocenter.

📝 Note: The orthocenter may lie inside or outside the triangle, depending on the type of triangle.

5. Finding the Nine-Point Center

The nine-point center of a triangle is the point of intersection of the lines that pass through the midpoints of the sides and the feet of the altitudes. To find the nine-point center, you can use the following steps:

• Draw a triangle on a piece of paper.
• Find the midpoint of each side by measuring the length of the side and dividing it by two.
• Draw a line from each midpoint to the foot of the altitude from the opposite vertex.
• The point where the three lines intersect is the nine-point center.

📝 Note: The nine-point center is equidistant from all three vertices and the midpoints of the sides.

Conclusion

In conclusion, finding the centers of triangles is a fascinating topic that can be explored using various methods. By understanding the properties of each center, you can gain a deeper appreciation for the geometry of triangles. Whether you are a student or a professional, learning about triangle centers can help you develop your problem-solving skills and improve your understanding of mathematics.