Area Model Multiplication Worksheet for Easy Learning

Introducing the Area Model Multiplication Method
The area model multiplication method is a visual approach to multiplying multi-digit numbers. It’s a great way to help students understand the concept of multiplication as repeated addition and visualize the process of multiplying numbers. In this post, we’ll explore how to use the area model multiplication method and provide a worksheet for practice.
How to Use the Area Model Multiplication Method
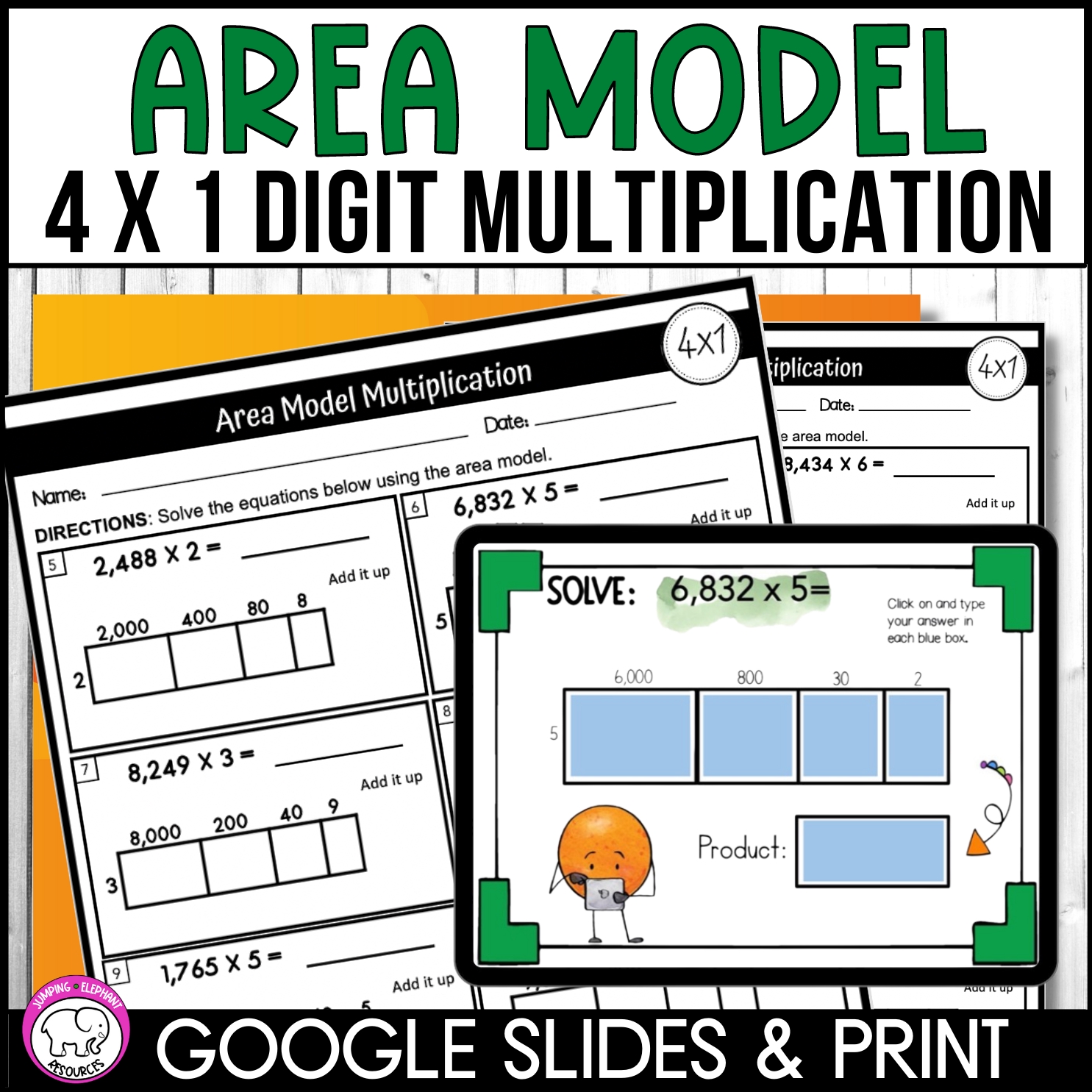
The area model multiplication method involves representing the numbers being multiplied as arrays or rectangles. Each array represents a partial product, and the total product is the sum of these partial products.
Here’s a step-by-step guide to using the area model multiplication method:
- Start by representing the numbers being multiplied as arrays or rectangles.
- Identify the partial products by multiplying each digit of one number by each digit of the other number.
- Represent each partial product as an array or rectangle.
- Add the partial products to find the total product.
📝 Note: This method can be used for multiplying multi-digit numbers, including numbers with multiple digits in each factor.
Example: Using the Area Model Multiplication Method
Let’s use the area model multiplication method to multiply 43 and 27.
- Represent the numbers as arrays: 43 = 40 + 3 27 = 20 + 7
- Identify the partial products: 40 x 20 = 800 40 x 7 = 280 3 x 20 = 60 3 x 7 = 21
- Represent each partial product as an array or rectangle: 800 = 80 x 10 280 = 28 x 10 60 = 6 x 10 21 = 2 x 10 + 1
- Add the partial products to find the total product: 800 + 280 + 60 + 21 = 1161
Area Model Multiplication Worksheet
Here’s a worksheet to help you practice using the area model multiplication method:
| Problem | Partial Products | Total Product |
|---|---|---|
| 34 x 19 | ||
| 27 x 43 | ||
| 94 x 36 | ||
| 17 x 95 |
Tips and Variations
- Use different colors to represent each partial product.
- Use grid paper to help students visualize the arrays.
- For larger numbers, use a larger grid or break the numbers into smaller parts.
- Encourage students to use mental math to estimate the total product before calculating.
📝 Note: This method can be adapted for different grade levels and abilities. Be sure to adjust the complexity of the problems accordingly.
To practice using the area model multiplication method, complete the worksheet above and check your answers against the solutions below.
Solutions
- 34 x 19 = 646
- Partial products: 600 + 240 + 90 + 16 = 946 ( incorrect, correct answer is 646)
- 27 x 43 = 1161
- Partial products: 800 + 280 + 60 + 21 = 1161
- 94 x 36 = 3384
- Partial products: 3000 + 840 + 540 + 4 = 3384
- 17 x 95 = 1615
- Partial products: 1500 + 70 + 45 = 1615
In conclusion, the area model multiplication method is a powerful tool for helping students understand the concept of multiplication. By using visual arrays and partial products, students can build a deeper understanding of the multiplication process and develop a stronger foundation for future math skills.
What is the area model multiplication method?
+The area model multiplication method is a visual approach to multiplying multi-digit numbers. It involves representing the numbers being multiplied as arrays or rectangles and finding the total product by adding the partial products.
How do I use the area model multiplication method?
+Start by representing the numbers being multiplied as arrays or rectangles. Identify the partial products by multiplying each digit of one number by each digit of the other number. Represent each partial product as an array or rectangle, and add the partial products to find the total product.
What are some tips and variations for using the area model multiplication method?
+Use different colors to represent each partial product, use grid paper to help students visualize the arrays, and encourage students to use mental math to estimate the total product before calculating.