Master 5 Essential Arcs and Angles Worksheet Answers

Mastering Essential Arcs and Angles: A Comprehensive Guide

When it comes to geometry and trigonometry, understanding arcs and angles is crucial for problem-solving and critical thinking. In this article, we’ll delve into the world of arcs and angles, exploring five essential concepts that will help you master these fundamental ideas.

What are Arcs and Angles?

Before we dive into the essential arcs and angles, let’s define what they are. An arc is a segment of a curve, typically a part of a circle. Angles, on the other hand, are formed by two rays sharing a common endpoint, called the vertex.

1. Understanding the Unit Circle

The unit circle is a fundamental concept in trigonometry, and it’s essential to understand how arcs and angles relate to it. The unit circle is a circle with a radius of 1, centered at the origin (0,0) of a coordinate plane.

Key Takeaways:

• The unit circle has a circumference of 2π.
• Angles in the unit circle are measured in radians.
• The sine, cosine, and tangent functions are defined using the unit circle.

Example Problem:

Find the length of the arc subtended by a central angle of 60° in a unit circle.

Solution:

Since the central angle is 60°, we can convert it to radians: 60° = π/3 radians.

The length of the arc is given by the formula: s = rθ, where s is the arc length, r is the radius (1 in this case), and θ is the central angle in radians.

s = 1 × π/3 = π/3

Therefore, the length of the arc is π/3.

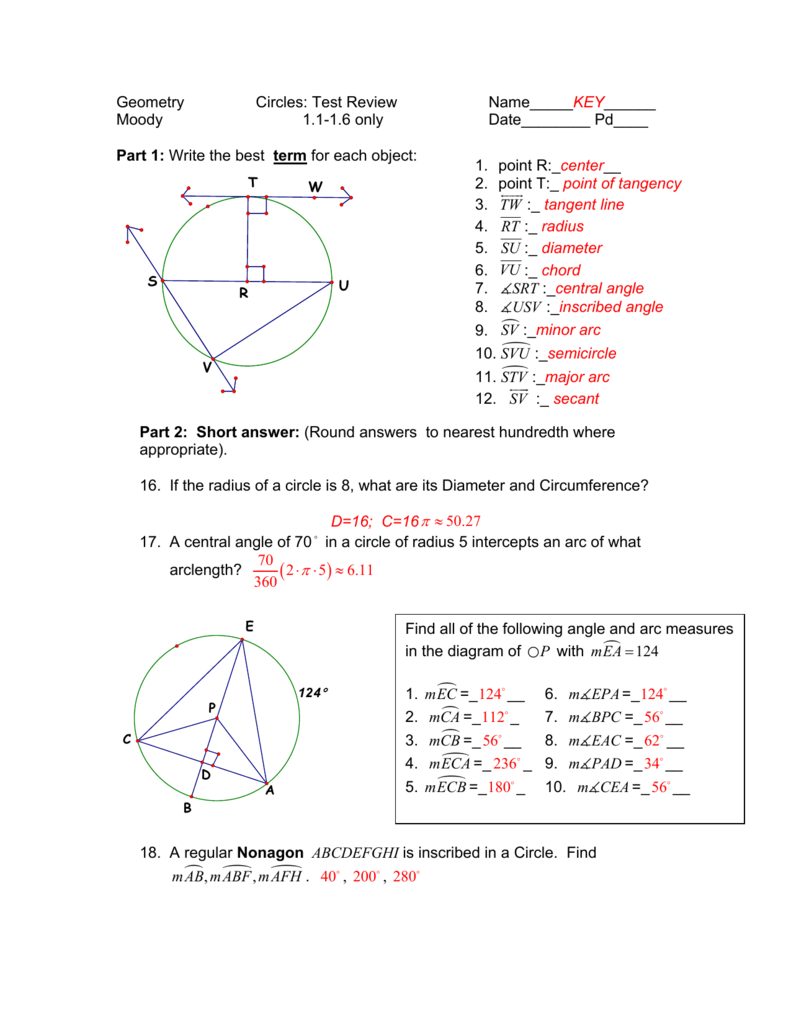
2. Inscribed Angles and Arcs

An inscribed angle is an angle formed by two chords or secants that intersect on a circle. The inscribed angle theorem states that the measure of an inscribed angle is half the measure of its intercepted arc.

Key Takeaways:

• Inscribed angles are half the measure of their intercepted arcs.
• The inscribed angle theorem applies to both acute and obtuse angles.

Example Problem:

In a circle, an inscribed angle intercepts an arc of 120°. Find the measure of the inscribed angle.

Solution:

Using the inscribed angle theorem, we know that the measure of the inscribed angle is half the measure of its intercepted arc:

m∠ = (¹⁄₂) × 120° = 60°

Therefore, the measure of the inscribed angle is 60°.

3. Central Angles and Arcs

A central angle is an angle formed by two radii of a circle. The central angle theorem states that the measure of a central angle is equal to the measure of its intercepted arc.

Key Takeaways:

• Central angles are equal to their intercepted arcs.
• Central angles can be acute, obtuse, or right angles.

Example Problem:

In a circle, a central angle intercepts an arc of 90°. Find the measure of the central angle.

Solution:

Using the central angle theorem, we know that the measure of the central angle is equal to the measure of its intercepted arc:

m∠ = 90°

Therefore, the measure of the central angle is 90°.

4. Arc Length and Circumference

The arc length of a circle is the distance along the circle’s circumference between two points. The circumference of a circle is the distance around the circle.

Key Takeaways:

• Arc length is a fraction of the circumference.
• The formula for arc length is: s = rθ, where s is the arc length, r is the radius, and θ is the central angle in radians.

Example Problem:

Find the arc length of a sector with a central angle of 45° and a radius of 4 cm.

Solution:

First, convert the central angle to radians: 45° = π/4 radians.

Then, use the formula for arc length: s = rθ = 4 × π/4 = π

Therefore, the arc length is π cm.

5. Sectors and Arcs

A sector is a region of a circle bounded by two radii and an arc. Sectors can be used to find the area of a circle.

Key Takeaways:

• Sectors are used to find the area of a circle.
• The formula for the area of a sector is: A = (θ/360) × πr^2, where A is the area, θ is the central angle in degrees, and r is the radius.

Example Problem:

Find the area of a sector with a central angle of 120° and a radius of 6 cm.

Solution:

Use the formula for the area of a sector: A = (θ/360) × πr^2 = (¹²⁰⁄₃₆₀) × π × 6^2 = 12π

Therefore, the area of the sector is 12π cm^2.

📝 Note: Practice problems and exercises are essential to mastering arcs and angles. Make sure to work through plenty of examples to reinforce your understanding of these concepts.

In conclusion, mastering arcs and angles is crucial for success in geometry and trigonometry. By understanding the unit circle, inscribed angles, central angles, arc length and circumference, and sectors, you’ll be well-equipped to tackle complex problems and challenges. Remember to practice regularly and reinforce your understanding with real-world applications.

Related Terms:

• Arcs and Chords worksheet
• Inscribed Angles Worksheet answers