5 Ways to Master Multiplying Fractions Worksheets
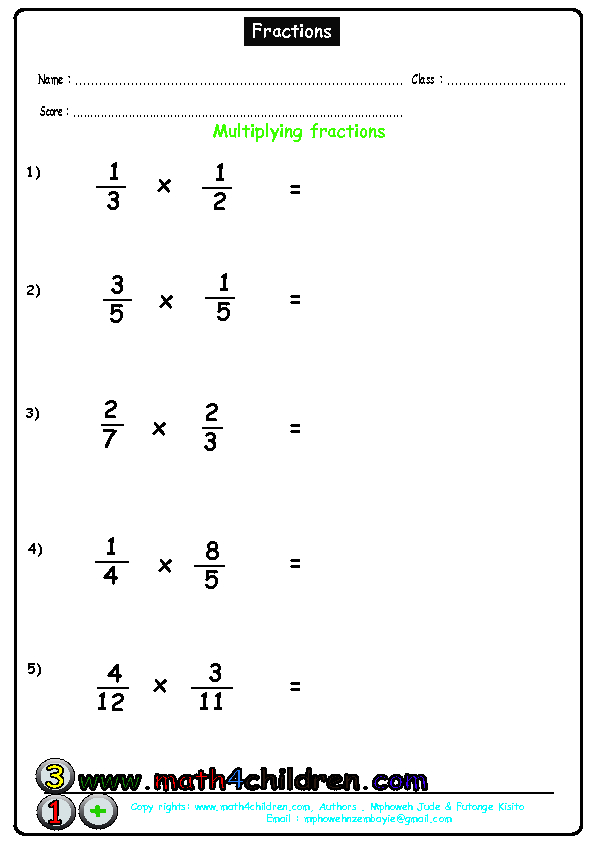
Unlocking the Secrets of Multiplying Fractions Worksheets
Multiplying fractions worksheets can be a daunting task for many students. However, with the right approach and techniques, mastering these worksheets can become a breeze. In this article, we will explore five effective ways to help you conquer multiplying fractions worksheets and become a math whiz.
Understanding the Basics
Before we dive into the five ways to master multiplying fractions worksheets, it’s essential to understand the basics of multiplying fractions. Multiplying fractions involves multiplying the numerators (the numbers on top) and multiplying the denominators (the numbers on the bottom). The formula for multiplying fractions is:
Formula: (a/b) × (c/d) = (ac)/(bd)
Where a, b, c, and d are numbers.
1. Visualize the Problem
One of the most effective ways to master multiplying fractions worksheets is to visualize the problem. Drawing diagrams or pictures can help you understand the concept of multiplying fractions better. For example, if you have to multiply 1⁄2 and 3⁄4, you can draw a diagram to represent the fractions.
Example:
1⁄2 = 1 part out of 2
3⁄4 = 3 parts out of 4
When you multiply these fractions, you are essentially multiplying the number of parts. So, the product would be:
(1 × 3) / (2 × 4) = 3⁄8
2. Use Real-World Examples
Using real-world examples can make multiplying fractions more meaningful and fun. For instance, if you have to multiply 2⁄3 and 3⁄4, you can think of a real-world scenario where you need to multiply these fractions.
Example:
If you have 2⁄3 of a pizza and you want to share it with your friend who has 3⁄4 of a pizza, how much pizza do you have in total?
(2⁄3) × (3⁄4) = (2 × 3) / (3 × 4) = 6⁄12
You have 6⁄12 or 1⁄2 of a pizza in total.
3. Practice with Simple Fractions
Practicing with simple fractions can help build your confidence and fluency in multiplying fractions. Start with simple fractions like 1⁄2, 1⁄3, 2⁄3, etc. and gradually move on to more complex fractions.
Example:
(1⁄2) × (1⁄3) = (1 × 1) / (2 × 3) = 1⁄6
4. Use the "Flipping" Method
The “flipping” method involves flipping the second fraction and changing the multiplication sign to division. This method can be helpful when multiplying fractions with large numbers.
Example:
(2⁄3) × (4⁄5) = (2 × 5) / (3 × 4) = 10⁄12
Using the “flipping” method, you can rewrite the problem as:
(2⁄3) ÷ (5⁄4) = (2 × 4) / (3 × 5) = 8⁄15
5. Play Fraction Games
Playing fraction games can make learning fun and engaging. You can create your own games or use online resources to practice multiplying fractions.
Example:
Fraction War: Create a deck of fraction cards and play a game of war with your friend. The player with the highest product wins the round.
Fraction Bingo: Create bingo cards with fractions and play a game of bingo. When you get a fraction problem, solve it and mark the answer on your card.
📝 Note: Practice regularly to master multiplying fractions worksheets. Start with simple fractions and gradually move on to more complex ones.
In conclusion, mastering multiplying fractions worksheets requires practice, patience, and persistence. By visualizing the problem, using real-world examples, practicing with simple fractions, using the “flipping” method, and playing fraction games, you can become a math whiz and conquer multiplying fractions worksheets with ease.
What is the formula for multiplying fractions?
+The formula for multiplying fractions is (a/b) × (c/d) = (ac)/(bd)
How can I visualize the problem when multiplying fractions?
+You can draw diagrams or pictures to represent the fractions and understand the concept of multiplying fractions better.
What is the “flipping” method in multiplying fractions?
+The “flipping” method involves flipping the second fraction and changing the multiplication sign to division.