5 Ways to Master Triangle Sum Theorem

Unlocking the Secrets of Triangle Sum Theorem

The Triangle Sum Theorem, also known as the Triangle Inequality Theorem, is a fundamental concept in geometry that has far-reaching implications in various fields, including mathematics, physics, and engineering. This theorem states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. In this article, we will delve into the world of triangle sum theorem and explore five ways to master this essential concept.

Understanding the Basics

Before we dive into the advanced techniques, let’s first review the basics of the Triangle Sum Theorem. The theorem can be stated as follows:

Theorem: In any triangle, the sum of the lengths of any two sides is greater than the length of the third side.

Mathematical Representation:

Let’s consider a triangle with sides of lengths a, b, and c. Then, the triangle sum theorem can be represented mathematically as:

a + b > c a + c > b b + c > a

5 Ways to Master Triangle Sum Theorem

Now that we have a solid grasp of the basics, let’s explore five ways to master the Triangle Sum Theorem:

1. Visualizing the Theorem

One of the best ways to understand the Triangle Sum Theorem is to visualize it. Draw a triangle with sides of different lengths and label each side. Then, use a ruler or a measuring tape to measure the lengths of each side. By observing the relationships between the sides, you can gain a deeper understanding of the theorem.

Activity:

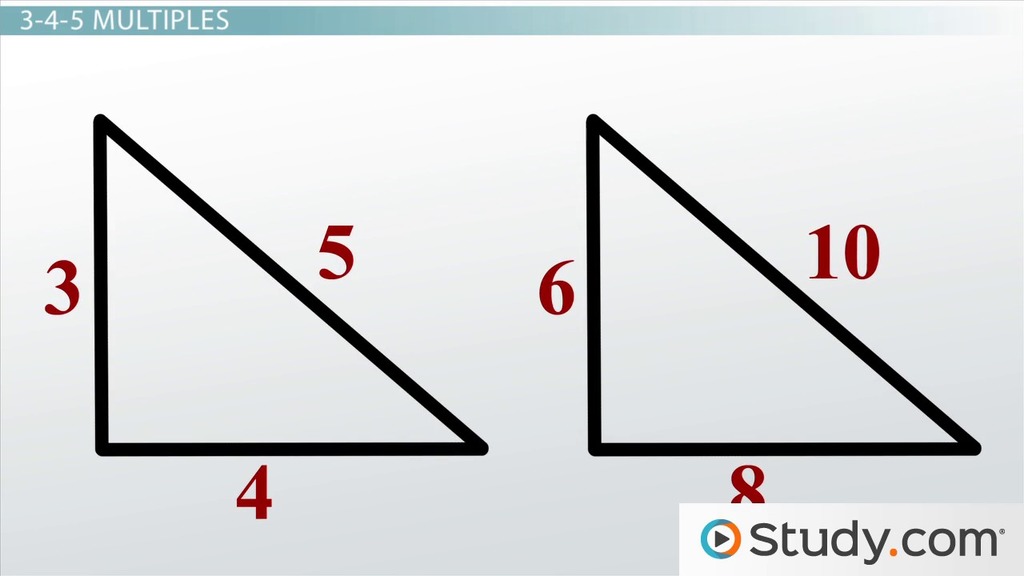
• Draw a triangle with sides of lengths 3 cm, 4 cm, and 5 cm.
• Measure the lengths of each side and verify that the sum of the lengths of any two sides is greater than the length of the third side.

2. Using Algebraic Manipulation

The Triangle Sum Theorem can also be understood using algebraic manipulation. By rearranging the inequalities, we can derive new relationships between the sides of the triangle.

Example:

Let’s consider a triangle with sides of lengths a, b, and c. We can rearrange the inequalities as follows:

a + b > c => a > c - b a + c > b => a > b - c b + c > a => b > a - c

Activity:

• Use algebraic manipulation to derive new relationships between the sides of a triangle.
• Verify that the derived relationships hold true for different triangles.

3. Exploring Real-World Applications

The Triangle Sum Theorem has numerous real-world applications, including physics, engineering, and computer science. By exploring these applications, we can gain a deeper appreciation for the theorem.

Example:

In physics, the Triangle Sum Theorem is used to calculate the range of a projectile. By using the theorem, we can determine the maximum range of a projectile given its initial velocity and angle of projection.

Activity:

• Research real-world applications of the Triangle Sum Theorem.
• Use the theorem to solve problems in physics, engineering, or computer science.

4. Using Geometric Proof

Geometric proof is a powerful tool for understanding the Triangle Sum Theorem. By using geometric proof, we can visualize the relationships between the sides of a triangle.

Example:

Let’s consider a triangle with sides of lengths a, b, and c. We can draw a line segment from point A to point C, parallel to side BC. This creates a new triangle with sides of lengths a, x, and c.

Activity:

• Use geometric proof to demonstrate the Triangle Sum Theorem.
• Verify that the proof holds true for different triangles.

5. Practicing with Examples

Finally, the best way to master the Triangle Sum Theorem is to practice with examples. By solving problems and working through examples, we can develop a deeper understanding of the theorem.

Example:

Let’s consider a triangle with sides of lengths 5 cm, 7 cm, and x cm. Using the Triangle Sum Theorem, we can determine the range of values for x.

Activity:

• Practice solving problems using the Triangle Sum Theorem.
• Work through examples and verify that the theorem holds true for different triangles.

📝 Note: The Triangle Sum Theorem is a fundamental concept in geometry, and mastering it requires practice and patience. By using these five methods, you can develop a deep understanding of the theorem and its applications.

As we have seen, the Triangle Sum Theorem is a powerful tool for understanding the relationships between the sides of a triangle. By mastering this theorem, we can gain a deeper appreciation for geometry and its applications. Whether you’re a student, teacher, or engineer, understanding the Triangle Sum Theorem is essential for success in mathematics and science.

In conclusion, mastering the Triangle Sum Theorem requires a combination of visualization, algebraic manipulation, real-world applications, geometric proof, and practice. By using these five methods, you can develop a deep understanding of the theorem and its applications. Remember to practice regularly and work through examples to reinforce your understanding.

Related Terms:

• Triangle Sum Theorem Worksheet PDF
• Triangle Sum Theorem Kuta Software
• Triangle Sum Theorem Notes PDF