Algebraic Proofs Worksheet Solutions and Study Guide

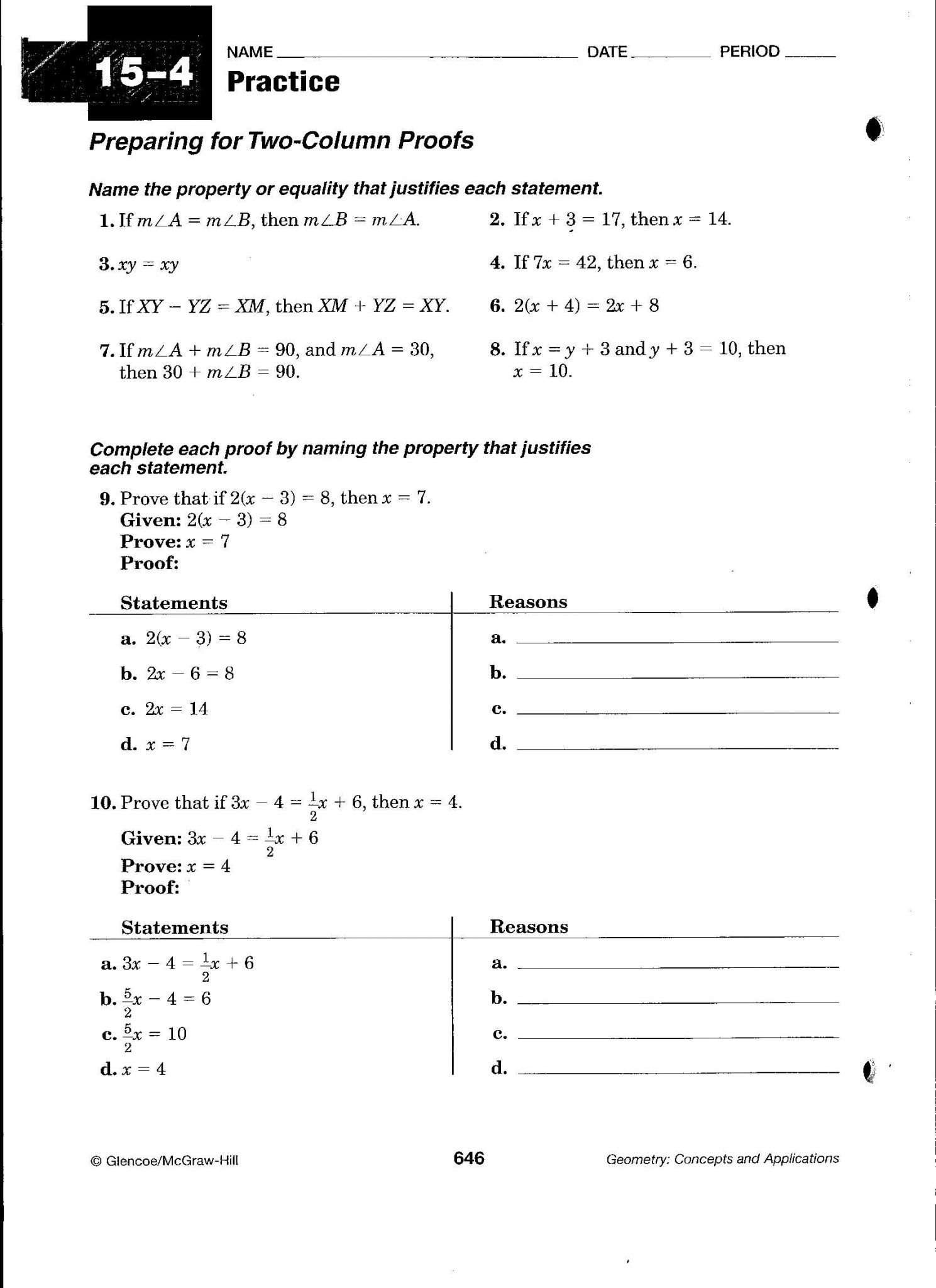
Understanding Algebraic Proofs: A Comprehensive Study Guide

Algebraic proofs are a fundamental concept in mathematics, particularly in algebra and geometry. They involve using logical reasoning and mathematical statements to prove the validity of a given statement or theorem. In this study guide, we will delve into the world of algebraic proofs, exploring the basics, types, and techniques involved. We will also provide solutions to common algebraic proofs worksheet problems.

What is an Algebraic Proof?

An algebraic proof is a step-by-step demonstration of the truth of a mathematical statement, using logical reasoning and algebraic manipulations. It involves starting with a given statement, applying various algebraic operations and rules, and arriving at a conclusion that confirms the original statement. Algebraic proofs are used to establish the validity of mathematical theorems, formulas, and equations.

Types of Algebraic Proofs

There are several types of algebraic proofs, including:

• Direct Proof: A direct proof involves proving a statement directly, using a series of logical steps and algebraic manipulations.
• Indirect Proof: An indirect proof involves proving a statement by assuming the opposite is true and then showing that this assumption leads to a contradiction.
• Proof by Contradiction: A proof by contradiction involves assuming the opposite of the statement is true and then showing that this assumption leads to a logical contradiction.

Techniques for Algebraic Proofs

Several techniques are used in algebraic proofs, including:

• Algebraic Manipulation: Using algebraic operations, such as addition, subtraction, multiplication, and division, to manipulate equations and expressions.
• Substitution: Substituting one expression for another in an equation or expression.
• Cancellation: Cancelling out common factors in an equation or expression.
• Factoring: Factoring expressions into simpler forms.

Solutions to Algebraic Proofs Worksheet Problems

Here are solutions to some common algebraic proofs worksheet problems:

Problem 1: Prove that the sum of the interior angles of a triangle is always 180°.

Solution:

Let’s consider a triangle with angles A, B, and C. We can use the fact that the sum of the interior angles of a triangle is always 180°.

A + B + C = 180°

We can then use algebraic manipulation to rewrite this equation:

A + B = 180° - C

Problem 2: Prove that the equation x^2 + 5x + 6 = 0 has two solutions.

Solution:

We can start by factoring the left-hand side of the equation:

(x + 3)(x + 2) = 0

This tells us that either (x + 3) = 0 or (x + 2) = 0.

Solving for x, we get:

x = -3 or x = -2

Therefore, the equation has two solutions.

Problem 3: Prove that the statement “If x is an odd number, then x^2 is an odd number” is true.

Solution:

Let’s assume that x is an odd number. We can write x as:

x = 2k + 1

where k is an integer.

Squaring both sides, we get:

x^2 = (2k + 1)^2

Expanding the right-hand side, we get:

x^2 = 4k^2 + 4k + 1

This expression is clearly an odd number, since it ends in 1.

Therefore, we have shown that if x is an odd number, then x^2 is also an odd number.

📝 Note: These solutions are just a few examples of how to approach algebraic proofs. Remember to always read the problem carefully and use logical reasoning and algebraic manipulations to arrive at a conclusion.

Conclusion

In conclusion, algebraic proofs are an essential part of mathematics, particularly in algebra and geometry. By understanding the basics, types, and techniques involved, you can become proficient in solving algebraic proofs worksheet problems. Remember to always read the problem carefully, use logical reasoning and algebraic manipulations, and arrive at a conclusion that confirms the original statement.