Algebra Proofs Worksheet Answer Key Solutions Guide
Mastering Algebra Proofs: A Comprehensive Guide
Algebra proofs are a fundamental concept in mathematics, and mastering them is essential for any student looking to excel in algebra and beyond. In this guide, we will walk you through the process of solving algebra proofs, providing you with a step-by-step approach to understanding and tackling these types of problems.
What are Algebra Proofs?
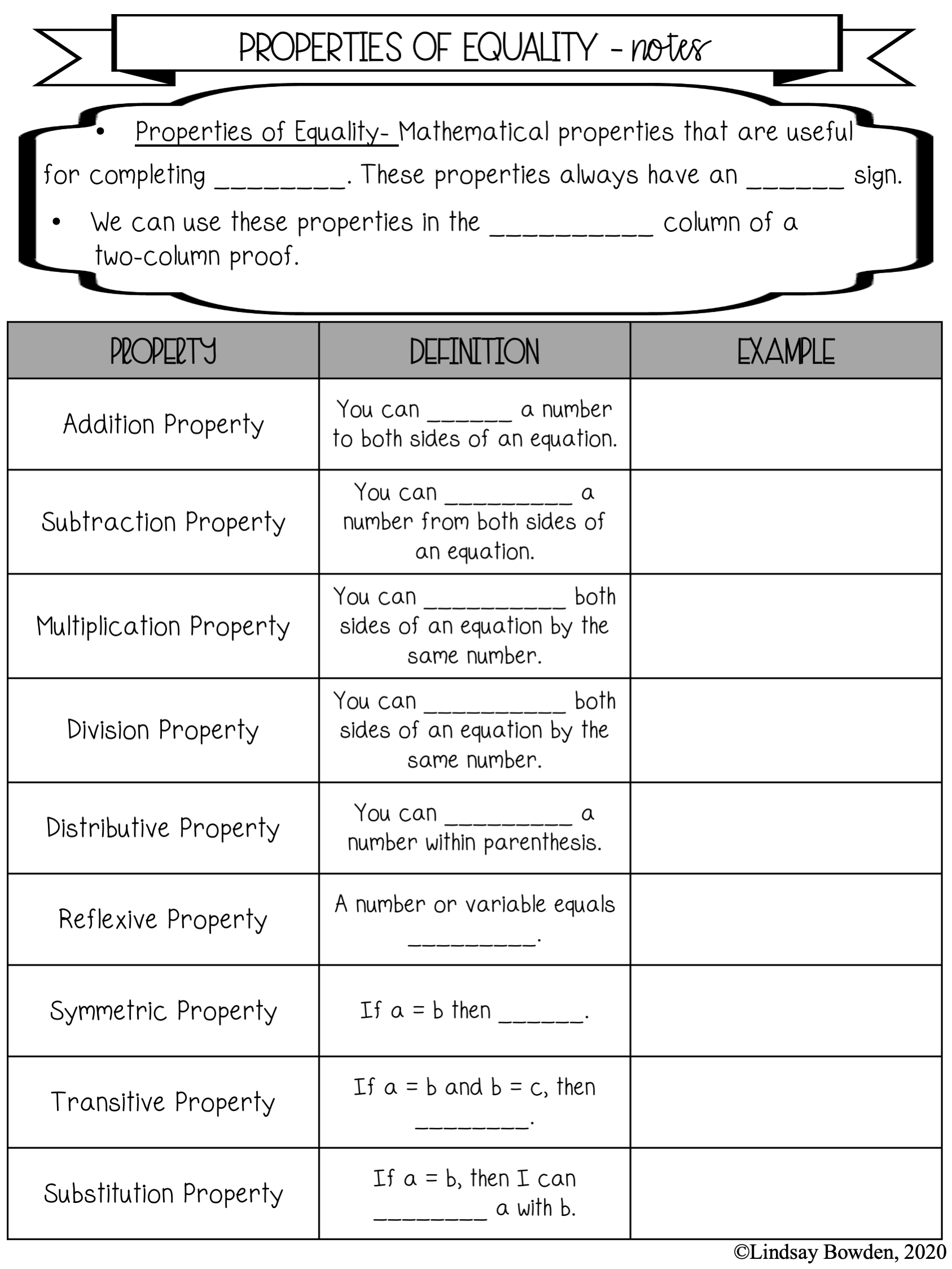
Algebra proofs are a series of logical statements used to prove the validity of an algebraic statement. They involve a series of steps, each of which is justified by a specific property or rule of algebra. Algebra proofs are used to establish the truth of a statement, and they are an essential tool for mathematicians and scientists alike.
Types of Algebra Proofs
There are several types of algebra proofs, including:
- Direct Proofs: These involve a series of logical statements that lead directly to the conclusion.
- Indirect Proofs: These involve assuming the opposite of what you want to prove and then showing that this assumption leads to a logical contradiction.
- Proofs by Contradiction: These involve assuming the opposite of what you want to prove and then showing that this assumption leads to a logical contradiction.
How to Solve Algebra Proofs
Solving algebra proofs involves a series of steps, each of which is designed to help you understand and tackle the problem at hand. Here are the steps you can follow:
- Read the Problem Carefully: Take the time to read the problem carefully and understand what is being asked.
- Identify the Type of Proof: Determine what type of proof you are dealing with (direct, indirect, or proof by contradiction).
- Plan Your Approach: Take a moment to plan your approach and think about how you will tackle the problem.
- Write Your Proof: Write your proof, step by step, using logical statements and justifications for each step.
- Check Your Work: Check your work to ensure that it is accurate and complete.
Example of an Algebra Proof
Here is an example of an algebra proof:
Problem: Prove that for all real numbers x, if x > 0, then x^2 > 0.
Solution:
- Let x be a real number such that x > 0.
- Multiply both sides of the inequality by x to get x^2 > 0.
- Therefore, for all real numbers x, if x > 0, then x^2 > 0.
Important Notes
📝 Note: When writing an algebra proof, it is essential to use logical statements and justifications for each step.
📝 Note: Make sure to check your work to ensure that it is accurate and complete.
Algebra Proofs Worksheet Answer Key Solutions Guide
Here is a sample algebra proofs worksheet with answer key:

| Problem | Solution |
|---|---|
| Prove that for all real numbers x, if x > 0, then x^2 > 0. | 1. Let x be a real number such that x > 0. 2. Multiply both sides of the inequality by x to get x^2 > 0. 3. Therefore, for all real numbers x, if x > 0, then x^2 > 0. |
| Prove that for all real numbers x and y, if x + y = 0, then x = -y. | 1. Let x and y be real numbers such that x + y = 0. 2. Subtract x from both sides of the equation to get y = -x. 3. Therefore, for all real numbers x and y, if x + y = 0, then x = -y. |
Frequently Asked Questions
What is an algebra proof?
+An algebra proof is a series of logical statements used to prove the validity of an algebraic statement.
What are the different types of algebra proofs?
+There are three main types of algebra proofs: direct proofs, indirect proofs, and proofs by contradiction.
How do I write an algebra proof?
+To write an algebra proof, start by reading the problem carefully, identifying the type of proof, planning your approach, writing your proof, and checking your work.
In conclusion, algebra proofs are a fundamental concept in mathematics, and mastering them is essential for any student looking to excel in algebra and beyond. By following the steps outlined in this guide, you can learn to solve algebra proofs with confidence and accuracy.
Related Terms:
- Algebraic Proofs Worksheet PDF
- Algebraic proofs Reasons Worksheet
- Algebraic proofs list
- Segment Proofs Worksheet with answers