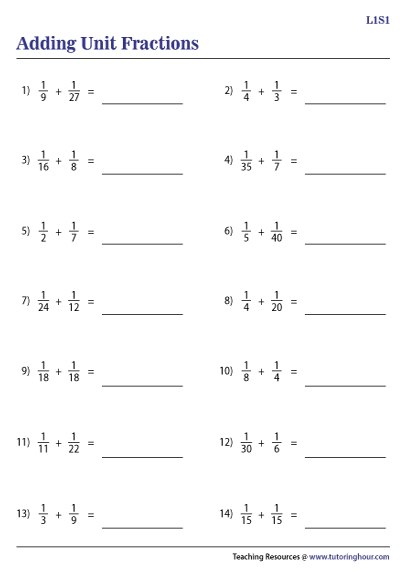
Adding Fractions With Unlike Denominators Made Easy
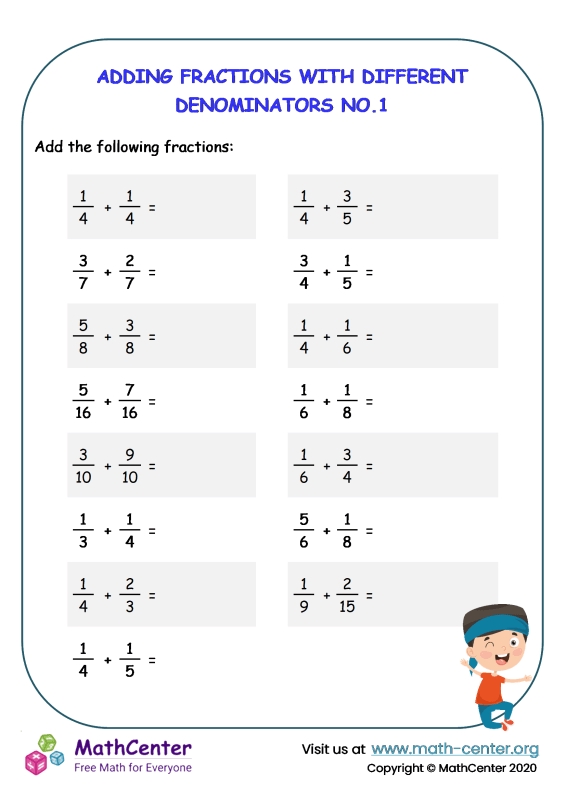
Mastering the Art of Adding Fractions With Unlike Denominators
Adding fractions with unlike denominators can be a daunting task for many students. However, with the right approach and techniques, it can be made easy and straightforward. In this article, we will explore the steps involved in adding fractions with unlike denominators, provide examples and explanations, and offer tips and tricks to make the process easier.
Understanding the Concept of Unlike Denominators
Before we dive into the steps of adding fractions with unlike denominators, it’s essential to understand what unlike denominators mean. Unlike denominators refer to fractions that have different denominators. For example, 1⁄4 and 1⁄6 are fractions with unlike denominators because they have different denominators (4 and 6).
The Steps Involved in Adding Fractions With Unlike Denominators
To add fractions with unlike denominators, follow these steps:
- Find the Least Common Multiple (LCM): The first step is to find the least common multiple (LCM) of the two denominators. The LCM is the smallest number that both denominators can divide into evenly. For example, the LCM of 4 and 6 is 12.
- Convert Both Fractions to Have the LCM as the Denominator: Once you have found the LCM, convert both fractions to have the LCM as the denominator. To do this, multiply the numerator and denominator of each fraction by the necessary multiples. For example, to convert 1⁄4 to have a denominator of 12, multiply the numerator and denominator by 3 (1 x 3 / 4 x 3 = 3⁄12).
- Add the Fractions: Now that both fractions have the same denominator (the LCM), you can add them together. Simply add the numerators (the numbers on top) and keep the denominator the same.
- Simplify the Answer: Finally, simplify the answer by dividing both the numerator and denominator by their greatest common divisor (GCD).
📝 Note: The GCD is the largest number that divides both the numerator and denominator evenly.
Examples and Explanations
Let’s use the example of adding 1⁄4 and 1⁄6 to illustrate the steps:
- Find the LCM: The LCM of 4 and 6 is 12.
- Convert Both Fractions to Have the LCM as the Denominator: Convert 1⁄4 to have a denominator of 12 by multiplying the numerator and denominator by 3 (1 x 3 / 4 x 3 = 3⁄12). Convert 1⁄6 to have a denominator of 12 by multiplying the numerator and denominator by 2 (1 x 2 / 6 x 2 = 2⁄12).
- Add the Fractions: Add the fractions 3⁄12 and 2⁄12 by adding the numerators (3 + 2 = 5) and keeping the denominator the same (12).
- Simplify the Answer: Simplify the answer by dividing both the numerator and denominator by their GCD (5 and 12). The GCD of 5 and 12 is 1, so the answer remains 5⁄12.
| Step | Example |
|---|---|
| Find the LCM | LCM of 4 and 6 = 12 |
| Convert Both Fractions to Have the LCM as the Denominator | 1/4 = 3/12, 1/6 = 2/12 |
| Add the Fractions | 3/12 + 2/12 = 5/12 |
| Simplify the Answer | 5/12 (no simplification needed) |
Tips and Tricks
Here are some tips and tricks to make adding fractions with unlike denominators easier:
- Use a chart or table to find the LCM: If you’re having trouble finding the LCM, use a chart or table to help you.
- Use a common denominator: If the denominators are close together, you can use a common denominator instead of the LCM.
- Simplify as you go: Simplify the fractions as you go to avoid complicated calculations.
- Use visual aids: Use visual aids such as diagrams or blocks to help you understand the concept of adding fractions with unlike denominators.
To summarize, adding fractions with unlike denominators involves finding the LCM, converting both fractions to have the LCM as the denominator, adding the fractions, and simplifying the answer. By following these steps and using the tips and tricks provided, you’ll be able to master the art of adding fractions with unlike denominators.
What is the least common multiple (LCM)?
+The least common multiple (LCM) is the smallest number that both denominators can divide into evenly.
How do I find the LCM of two numbers?
+To find the LCM of two numbers, list the multiples of each number and find the smallest multiple that they have in common.
What is the greatest common divisor (GCD)?
+The greatest common divisor (GCD) is the largest number that divides both the numerator and denominator evenly.