7 Ways to Add Fractions With Different Denominators
Understanding the Concept of Fractions
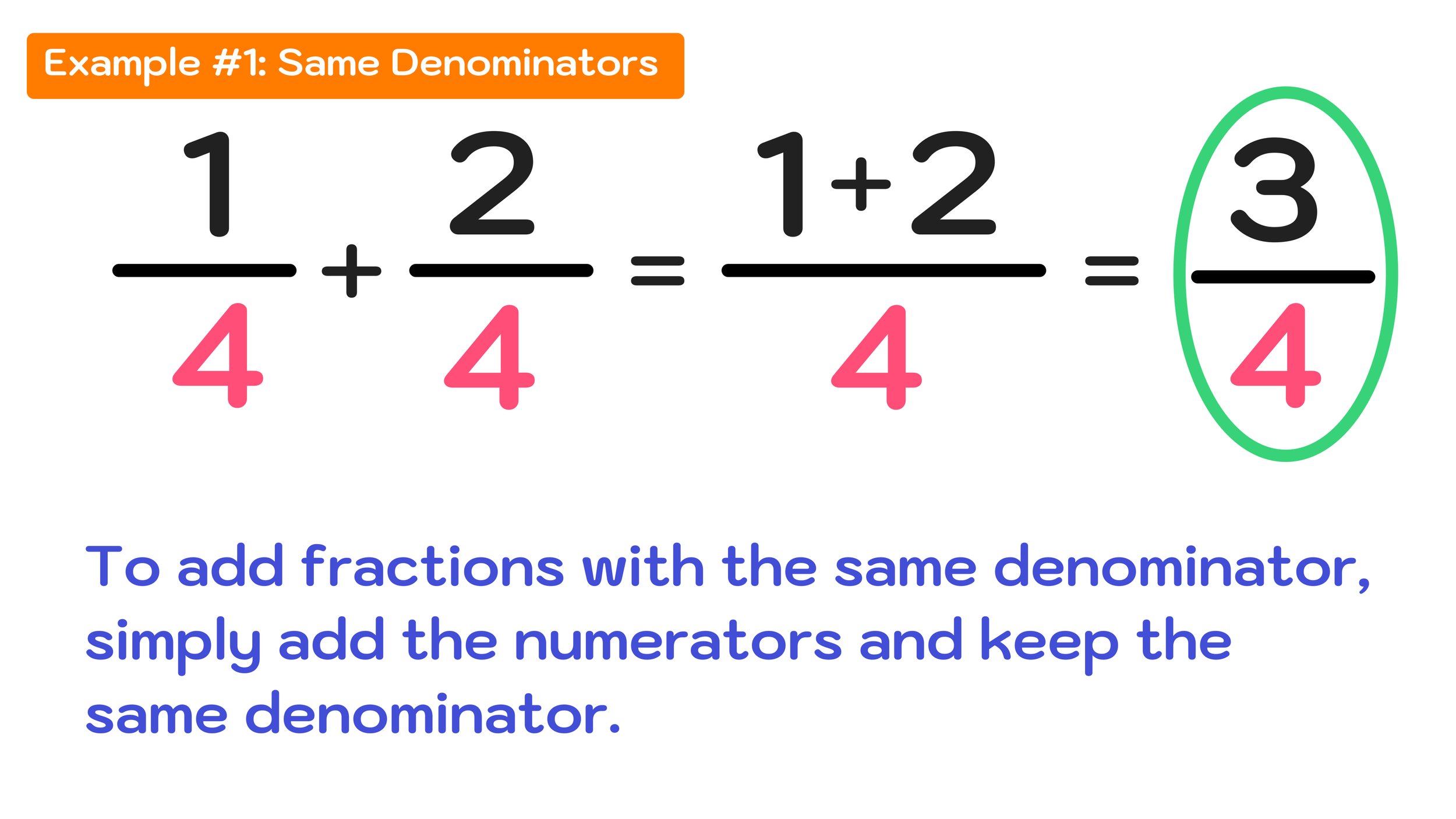
Fractions are a fundamental concept in mathematics, representing a part of a whole. They consist of two parts: the numerator and the denominator. The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into. For instance, in the fraction 3⁄4, the numerator is 3, and the denominator is 4, meaning we have 3 parts out of a total of 4 equal parts.
Adding Fractions with Different Denominators
When adding fractions with different denominators, we need to find a common denominator first. This is because we cannot add fractions with different denominators directly. Here are 7 ways to add fractions with different denominators:
1. Finding the Least Common Multiple (LCM)
One way to add fractions with different denominators is to find the least common multiple (LCM) of the two denominators. The LCM is the smallest number that both denominators can divide into evenly.
For example, let’s say we want to add 1⁄4 and 1⁄6. To find the LCM, we can list the multiples of each denominator:
Multiples of 4: 4, 8, 12, 16, 20, 24,… Multiples of 6: 6, 12, 18, 24, 30,…
The smallest number that appears in both lists is 12, so the LCM is 12. We can then rewrite each fraction with a denominator of 12:
1⁄4 = 3⁄12 1⁄6 = 2⁄12
Now we can add the fractions:
3⁄12 + 2⁄12 = 5⁄12
📝 Note: Finding the LCM can be time-consuming, especially for larger numbers. There are other methods to find the common denominator, which we will discuss below.
2. Using the Greatest Common Divisor (GCD)
Another way to add fractions with different denominators is to use the greatest common divisor (GCD) of the two denominators. The GCD is the largest number that divides both denominators evenly.
For example, let’s say we want to add 2⁄8 and 3⁄12. To find the GCD, we can list the factors of each denominator:
Factors of 8: 1, 2, 4, 8 Factors of 12: 1, 2, 3, 4, 6, 12
The largest number that appears in both lists is 4, so the GCD is 4. We can then divide each denominator by the GCD to find the common denominator:
8 ÷ 4 = 2 12 ÷ 4 = 3
The common denominator is 2 x 3 = 6. We can then rewrite each fraction with a denominator of 6:
2⁄8 = 1⁄4 = 3⁄12 3⁄12 = 2⁄8 = 3⁄12
Now we can add the fractions:
3⁄12 + 3⁄12 = 6⁄12 = 1⁄2
3. Using a Common Denominator Chart
A common denominator chart is a table that lists the multiples of each denominator. We can use this chart to find the common denominator quickly.
For example, let’s say we want to add 3⁄8 and 2⁄12. We can look up the multiples of each denominator in the chart:
| Denominator | Multiples |
|---|---|
| 8 | 8, 16, 24, 32,… |
| 12 | 12, 24, 36, 48,… |
The smallest number that appears in both lists is 24, so the common denominator is 24. We can then rewrite each fraction with a denominator of 24:
3⁄8 = 9⁄24 2⁄12 = 4⁄24
Now we can add the fractions:
9⁄24 + 4⁄24 = 13⁄24
4. Using a Fraction Calculator
There are many online fraction calculators available that can help us add fractions with different denominators quickly. These calculators can find the common denominator and add the fractions for us.
For example, let’s say we want to add 2⁄3 and 3⁄4. We can enter the fractions into the calculator, and it will give us the result:
2⁄3 + 3⁄4 = 17⁄12
5. Converting to Decimals
Another way to add fractions with different denominators is to convert them to decimals first. We can then add the decimals and convert the result back to a fraction.
For example, let’s say we want to add 1⁄2 and 1⁄4. We can convert each fraction to a decimal:
1⁄2 = 0.5 1⁄4 = 0.25
Now we can add the decimals:
0.5 + 0.25 = 0.75
We can then convert the result back to a fraction:
0.75 = 3⁄4
6. Using Visual Aids
Visual aids such as number lines, fraction strips, or circles can help us add fractions with different denominators. These aids can help us visualize the fractions and find the common denominator.
For example, let’s say we want to add 2⁄3 and 3⁄4. We can draw a number line with both fractions marked:
We can see that the common denominator is 12. We can then rewrite each fraction with a denominator of 12:
2⁄3 = 8⁄12 3⁄4 = 9⁄12
Now we can add the fractions:
8⁄12 + 9⁄12 = 17⁄12
7. Using Real-World Examples
Finally, we can use real-world examples to help us add fractions with different denominators. For example, let’s say we have 1⁄4 of a pizza and our friend has 1⁄6 of a pizza. We can find the common denominator by listing the multiples of each denominator:
Multiples of 4: 4, 8, 12, 16,… Multiples of 6: 6, 12, 18, 24,…
The smallest number that appears in both lists is 12, so the common denominator is 12. We can then rewrite each fraction with a denominator of 12:
1⁄4 = 3⁄12 1⁄6 = 2⁄12
Now we can add the fractions:
3⁄12 + 2⁄12 = 5⁄12
In conclusion, there are many ways to add fractions with different denominators. We can use methods such as finding the LCM, using the GCD, creating a common denominator chart, using a fraction calculator, converting to decimals, using visual aids, or using real-world examples. By mastering these methods, we can become more confident and proficient in our ability to add fractions with different denominators.
What is the least common multiple (LCM) of two numbers?
+
The least common multiple (LCM) of two numbers is the smallest number that both numbers can divide into evenly.
How can I add fractions with different denominators using visual aids?
+
Visual aids such as number lines, fraction strips, or circles can help you visualize the fractions and find the common denominator.
What is the greatest common divisor (GCD) of two numbers?
+
The greatest common divisor (GCD) of two numbers is the largest number that divides both numbers evenly.