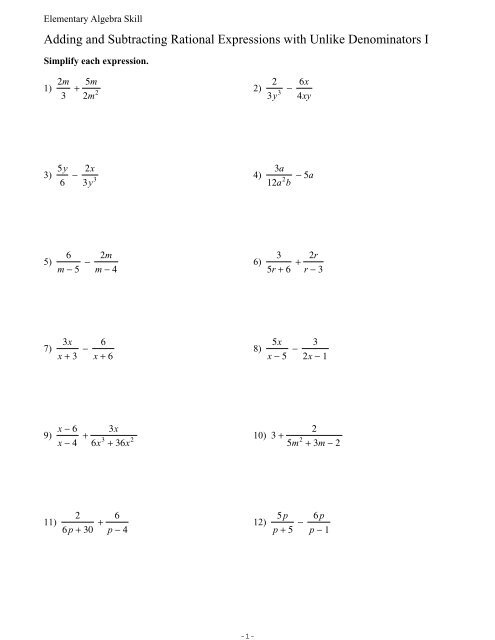
Rational Expressions Simplified: Adding and Subtracting Made Easy

Understanding Rational Expressions

Rational expressions are a fundamental concept in algebra, and they can seem intimidating at first, but with practice and patience, you can master them. A rational expression is a fraction that contains one or more variables in the numerator, denominator, or both. It is essential to simplify rational expressions before adding or subtracting them. Simplifying rational expressions involves factoring the numerator and denominator and canceling out any common factors.

Factors and Greatest Common Factors (GCFs)

Before we dive into adding and subtracting rational expressions, let’s review the concept of factors and greatest common factors (GCFs). A factor is a number or expression that divides another number or expression exactly without leaving a remainder. The GCF is the largest factor that two or more numbers or expressions have in common. To simplify rational expressions, we need to find the GCF of the numerator and denominator.

🤔 Note: To find the GCF, list all the factors of each number or expression and find the largest common factor.

Addition and Subtraction of Rational Expressions

Now that we have reviewed factors and GCFs, let’s move on to adding and subtracting rational expressions. To add or subtract rational expressions, we need to follow these steps:

1. Simplify each rational expression: Factor the numerator and denominator of each rational expression and cancel out any common factors.
2. Find the least common denominator (LCD): The LCD is the smallest multiple of the denominators that all the denominators can divide into evenly. To find the LCD, list the multiples of each denominator and find the smallest multiple that they all have in common.
3. Write each rational expression with the LCD: Multiply the numerator and denominator of each rational expression by the necessary factors to get the LCD.
4. Add or subtract the rational expressions: Once the rational expressions have the same denominator, we can add or subtract them by adding or subtracting the numerators.

Examples of Adding and Subtracting Rational Expressions

Let’s consider a few examples to illustrate the process of adding and subtracting rational expressions.

Example 1: Adding Rational Expressions

Simplify and add:

\[\frac{2x}{x+3} + \frac{x}{x-2}\]

Step 1: Simplify each rational expression

Factor the numerator and denominator of each rational expression and cancel out any common factors.

\[\frac{2x}{x+3} = \frac{2x}{x+3}\]

\[\frac{x}{x-2} = \frac{x}{x-2}\]

Step 2: Find the LCD

List the multiples of each denominator and find the smallest multiple that they all have in common.

The LCD is (x+3)(x-2).

Step 3: Write each rational expression with the LCD

Multiply the numerator and denominator of each rational expression by the necessary factors to get the LCD.

\[\frac{2x}{x+3} = \frac{2x(x-2)}{(x+3)(x-2)}\]

\[\frac{x}{x-2} = \frac{x(x+3)}{(x+3)(x-2)}\]

Step 4: Add the rational expressions

Add the rational expressions by adding the numerators.

\[\frac{2x(x-2)}{(x+3)(x-2)} + \frac{x(x+3)}{(x+3)(x-2)} = \frac{2x^2 - 4x + x^2 + 3x}{(x+3)(x-2)} = \frac{3x^2 - x}{(x+3)(x-2)}\]

Example 2: Subtracting Rational Expressions

Simplify and subtract:

\[\frac{x+1}{x-2} - \frac{x-1}{x+3}\]

Step 1: Simplify each rational expression

Factor the numerator and denominator of each rational expression and cancel out any common factors.

\[\frac{x+1}{x-2} = \frac{x+1}{x-2}\]

\[\frac{x-1}{x+3} = \frac{x-1}{x+3}\]

Step 2: Find the LCD

List the multiples of each denominator and find the smallest multiple that they all have in common.

The LCD is (x-2)(x+3).

Step 3: Write each rational expression with the LCD

Multiply the numerator and denominator of each rational expression by the necessary factors to get the LCD.

\[\frac{x+1}{x-2} = \frac{(x+1)(x+3)}{(x-2)(x+3)}\]

\[\frac{x-1}{x+3} = \frac{(x-1)(x-2)}{(x+3)(x-2)}\]

Step 4: Subtract the rational expressions

Subtract the rational expressions by subtracting the numerators.

\[\frac{(x+1)(x+3)}{(x-2)(x+3)} - \frac{(x-1)(x-2)}{(x+3)(x-2)} = \frac{x^2 + 4x + 3 - (x^2 - 3x + 2)}{(x-2)(x+3)} = \frac{7x + 1}{(x-2)(x+3)}\]

Conclusion

Adding and subtracting rational expressions can seem daunting at first, but by breaking down the process into manageable steps, we can simplify and combine rational expressions with ease. Remember to simplify each rational expression, find the least common denominator, write each rational expression with the LCD, and add or subtract the rational expressions. With practice and patience, you can master the art of adding and subtracting rational expressions.