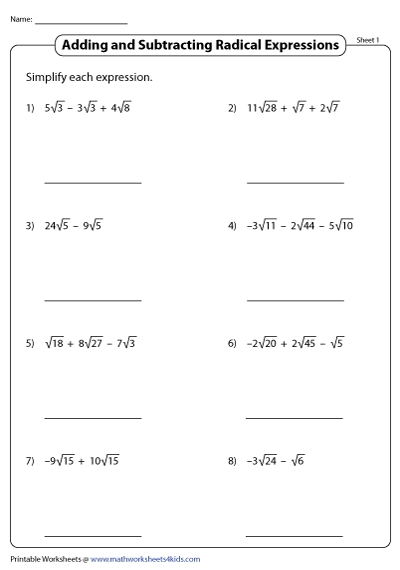
Radicals Made Easy: Adding and Subtracting Simplified

Understanding Radicals

Radicals, also known as roots, are a fundamental concept in mathematics, representing a way to express numbers in terms of their roots. The most common type of radical is the square root, which is used to find the number that, when multiplied by itself, gives a specified value. However, radicals can also be used to represent cube roots, fourth roots, and so on.

What are Simplified Radicals?

Simplified radicals are radical expressions that have been reduced to their simplest form. This means that the number inside the radical sign (the radicand) has been factored to remove any perfect squares, cubes, or other powers of the radical. Simplifying radicals is essential for working with radical expressions, as it makes them easier to add, subtract, multiply, and divide.

Why Add and Subtract Simplified Radicals?

Adding and subtracting simplified radicals is a crucial skill in mathematics, particularly in algebra and calculus. When working with radical expressions, it’s often necessary to combine them, which involves adding or subtracting radicals. Simplified radicals make this process much easier, as they allow you to combine like terms and simplify the resulting expression.

How to Add Simplified Radicals

Adding simplified radicals involves combining radical expressions with the same index (the number outside the radical sign) and the same radicand (the number inside the radical sign). Here are the steps to follow:

• Step 1: Check if the radicals have the same index and radicand. If they do, you can add them.
• Step 2: Add the coefficients (the numbers in front of the radicals) and keep the radical sign and radicand the same.
• Step 3: Simplify the resulting expression by factoring out any perfect squares or other powers of the radical.

Example:

Add 2√3 and 5√3.

• Step 1: Both radicals have the same index (2) and radicand (√3), so you can add them.
• Step 2: Add the coefficients (2 and 5) to get 7√3.
• Step 3: The resulting expression is already simplified, so the final answer is 7√3.

How to Subtract Simplified Radicals

Subtracting simplified radicals involves combining radical expressions with the same index and radicand, but with opposite signs. Here are the steps to follow:

• Step 1: Check if the radicals have the same index and radicand. If they do, you can subtract them.
• Step 2: Subtract the coefficients (the numbers in front of the radicals) and keep the radical sign and radicand the same.
• Step 3: Simplify the resulting expression by factoring out any perfect squares or other powers of the radical.

Example:

Subtract 3√5 from 2√5.

• Step 1: Both radicals have the same index (2) and radicand (√5), so you can subtract them.
• Step 2: Subtract the coefficients (2 and 3) to get -1√5.
• Step 3: The resulting expression is already simplified, so the final answer is -√5.

🤔 Note: When subtracting radicals, make sure to keep the negative sign outside the radical sign.

Common Mistakes to Avoid

When adding and subtracting simplified radicals, there are some common mistakes to avoid:

• Mistake 1: Adding or subtracting radicals with different indexes or radicands.
• Mistake 2: Forgetting to simplify the resulting expression.
• Mistake 3: Not keeping the negative sign outside the radical sign when subtracting radicals.

By following the steps outlined above and avoiding common mistakes, you’ll become proficient in adding and subtracting simplified radicals.

Radicals are an essential part of mathematics, and being able to add and subtract them is a fundamental skill. With practice and patience, you’ll become more confident and proficient in working with radicals. Remember to always simplify radical expressions and avoid common mistakes to ensure accurate results.