5 Easy Ways to Master Mixed Fractions
Understanding Mixed Fractions
Mixed fractions can seem daunting at first, but with practice and the right approach, they can become second nature. A mixed fraction is a combination of a whole number and a proper fraction. For instance, 2 1⁄2 is a mixed fraction where 2 is the whole number and 1⁄2 is the proper fraction. In this article, we’ll explore five easy ways to master mixed fractions, making it easier to work with them in various mathematical operations.
Method 1: Converting Mixed Fractions to Improper Fractions
One way to master mixed fractions is to convert them into improper fractions. An improper fraction is a fraction where the numerator is greater than the denominator. To convert a mixed fraction to an improper fraction, follow these steps:
- Multiply the whole number by the denominator.
- Add the numerator to the result.
- Keep the denominator the same.
For example, let’s convert 2 1⁄2 to an improper fraction:
- Multiply 2 (whole number) by 2 (denominator) = 4
- Add 1 (numerator) to 4 = 5
- Keep the denominator as 2
So, 2 1⁄2 = 5⁄2.
📝 Note: Converting mixed fractions to improper fractions can make it easier to perform mathematical operations, such as addition and subtraction.
Method 2: Simplifying Mixed Fractions
Simplifying mixed fractions is another way to master them. To simplify a mixed fraction, follow these steps:
- Check if the numerator and denominator have any common factors.
- If they do, divide both the numerator and denominator by the common factor.
For example, let’s simplify 4 3⁄6:
- Check if 3 and 6 have any common factors. They do (3).
- Divide both 3 and 6 by 3 = 1 and 2
- So, 4 3⁄6 = 4 1⁄2.
Method 3: Comparing Mixed Fractions
Comparing mixed fractions can be tricky, but with the right approach, it becomes easier. To compare mixed fractions, follow these steps:
- Convert both mixed fractions to improper fractions.
- Compare the numerators.
- If the numerators are equal, compare the denominators.
For example, let’s compare 2 1⁄2 and 2 1⁄3:
- Convert both mixed fractions to improper fractions: 2 1⁄2 = 5⁄2 and 2 1⁄3 = 7⁄3
- Compare the numerators: 5 and 7
- Since 5 is less than 7, 2 1⁄2 is less than 2 1⁄3.
Method 4: Adding and Subtracting Mixed Fractions
Adding and subtracting mixed fractions can be challenging, but with practice, it becomes easier. To add or subtract mixed fractions, follow these steps:
- Convert both mixed fractions to improper fractions.
- Find a common denominator.
- Add or subtract the numerators.
- Keep the denominator the same.
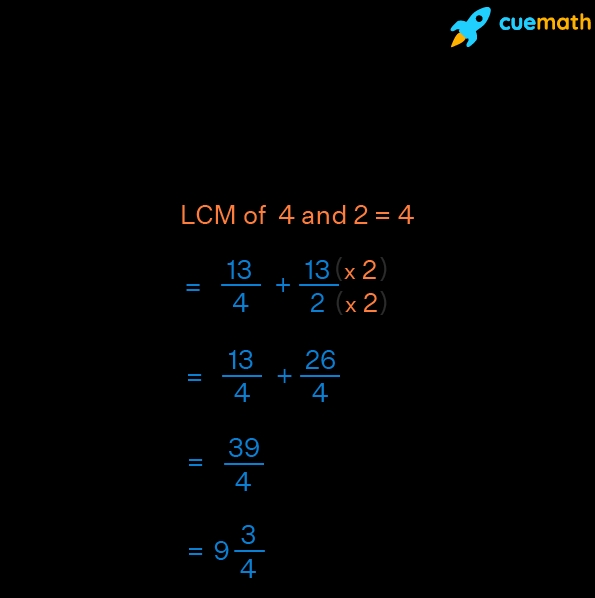
For example, let’s add 2 1⁄2 and 1 1⁄2:
- Convert both mixed fractions to improper fractions: 2 1⁄2 = 5⁄2 and 1 1⁄2 = 3⁄2
- Find a common denominator (2)
- Add the numerators: 5 + 3 = 8
- Keep the denominator the same: 8⁄2
- So, 2 1⁄2 + 1 1⁄2 = 4.
📝 Note: When adding or subtracting mixed fractions, make sure to find a common denominator to avoid errors.
Method 5: Multiplying and Dividing Mixed Fractions
Multiplying and dividing mixed fractions can be complex, but with the right approach, it becomes easier. To multiply or divide mixed fractions, follow these steps:
- Convert both mixed fractions to improper fractions.
- Multiply or divide the numerators.
- Multiply or divide the denominators.
- Simplify the result.
For example, let’s multiply 2 1⁄2 and 1 1⁄2:
- Convert both mixed fractions to improper fractions: 2 1⁄2 = 5⁄2 and 1 1⁄2 = 3⁄2
- Multiply the numerators: 5 × 3 = 15
- Multiply the denominators: 2 × 2 = 4
- Simplify the result: 15⁄4 = 3 3⁄4.
To divide mixed fractions, follow the same steps, but divide the numerators and denominators instead of multiplying.
| Mixed Fraction | Improper Fraction |
|---|---|
| 2 1/2 | 5/2 |
| 1 1/2 | 3/2 |
| 3 3/4 | 15/4 |
Mastering mixed fractions takes practice, but with the right approach, it becomes easier. By converting mixed fractions to improper fractions, simplifying, comparing, adding, subtracting, multiplying, and dividing, you’ll become a pro at working with mixed fractions.
Without a solid understanding of mixed fractions, mathematical operations can become confusing and overwhelming. However, with the five easy methods outlined in this article, you’ll be able to tackle mixed fractions with confidence.
What is a mixed fraction?
+A mixed fraction is a combination of a whole number and a proper fraction. For example, 2 1⁄2 is a mixed fraction where 2 is the whole number and 1⁄2 is the proper fraction.
How do I convert a mixed fraction to an improper fraction?
+To convert a mixed fraction to an improper fraction, multiply the whole number by the denominator, add the numerator to the result, and keep the denominator the same.
How do I simplify a mixed fraction?
+To simplify a mixed fraction, check if the numerator and denominator have any common factors. If they do, divide both the numerator and denominator by the common factor.