Add and Subtract Mixed Numbers Made Easy

Add and Subtract Mixed Numbers Made Easy

Are you struggling to add and subtract mixed numbers? Do you get confused with the whole numbers, fractions, and decimal points? Worry no more! In this post, we will break down the steps to make adding and subtracting mixed numbers a breeze.

What are Mixed Numbers?

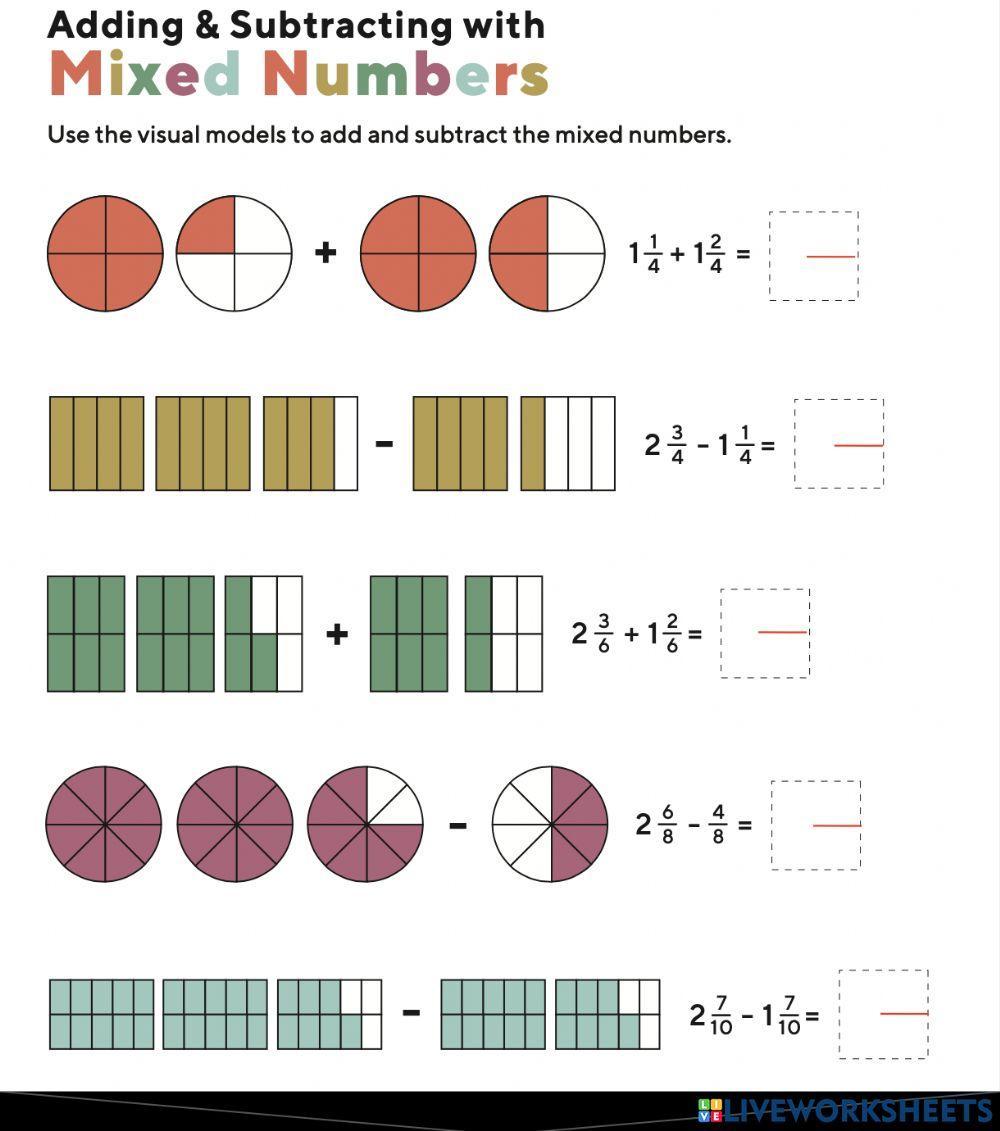
A mixed number is a combination of a whole number and a fraction. For example, 3 ¹⁄₂ is a mixed number where 3 is the whole number and ¹⁄₂ is the fraction. Mixed numbers can be simplified to improper fractions, which can be added and subtracted like regular fractions.

Adding Mixed Numbers

To add mixed numbers, follow these simple steps:

• Step 1: Convert the mixed numbers to improper fractions. To do this, multiply the whole number by the denominator and add the numerator.
• Step 2: Find a common denominator for the two improper fractions. This will ensure that you are comparing apples to apples.
• Step 3: Add the two improper fractions together.
• Step 4: Simplify the resulting improper fraction, if possible.

Here’s an example:

Example: Add 2 ¹⁄₄ and 1 ³⁄₄

• Step 1: Convert the mixed numbers to improper fractions. 2 ¹⁄₄ = ⁹⁄₄ and 1 ³⁄₄ = ⁷⁄₄
• Step 2: Find a common denominator, which is 4 in this case.
• Step 3: Add the two improper fractions together. ⁹⁄₄ + ⁷⁄₄ = ¹⁶⁄₄
• Step 4: Simplify the resulting improper fraction. ¹⁶⁄₄ = 4

Answer: 4

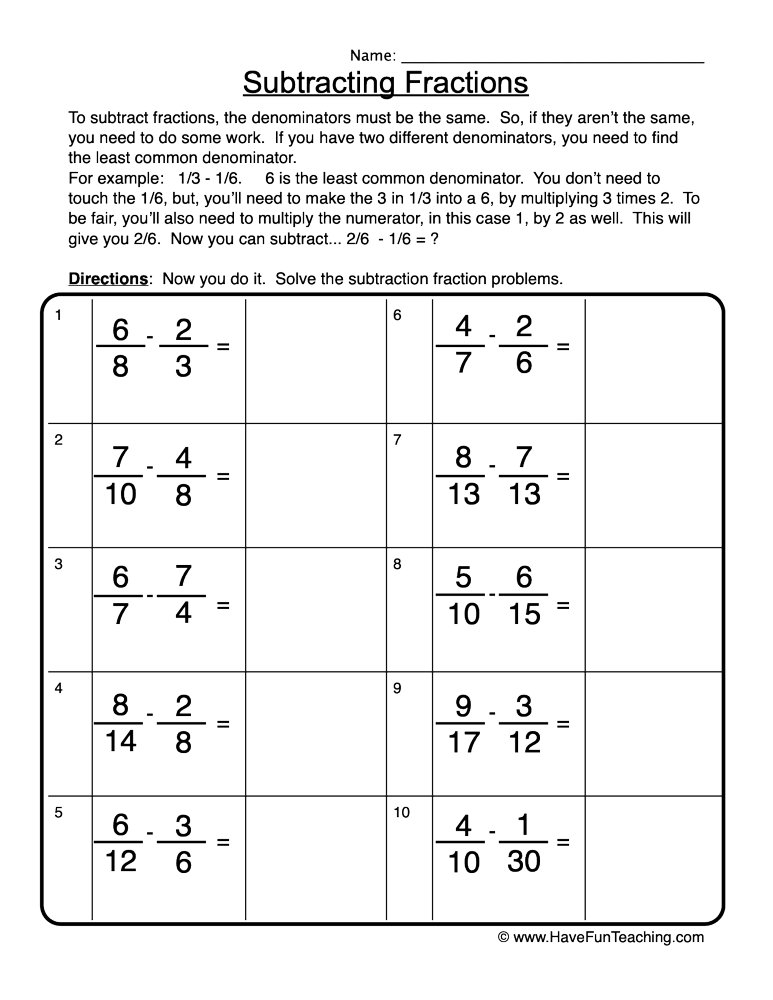
Subtracting Mixed Numbers

To subtract mixed numbers, follow these simple steps:

• Step 1: Convert the mixed numbers to improper fractions. To do this, multiply the whole number by the denominator and add the numerator.
• Step 2: Find a common denominator for the two improper fractions. This will ensure that you are comparing apples to apples.
• Step 3: Subtract the two improper fractions together.
• Step 4: Simplify the resulting improper fraction, if possible.

Here’s an example:

Example: Subtract 3 ¹⁄₂ from 4 ³⁄₄

• Step 1: Convert the mixed numbers to improper fractions. 3 ¹⁄₂ = ⁷⁄₂ and 4 ³⁄₄ = ¹⁹⁄₄
• Step 2: Find a common denominator, which is 4 in this case. Convert ⁷⁄₂ to ¹⁴⁄₄.
• Step 3: Subtract the two improper fractions together. ¹⁹⁄₄ - ¹⁴⁄₄ = ⁵⁄₄
• Step 4: Simplify the resulting improper fraction. ⁵⁄₄ = 1 ¹⁄₄

Answer: 1 ¹⁄₄

Real-World Applications

Adding and subtracting mixed numbers is a crucial skill in real-world applications such as:

• Cooking: Recipes often require adding and subtracting mixed numbers of ingredients.
• Construction: Building projects require adding and subtracting mixed numbers of measurements.
• Finance: Budgeting and financial planning require adding and subtracting mixed numbers of dollars and cents.

Common Mistakes

Here are some common mistakes to avoid when adding and subtracting mixed numbers:

• Not converting to improper fractions: Make sure to convert mixed numbers to improper fractions before adding or subtracting.
• Not finding a common denominator: Ensure that you find a common denominator for the two improper fractions.
• Not simplifying the resulting fraction: Simplify the resulting improper fraction, if possible.

💡 Note: Practice makes perfect! Make sure to practice adding and subtracting mixed numbers with different denominators and whole numbers.

In conclusion, adding and subtracting mixed numbers is a straightforward process that requires converting to improper fractions, finding a common denominator, and simplifying the resulting fraction. With practice and patience, you will become a pro at adding and subtracting mixed numbers in no time!

Related Terms:

• Fraction addition and subtraction worksheet
• Multiplication mixed number worksheet