Solve with Ease: 8th Grade Equations Worksheet Guide

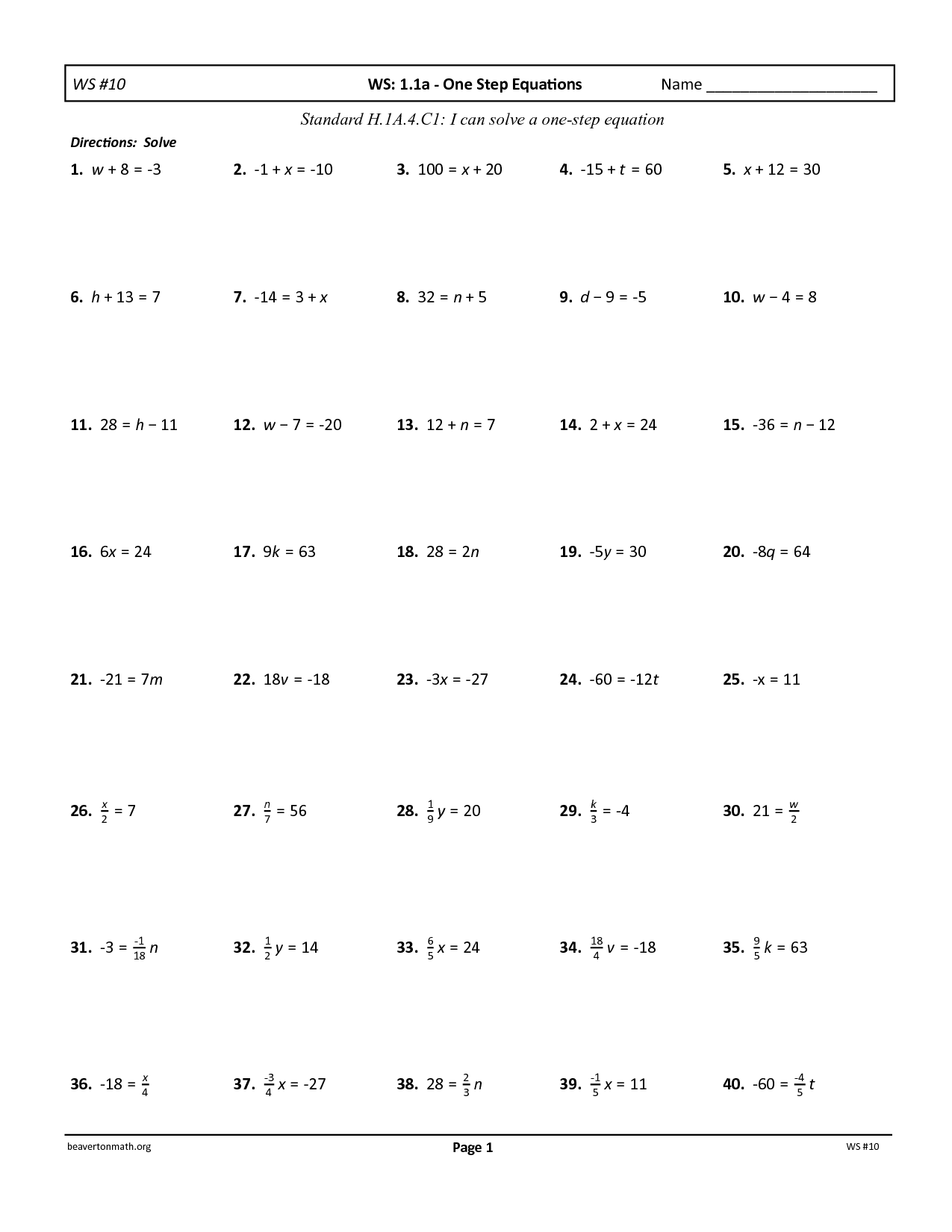
Understanding 8th Grade Equations: A Comprehensive Guide

As students progress through their 8th-grade math curriculum, they encounter more complex equations that require a deeper understanding of algebraic concepts. This guide aims to provide a thorough explanation of 8th-grade equations, along with practical examples and tips to help students solve them with ease.

What are Equations?

An equation is a mathematical statement that expresses the equality of two expressions, often containing variables, constants, and mathematical operations. Equations are fundamental to algebra and are used to solve problems in various fields, including physics, engineering, and economics.

Types of Equations in 8th Grade

In 8th grade, students typically encounter the following types of equations:

• Linear Equations: Equations in which the highest power of the variable(s) is 1. Examples: 2x + 3 = 5, x - 2 = 3
• Quadratic Equations: Equations in which the highest power of the variable(s) is 2. Examples: x^2 + 4x + 4 = 0, x^2 - 3x - 2 = 0
• Systems of Equations: A set of two or more equations that must be solved simultaneously. Examples: 2x + y = 4, x - 2y = -3

How to Solve Linear Equations

Solving linear equations involves isolating the variable on one side of the equation. Here are the general steps:

1. Add or subtract the same value to both sides: This helps to eliminate any constants on the same side as the variable.
2. Multiply or divide both sides by the same value: This helps to eliminate any coefficients (numbers multiplied by the variable).
3. Simplify the equation: Combine like terms and simplify the equation to its simplest form.

Example: Solve for x in the equation 2x + 3 = 5

• Subtract 3 from both sides: 2x = 5 - 3
• Simplify: 2x = 2
• Divide both sides by 2: x = ²⁄₂
• Simplify: x = 1

How to Solve Quadratic Equations

Solving quadratic equations involves factoring, using the quadratic formula, or completing the square. Here are the general steps:

1. Factor the equation (if possible): Look for two binomials that multiply to equal the quadratic expression.
2. Use the quadratic formula (if factoring is not possible): The quadratic formula is x = (-b ± √(b^2 - 4ac)) / 2a
3. Complete the square (if factoring is not possible): Rewrite the equation in the form (x + a)^2 = b

Example: Solve for x in the equation x^2 + 4x + 4 = 0

• Factor the equation: (x + 2)(x + 2) = 0
• Solve for x: x + 2 = 0 or x + 2 = 0
• Simplify: x = -2 or x = -2

How to Solve Systems of Equations

Solving systems of equations involves using substitution, elimination, or graphing. Here are the general steps:

1. Use substitution (if one equation is already solved for one variable): Substitute the expression for one variable into the other equation.
2. Use elimination (if the coefficients of one variable are the same in both equations): Add or subtract the equations to eliminate one variable.
3. Use graphing (if the equations are linear): Graph the equations on a coordinate plane and find the point of intersection.

Example: Solve the system of equations 2x + y = 4 and x - 2y = -3

• Use substitution: Substitute x = 2y - 3 into the first equation
• Simplify: 2(2y - 3) + y = 4
• Solve for y: 4y - 6 + y = 4
• Simplify: 5y = 10
• Solve for y: y = 2
• Substitute y back into one of the original equations to find x

💡 Note: Always check your solutions by plugging them back into the original equations to ensure they are true.

Conclusion

Solving equations is a fundamental skill in mathematics, and with practice and patience, 8th-grade students can master the techniques needed to solve linear, quadratic, and systems of equations. Remember to always follow the order of operations, simplify expressions, and check solutions to ensure accuracy.

Related Terms:

• Worksheet Algebra Grade 8
• Solving equations grade 8 Worksheet
• Fraction Grade 8 worksheet
• Math grade 8 worksheets pdf
• Math for grade 1 worksheet
• Gradient worksheet Grade 8