6 Ways to Master 6th Grade Exponents
Understanding Exponents: A Foundational Concept in Mathematics
Exponents are a fundamental concept in mathematics, and mastering them is crucial for success in various mathematical disciplines, including algebra, geometry, and calculus. In 6th grade, students are introduced to exponents, which can seem daunting at first, but with practice and the right approach, they can become proficient in this area. In this article, we will explore six ways to master 6th grade exponents, making it easier for students to grasp this concept.
1. Start with the Basics: Understanding Exponent Notation
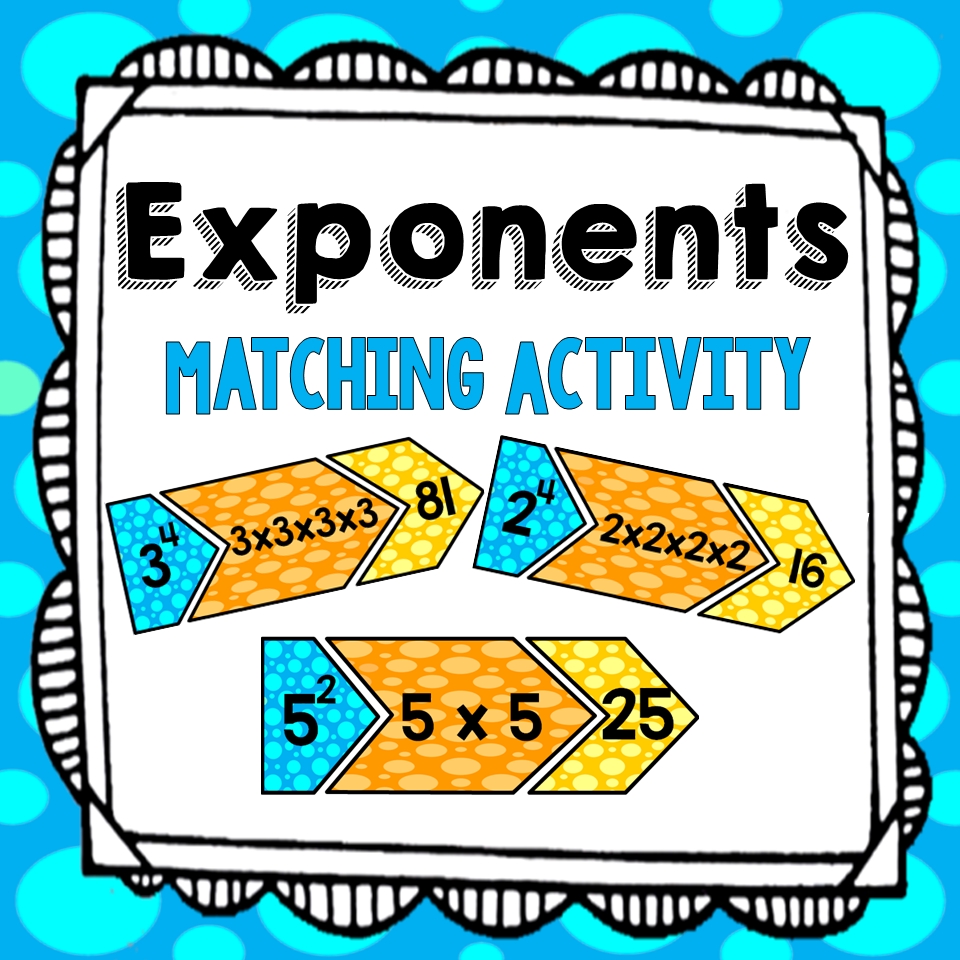
To master exponents, it’s essential to understand the notation. An exponent is a small number that is raised to a power, indicating how many times the base number should be multiplied by itself. For example, in the expression 2^3, the base is 2, and the exponent is 3. This expression means 2 multiplied by itself three times, resulting in 8.
Exponent Notation:
- A small number that indicates the power to which the base number should be raised
- Also known as the “power” or “index”
- Placed above and to the right of the base number
2. Practice, Practice, Practice: Simplifying Exponents
Practice is key to mastering exponents. Start by simplifying expressions with exponents. For example:
- 2^1 = 2
- 2^2 = 4
- 2^3 = 8
- 2^4 = 16
As you become more comfortable, move on to more complex expressions, such as:
- 3^2 = 9
- 4^3 = 64
- 5^2 = 25
📝 Note: Use flashcards or create a chart to help you memorize the exponent values.
3. Visualize the Process: Using Real-World Examples
Exponents can be difficult to understand, but using real-world examples can help make the concept more tangible. For instance:
- A population of bacteria doubles every hour. If there are 2 bacteria initially, how many will there be after 3 hours?
- 2^1 = 2 (initial population)
- 2^2 = 4 (after 1 hour)
- 2^3 = 8 (after 2 hours)
- 2^4 = 16 (after 3 hours)
This example illustrates how exponents can be used to model real-world situations.
4. Apply the Rules: Exponent Properties
Exponent properties are essential to understanding how to manipulate expressions with exponents. The three main properties are:
- Product of Powers: a^m × a^n = a^(m+n)
- Power of a Power: (a^m)^n = a^(m×n)
- Power of a Product: (ab)^m = a^m × b^m
These properties can help you simplify complex expressions and make calculations more efficient.

| Property | Rule | Example |
|---|---|---|
| Product of Powers | a^m × a^n = a^(m+n) | 2^2 × 2^3 = 2^(2+3) = 2^5 |
| Power of a Power | (a^m)^n = a^(m×n) | (2^2)^3 = 2^(2×3) = 2^6 |
| Power of a Product | (ab)^m = a^m × b^m | (2×3)^2 = 2^2 × 3^2 = 4 × 9 = 36 |
5. Use Online Resources: Interactive Tools and Games
There are many online resources available to help you master exponents, including interactive tools and games. Some popular options include:
- Khan Academy: Exponents
- Mathway: Exponents
- IXL: Exponents
These resources can provide additional practice and help you stay engaged.
6. Seek Help When Needed: Ask a Teacher or Tutor
Finally, don’t be afraid to ask for help if you’re struggling with exponents. Reach out to your teacher or a tutor for additional support. They can provide one-on-one guidance and help you understand the concepts more clearly.
By following these six steps, you’ll be well on your way to mastering 6th grade exponents. Remember to practice regularly, use real-world examples, and apply the exponent properties to become more confident in your abilities.
In conclusion, mastering exponents takes time and practice, but with the right approach, you can become proficient in this area. By starting with the basics, practicing regularly, and using real-world examples, you’ll be able to simplify expressions and apply exponent properties with ease. Don’t be afraid to ask for help when needed, and take advantage of online resources to stay engaged.
What is the difference between a base and an exponent?
+The base is the number that is being raised to a power, while the exponent is the small number that indicates the power to which the base should be raised.
How do I simplify expressions with exponents?
+To simplify expressions with exponents, apply the exponent properties, such as the product of powers, power of a power, and power of a product.
What are some real-world applications of exponents?
+Exponents are used in various real-world applications, such as population growth, chemical reactions, and finance.
Related Terms:
- Exponents worksheets PDF with answers
- Free printable exponents worksheets
- Fraction to decimal grade 6
- Exponents worksheets Grade 7 PDF