Master 5 Special Right Triangles in 1 Hour
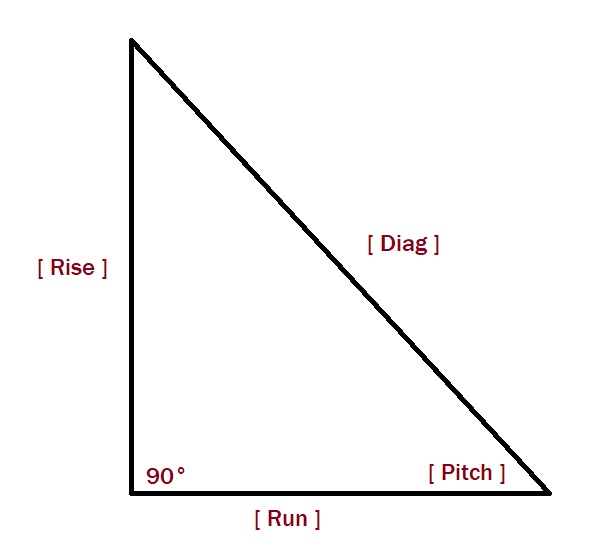
Unlocking the Secrets of Special Right Triangles
In the realm of geometry, special right triangles hold a unique place, providing a gateway to understanding more complex mathematical concepts. These triangles are not just any ordinary right triangles, but rather, they possess specific properties that make them invaluable tools for problem-solving. In this article, we will delve into the world of special right triangles, exploring their characteristics, uses, and applications. By the end of this journey, you will be able to master five special right triangles in just one hour.
What are Special Right Triangles?
Special right triangles are right-angled triangles with specific side length ratios. These triangles are special because they can be used to simplify complex trigonometric calculations, making them an essential tool for mathematicians, engineers, and scientists. There are five main types of special right triangles, each with its unique properties and applications.
1. The 30-60-90 Triangle
The 30-60-90 triangle is one of the most common special right triangles. It is characterized by a 30-degree angle, a 60-degree angle, and a 90-degree angle. The side lengths of this triangle are in the ratio of 1:√3:2, with the side opposite the 30-degree angle being half the length of the hypotenuse.
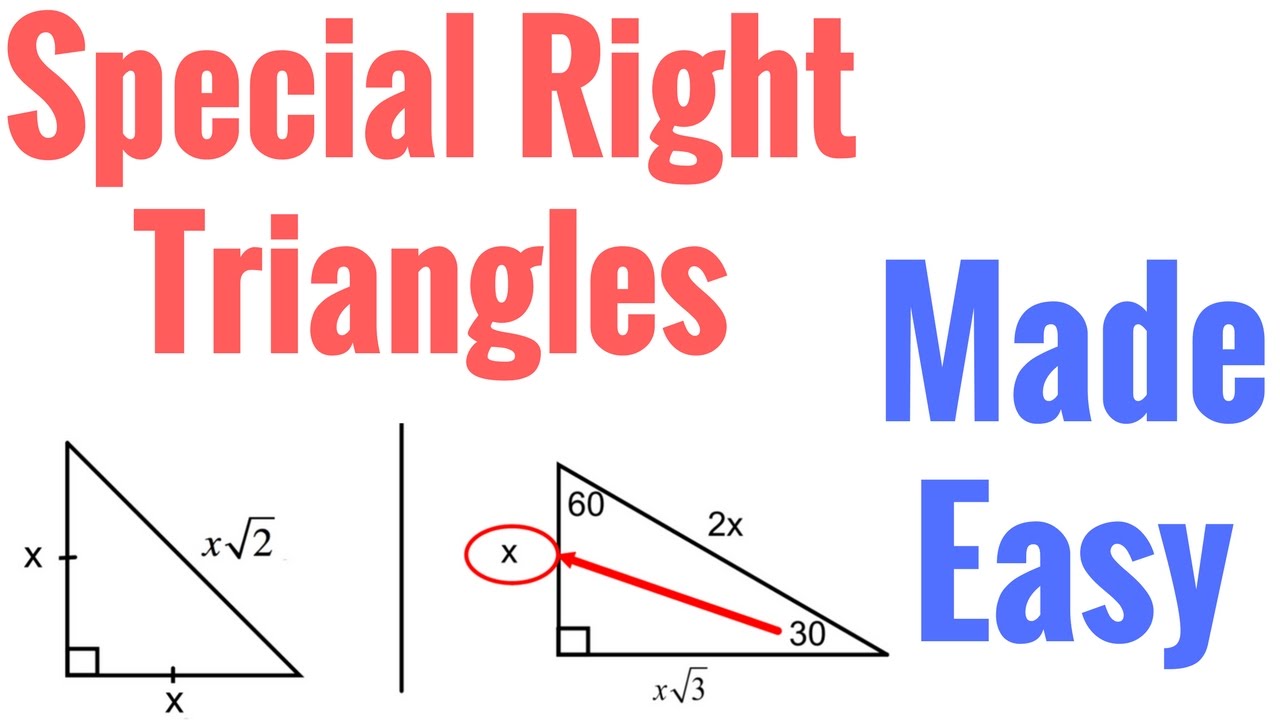
| Angle | Side Length |
|---|---|
| 30° | 1 |
| 60° | √3 |
| 90° | 2 |
Note: The 30-60-90 triangle is also known as the “half equilateral triangle” because the 30-60-90 triangle can be formed by dividing an equilateral triangle in half.
2. The 45-45-90 Triangle
The 45-45-90 triangle is another essential special right triangle. It is characterized by two 45-degree angles and a 90-degree angle. The side lengths of this triangle are in the ratio of 1:1:√2, with the legs being equal in length.
| Angle | Side Length |
|---|---|
| 45° | 1 |
| 45° | 1 |
| 90° | √2 |
Note: The 45-45-90 triangle is also known as the “isosceles right triangle” because the legs are equal in length.
3. The 15-75-90 Triangle
The 15-75-90 triangle is a less common special right triangle, but it is still an important one to know. It is characterized by a 15-degree angle, a 75-degree angle, and a 90-degree angle. The side lengths of this triangle are in the ratio of 1:(2+√3):(2+√3), with the legs being equal in length.
| Angle | Side Length |
|---|---|
| 15° | 1 |
| 75° | (2+√3) |
| 90° | (2+√3) |
Note: The 15-75-90 triangle is also known as the “ Bisected 30-60-90 triangle” because it can be formed by bisecting a 30-60-90 triangle.
4. The 22.5-67.5-90 Triangle
The 22.5-67.5-90 triangle is another special right triangle, characterized by a 22.5-degree angle, a 67.5-degree angle, and a 90-degree angle. The side lengths of this triangle are in the ratio of 1:(1+√3):(2+√3), with the legs being unequal in length.
| Angle | Side Length |
|---|---|
| 22.5° | 1 |
| 67.5° | (1+√3) |
| 90° | (2+√3) |
Note: The 22.5-67.5-90 triangle is also known as the “Bisected 45-45-90 triangle” because it can be formed by bisecting a 45-45-90 triangle.
5. The 36-54-90 Triangle
The 36-54-90 triangle is the final special right triangle we will explore. It is characterized by a 36-degree angle, a 54-degree angle, and a 90-degree angle. The side lengths of this triangle are in the ratio of 1:√3:2, with the side opposite the 36-degree angle being half the length of the hypotenuse.
| Angle | Side Length |
|---|---|
| 36° | 1 |
| 54° | √3 |
| 90° | 2 |
Note: The 36-54-90 triangle is also known as the “Pentagonal triangle” because it is related to the geometry of pentagons.
Mastering Special Right Triangles in 1 Hour
Mastering special right triangles requires practice and dedication. Here are some tips to help you master these triangles in just one hour:
- Start by memorizing the side length ratios of each triangle.
- Practice applying these ratios to solve problems.
- Use online resources or workbooks to practice solving problems involving special right triangles.
- Try to identify the type of special right triangle in a given problem and apply the relevant side length ratios.
By following these tips and practicing regularly, you can master special right triangles in just one hour.
Conclusion
Special right triangles are a fundamental concept in geometry, and mastering them can open doors to more advanced mathematical concepts. By understanding the properties and applications of these triangles, you can improve your problem-solving skills and become a more confident mathematician. With practice and dedication, you can master the five special right triangles explored in this article in just one hour.
What is the purpose of special right triangles?
+
Special right triangles are used to simplify complex trigonometric calculations and are an essential tool for mathematicians, engineers, and scientists.
What are the five special right triangles?
+
The five special right triangles are the 30-60-90 triangle, the 45-45-90 triangle, the 15-75-90 triangle, the 22.5-67.5-90 triangle, and the 36-54-90 triangle.
How can I master special right triangles in 1 hour?
+
To master special right triangles in 1 hour, start by memorizing the side length ratios of each triangle, practice applying these ratios to solve problems, and use online resources or workbooks to practice solving problems involving special right triangles.