5 Ways to Solve 2-Step Inequalities
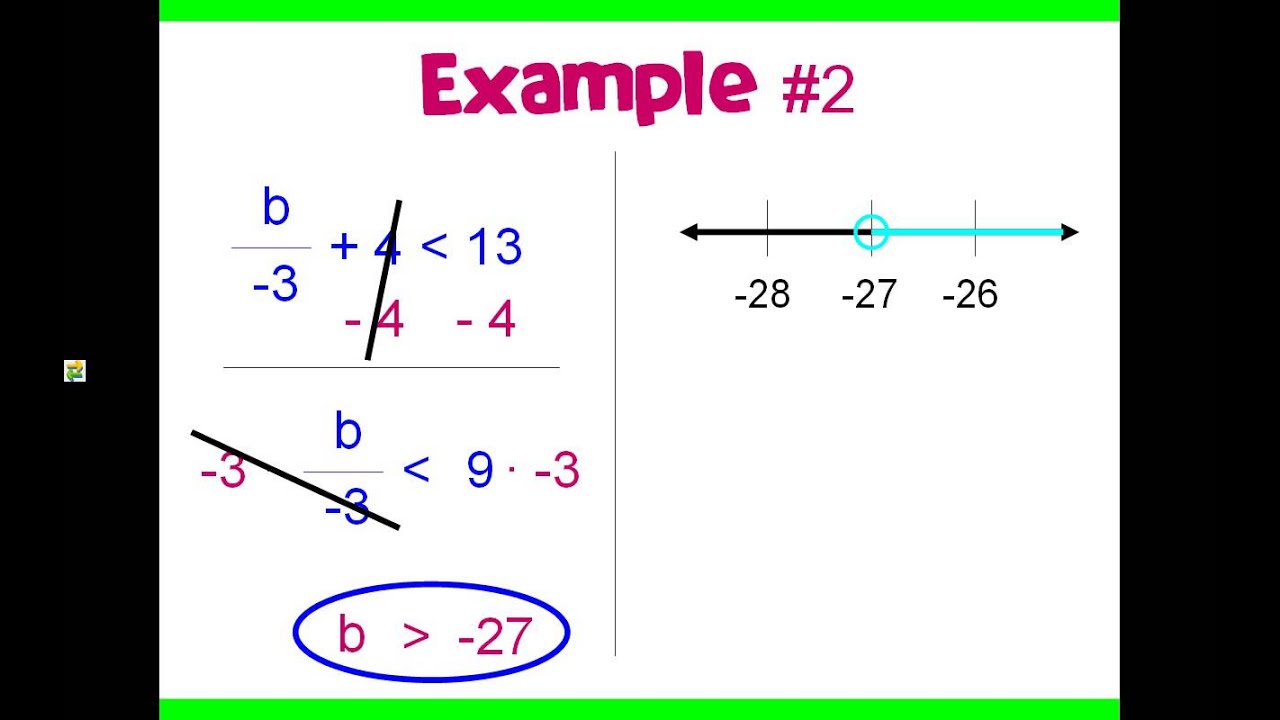
Understanding 2-Step Inequalities
Inequalities are a fundamental concept in mathematics, and they play a crucial role in various fields, including algebra, geometry, and calculus. A 2-step inequality is a type of inequality that requires two operations to solve. In this article, we will explore five ways to solve 2-step inequalities, and provide you with a comprehensive guide to help you master this concept.
What are 2-Step Inequalities?
A 2-step inequality is an inequality that requires two operations to solve. It involves two variables, and the solution requires two steps to isolate the variable. For example, 2x + 5 > 11 is a 2-step inequality because it requires two operations (subtracting 5 and then dividing by 2) to solve for x.
Method 1: Adding or Subtracting the Same Value
One way to solve 2-step inequalities is by adding or subtracting the same value to both sides of the inequality. This method is useful when the inequality has a constant term on one side.
Example: Solve for x in the inequality 2x + 5 > 11
Step 1: Subtract 5 from both sides of the inequality 2x + 5 - 5 > 11 - 5 2x > 6
Step 2: Divide both sides of the inequality by 2 2x / 2 > 6 / 2 x > 3
Therefore, the solution to the inequality 2x + 5 > 11 is x > 3.
Method 2: Multiplying or Dividing by the Same Value
Another way to solve 2-step inequalities is by multiplying or dividing both sides of the inequality by the same value. This method is useful when the inequality has a coefficient on the variable.
Example: Solve for x in the inequality 4x / 2 < 12
Step 1: Multiply both sides of the inequality by 2 (4x / 2) * 2 < 12 * 2 4x < 24
Step 2: Divide both sides of the inequality by 4 4x / 4 < 24 / 4 x < 6
Therefore, the solution to the inequality 4x / 2 < 12 is x < 6.
Method 3: Using Inverse Operations
Inverse operations can also be used to solve 2-step inequalities. Inverse operations are operations that “undo” each other, such as addition and subtraction, or multiplication and division.
Example: Solve for x in the inequality x - 3 > 7
Step 1: Add 3 to both sides of the inequality x - 3 + 3 > 7 + 3 x > 10
Therefore, the solution to the inequality x - 3 > 7 is x > 10.
Method 4: Using the Properties of Inequalities
The properties of inequalities can also be used to solve 2-step inequalities. For example, if a > b, then a + c > b + c, and if a > b, then ac > bc.
Example: Solve for x in the inequality x + 2 > 5
Step 1: Subtract 2 from both sides of the inequality x + 2 - 2 > 5 - 2 x > 3
Therefore, the solution to the inequality x + 2 > 5 is x > 3.
Method 5: Graphing the Inequality
Graphing the inequality is another way to solve 2-step inequalities. This method involves graphing the related equation and then testing points to determine the solution.
Example: Solve for x in the inequality 2x - 3 > 5
Step 1: Graph the related equation 2x - 3 = 5
Step 2: Test points to determine the solution
Therefore, the solution to the inequality 2x - 3 > 5 is x > 4.
🤔 Note: Graphing the inequality can be time-consuming, and it may not always be the most efficient method. However, it can be a useful method for visualizing the solution.
In conclusion, solving 2-step inequalities requires a combination of algebraic manipulations and logical reasoning. By using the five methods outlined in this article, you can develop a deeper understanding of 2-step inequalities and become more proficient in solving them.
What is a 2-step inequality?
+A 2-step inequality is an inequality that requires two operations to solve. It involves two variables, and the solution requires two steps to isolate the variable.
How do I solve a 2-step inequality?
+There are five ways to solve 2-step inequalities: adding or subtracting the same value, multiplying or dividing by the same value, using inverse operations, using the properties of inequalities, and graphing the inequality.
What is the difference between a 1-step inequality and a 2-step inequality?
+A 1-step inequality requires only one operation to solve, whereas a 2-step inequality requires two operations to solve.
Related Terms:
- two-step inequalities worksheet 7th grade
- One-Step Inequalities Worksheet PDF