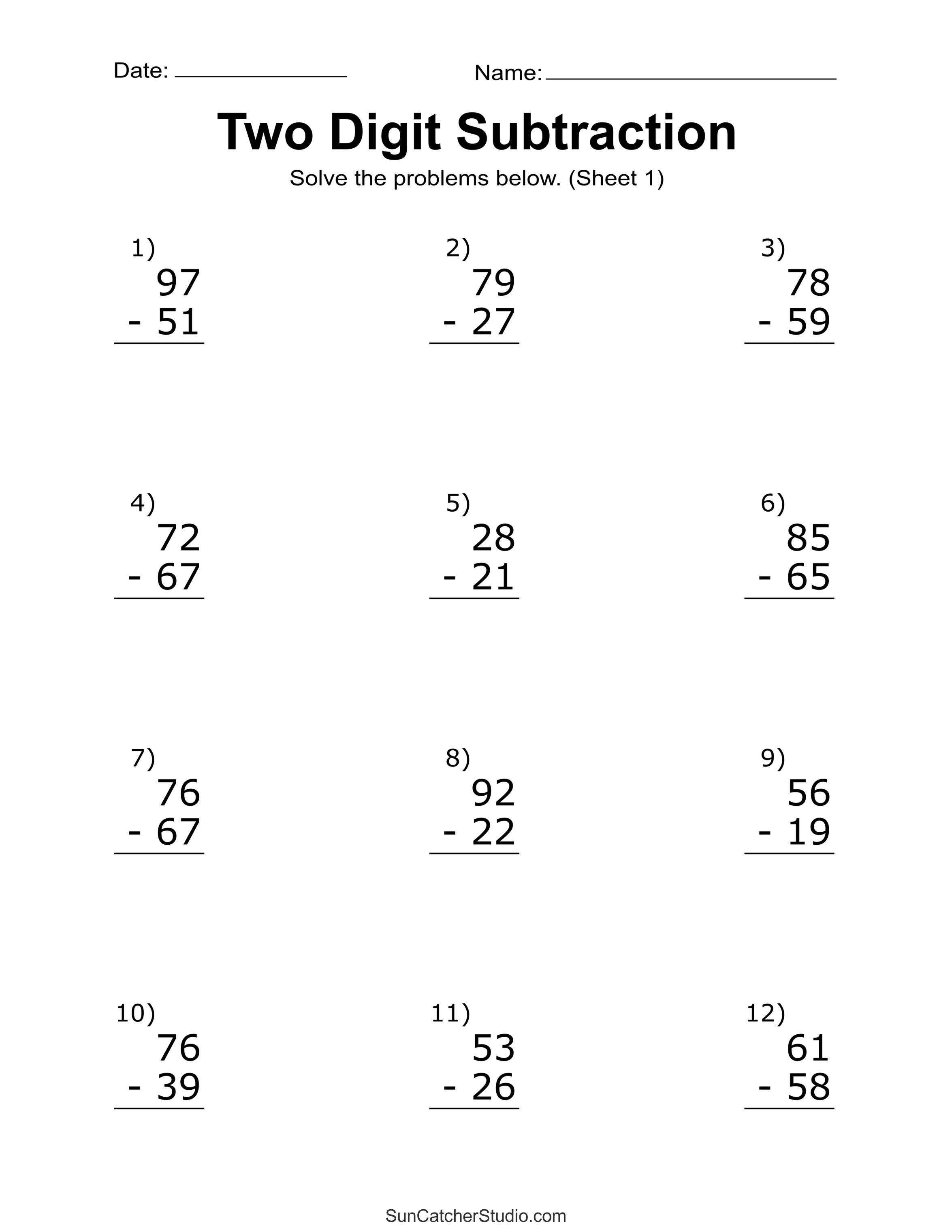
Write an Equation From a Table Worksheet
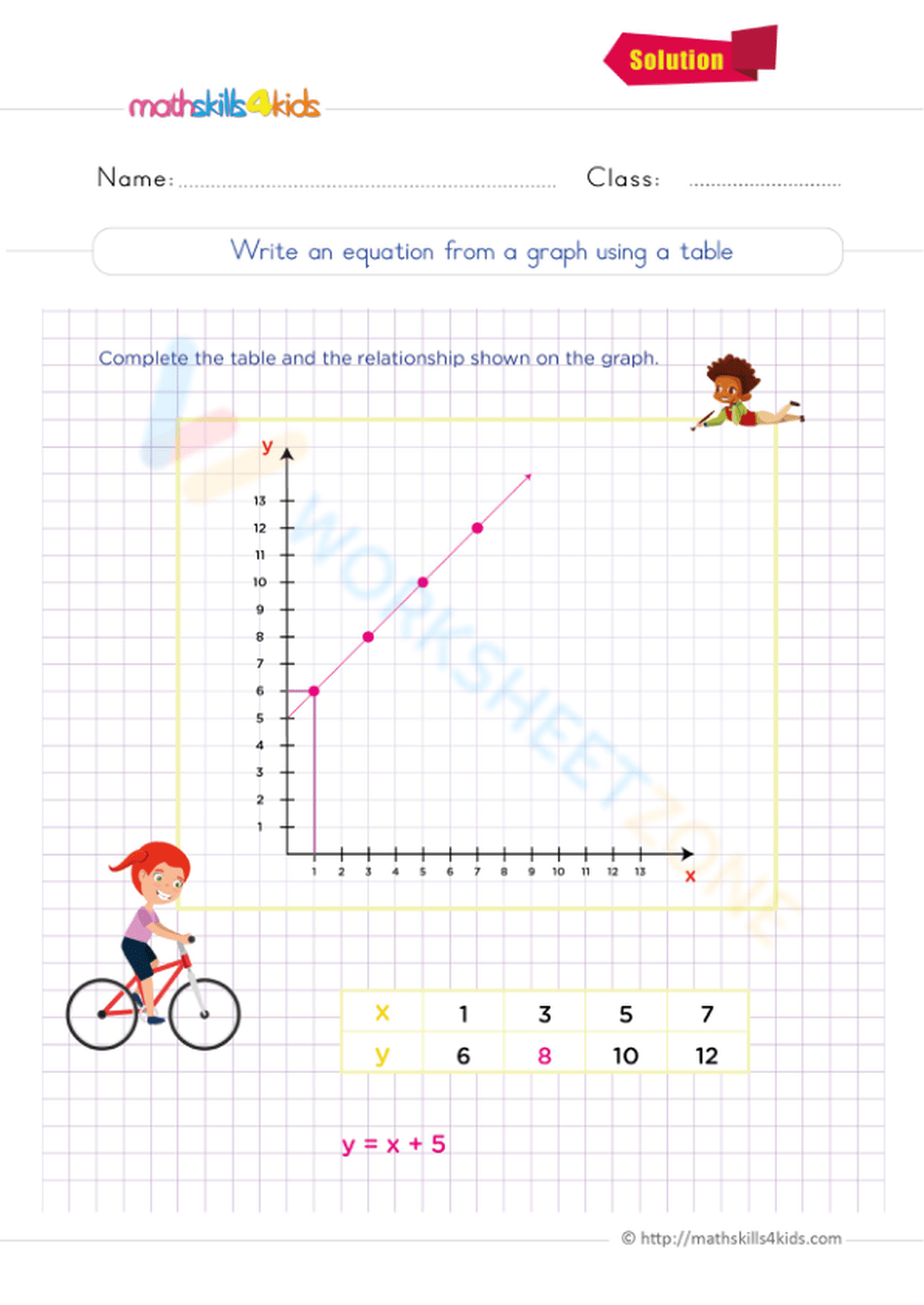
Creating an Equation from a Table Worksheet
When working with tables, it’s often useful to create an equation that represents the relationship between the variables. This can help us make predictions, identify patterns, and solve problems more efficiently. In this worksheet, we’ll practice creating an equation from a table.
Example 1: Simple Linear Relationship

| x | y |
|---|---|
| 1 | 2 |
| 2 | 4 |
| 3 | 6 |
| 4 | 8 |
Look at the table above. Can you identify the pattern in the data?
🤔 Note: Take a closer look at the relationship between x and y. Can you see a simple linear pattern?
To create an equation from this table, we need to find the rate of change between x and y. In this case, for every 1-unit increase in x, y increases by 2 units. This tells us that the slope of the equation is 2.
Now, let’s find the y-intercept. Looking at the table, we see that when x is 0 (not shown in the table), y would be 0 as well. Therefore, the y-intercept is 0.
Using the slope-intercept form of a linear equation (y = mx + b), where m is the slope and b is the y-intercept, we can write the equation as:
y = 2x
Example 2: More Complex Linear Relationship
| x | y |
|---|---|
| 2 | 5 |
| 4 | 9 |
| 6 | 13 |
| 8 | 17 |
Can you identify the pattern in the data?
🤔 Note: Take a closer look at the relationship between x and y. Can you see a linear pattern, but with a different slope and y-intercept?
To create an equation from this table, we need to find the rate of change between x and y. In this case, for every 2-unit increase in x, y increases by 4 units. This tells us that the slope of the equation is 2.
Now, let’s find the y-intercept. Looking at the table, we see that when x is 0 (not shown in the table), y would be 1. Therefore, the y-intercept is 1.
Using the slope-intercept form of a linear equation (y = mx + b), we can write the equation as:
y = 2x + 1
Your Turn!
| x | y |
|---|---|
| 1 | 3 |
| 2 | 6 |
| 3 | 9 |
| 4 | 12 |
Can you create an equation from this table? Remember to identify the pattern, find the slope, and determine the y-intercept.
Step-by-Step Solution
- Identify the pattern in the data.
- Find the rate of change between x and y.
- Determine the slope of the equation.
- Find the y-intercept.
- Write the equation in slope-intercept form (y = mx + b).
Tips and Variations
- When working with tables, look for patterns and relationships between the variables.
- Use the slope-intercept form of a linear equation to write the equation.
- If the relationship is not linear, you may need to use a different type of equation, such as a quadratic or exponential equation.
- Practice, practice, practice! The more you work with tables and equations, the more comfortable you’ll become with identifying patterns and creating equations.
Equation Writing Tips
- Always identify the pattern in the data before trying to write an equation.
- Use the slope-intercept form of a linear equation (y = mx + b) whenever possible.
- Check your work by plugging in values from the table to make sure the equation is correct.
What is the slope-intercept form of a linear equation?
+The slope-intercept form of a linear equation is y = mx + b, where m is the slope and b is the y-intercept.
How do I find the slope of an equation from a table?
+To find the slope, identify the rate of change between x and y. For every 1-unit increase in x, how much does y increase or decrease?
What is the y-intercept of an equation?
+The y-intercept is the value of y when x is 0. It's the point where the equation crosses the y-axis.
In conclusion, creating an equation from a table is an essential skill in mathematics and science. By identifying patterns and relationships between variables, we can write equations that help us make predictions, identify trends, and solve problems more efficiently. Remember to practice, practice, practice, and don’t be afraid to ask for help when you need it!