Mastering Significant Figures and Scientific Notation

Understanding Significant Figures

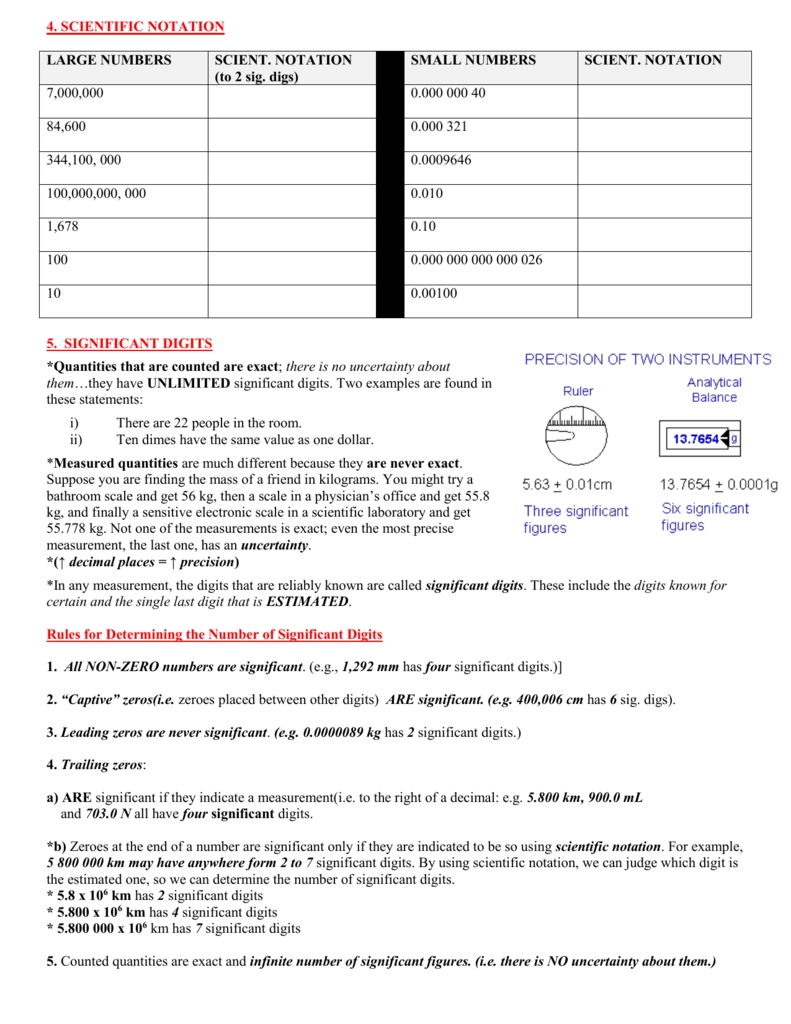
Significant figures are a fundamental concept in science and engineering, as they help us express measurements with the correct level of precision. In this section, we will explore the rules for determining significant figures and provide examples to illustrate each rule.

Rule 1: Non-zero digits are always significant

Any non-zero digit in a measurement is considered significant. For example, the number 456 has three significant figures.

Rule 2: Zeros between non-zero digits are significant

If a zero is sandwiched between two non-zero digits, it is considered significant. For instance, the number 405 has three significant figures.

Rule 3: Leading zeros are not significant

Leading zeros are not considered significant, as they simply indicate the magnitude of the measurement. For example, the number 0.045 has two significant figures.

Rule 4: Trailing zeros are significant if the number contains a decimal point

If a number contains a decimal point, trailing zeros are considered significant. For example, the number 45.00 has four significant figures.

Rule 5: Trailing zeros are not significant if the number does not contain a decimal point

If a number does not contain a decimal point, trailing zeros are not considered significant. For example, the number 4500 has two significant figures.

📝 Note: It's essential to understand these rules to accurately express measurements and avoid confusion.

Understanding Scientific Notation

Scientific notation is a compact way to express very large or very small numbers. It consists of a number between 1 and 10, multiplied by a power of 10.

The General Form of Scientific Notation

A number in scientific notation is written in the form:

a × 10^n

where a is a number between 1 and 10, and n is an integer.

Examples of Scientific Notation

• The number 456 can be written in scientific notation as 4.56 × 10^2.
• The number 0.045 can be written in scientific notation as 4.5 × 10^-2.
• The number 4500 can be written in scientific notation as 4.5 × 10^3.

📝 Note: Scientific notation is widely used in science and engineering to simplify complex calculations and express large or small numbers in a compact form.

Converting Between Standard and Scientific Notation

To convert a number from standard notation to scientific notation, follow these steps:

1. Move the decimal point to the left or right until you have a number between 1 and 10.
2. Count the number of places you moved the decimal point. This will be the exponent of 10.
3. Write the number in the form a × 10^n.

Examples of Converting to Scientific Notation

• The number 456 can be converted to scientific notation by moving the decimal point two places to the left, resulting in 4.56 × 10^2.
• The number 0.045 can be converted to scientific notation by moving the decimal point two places to the right, resulting in 4.5 × 10^-2.

To convert a number from scientific notation to standard notation, follow these steps:

1. Multiply the number by 10 raised to the power of the exponent.
2. Move the decimal point accordingly.

Examples of Converting to Standard Notation

• The number 4.56 × 10^2 can be converted to standard notation by multiplying by 10^2, resulting in 456.
• The number 4.5 × 10^-2 can be converted to standard notation by multiplying by 10^-2, resulting in 0.045.

Applying Significant Figures and Scientific Notation

In this section, we will explore how to apply significant figures and scientific notation in real-world scenarios.

Example 1: Measuring the Length of a Room

Suppose you measure the length of a room to be 12.5 meters. Using significant figures, you would express this measurement as 12.5 m, indicating three significant figures.

Example 2: Calculating the Volume of a Container

Suppose you need to calculate the volume of a container with a length of 5.00 meters, a width of 2.00 meters, and a height of 1.50 meters. Using scientific notation, you would express these measurements as:

Length: 5.00 × 10^0 m Width: 2.00 × 10^0 m Height: 1.50 × 10^0 m

You would then calculate the volume by multiplying these measurements together.

In summary, significant figures and scientific notation are essential tools in science and engineering, helping us express measurements with precision and simplify complex calculations. By understanding the rules and applications of significant figures and scientific notation, you can improve your accuracy and efficiency in scientific and engineering contexts.