Complementary and Supplementary Angles Worksheet Made Easy

Understanding Angles in Geometry
Geometry is a fundamental branch of mathematics that deals with the study of shapes, sizes, and positions of objects. One of the basic concepts in geometry is angles. An angle is formed when two rays share a common endpoint, known as the vertex. In this article, we will explore complementary and supplementary angles, and provide a worksheet to help you practice.
What are Complementary Angles?
Complementary angles are two angles whose measures add up to 90 degrees. In other words, if we have two angles whose sum is 90 degrees, they are complementary angles. For example, if we have an angle measuring 30 degrees, its complementary angle would be 60 degrees (since 30 + 60 = 90).
Characteristics of Complementary Angles
Here are some key characteristics of complementary angles:
- The sum of two complementary angles is always 90 degrees.
- Complementary angles can be adjacent (share a common side) or non-adjacent.
- Complementary angles are used to solve problems involving right triangles.
What are Supplementary Angles?
Supplementary angles are two angles whose measures add up to 180 degrees. In other words, if we have two angles whose sum is 180 degrees, they are supplementary angles. For example, if we have an angle measuring 120 degrees, its supplementary angle would be 60 degrees (since 120 + 60 = 180).
Characteristics of Supplementary Angles
Here are some key characteristics of supplementary angles:
- The sum of two supplementary angles is always 180 degrees.
- Supplementary angles can be adjacent (share a common side) or non-adjacent.
- Supplementary angles are used to solve problems involving straight lines and angles.
Complementary and Supplementary Angles Worksheet
Now, it’s time to practice! Here’s a worksheet with 10 problems to help you understand complementary and supplementary angles:
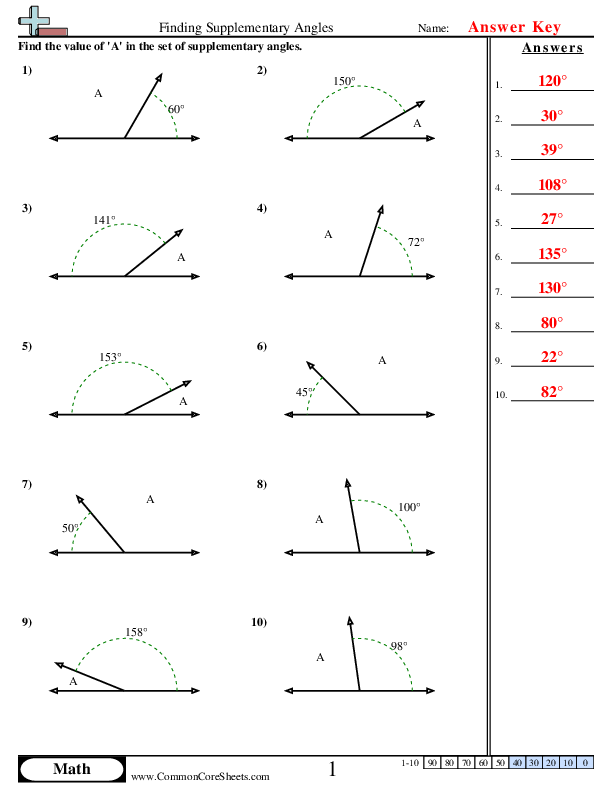
| Problem # | Angle 1 | Angle 2 | Find… |
|---|---|---|---|
| 1 | 30 | x | x |
| 2 | x | 60 | x |
| 3 | 120 | x | x |
| 4 | x | 90 | x |
| 5 | 45 | x | x |
| 6 | x | 120 | x |
| 7 | 75 | x | x |
| 8 | x | 45 | x |
| 9 | 90 | x | x |
| 10 | x | 75 | x |
🤔 Note: Use the definitions of complementary and supplementary angles to find the missing angle measures.
Solutions
Here are the solutions to the worksheet:
| Problem # | Angle 1 | Angle 2 | Answer |
|---|---|---|---|
| 1 | 30 | 60 | 60 |
| 2 | 30 | 60 | 30 |
| 3 | 120 | 60 | 60 |
| 4 | 90 | 90 | 90 |
| 5 | 45 | 45 | 45 |
| 6 | 60 | 120 | 60 |
| 7 | 75 | 15 | 15 |
| 8 | 45 | 45 | 45 |
| 9 | 90 | 90 | 90 |
| 10 | 105 | 75 | 105 |
🤓 Note: Make sure to check your work and use the definitions of complementary and supplementary angles to find the correct answers.
Conclusion
Complementary and supplementary angles are two fundamental concepts in geometry. By understanding the definitions and characteristics of these angles, you can solve problems involving right triangles, straight lines, and angles. We hope this worksheet has helped you practice and reinforce your knowledge of complementary and supplementary angles.
What is the difference between complementary and supplementary angles?
+Complementary angles add up to 90 degrees, while supplementary angles add up to 180 degrees.
Can complementary angles be adjacent?
+Yes, complementary angles can be adjacent (share a common side) or non-adjacent.
What is the sum of two supplementary angles?
+The sum of two supplementary angles is always 180 degrees.
Related Terms:
- Supplementary angle worksheet