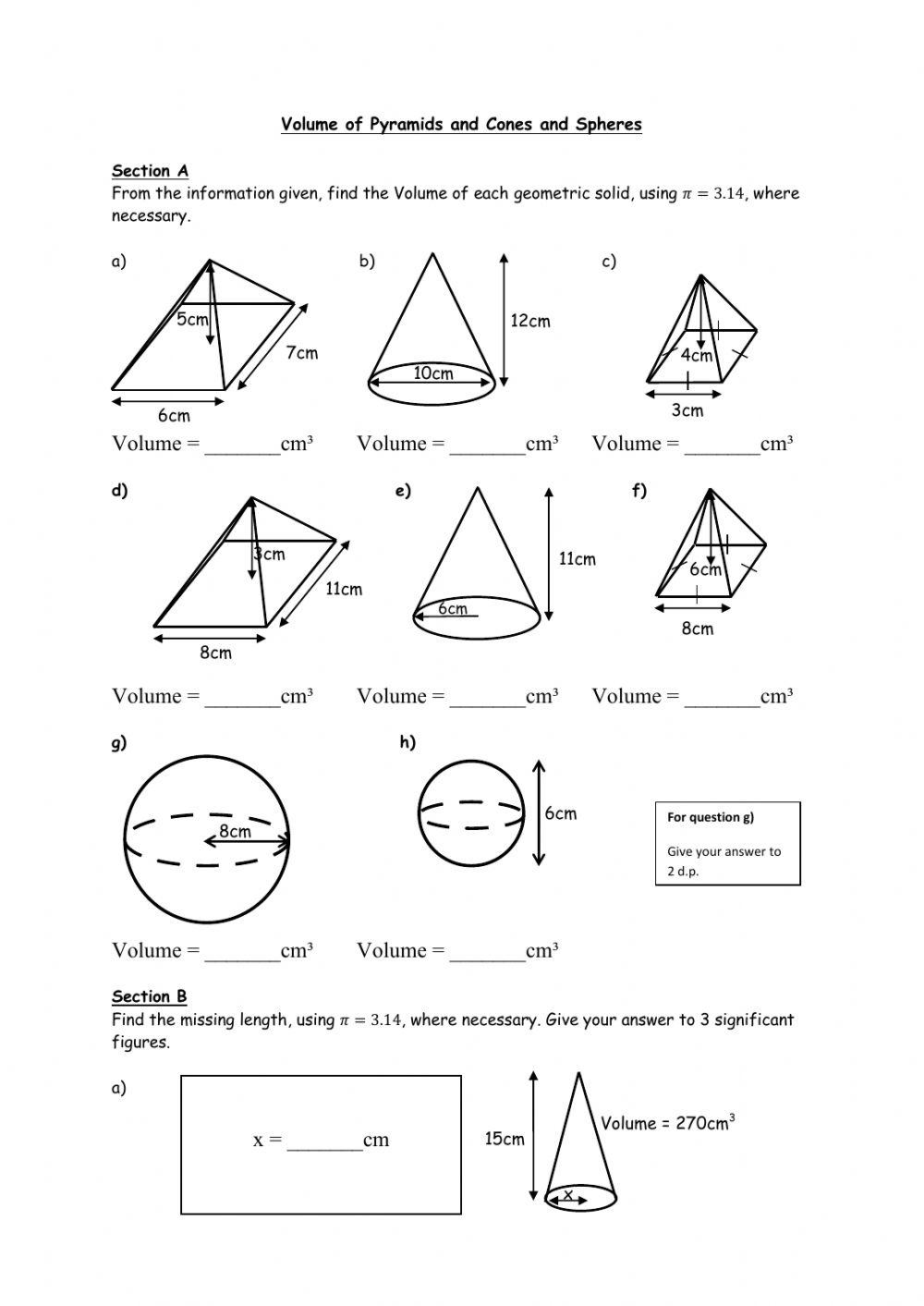
5 Ways to Calculate Pyramid and Cone Volumes
Understanding the Concept of Volumes
Calculating the volume of various shapes is an essential aspect of mathematics and engineering. Two fundamental shapes in geometry are the pyramid and the cone. Both shapes have unique properties and formulas for calculating their volumes. In this article, we will delve into five ways to calculate pyramid and cone volumes, emphasizing the importance of each method.
Method 1: Basic Volume Formula for Pyramids
The basic volume formula for a pyramid is:
Volume = (1⁄3) × Base Area × Height
This formula is applicable to all types of pyramids, including triangular and square-based pyramids.

| Variables | Definitions |
|---|---|
| Volume (V) | The volume of the pyramid |
| Base Area (B) | The area of the pyramid's base |
| Height (h) | The perpendicular height of the pyramid |
📝 Note: The base area must be a flat surface, and the height must be perpendicular to the base.
Method 2: Using Similar Triangles for Cones
When dealing with cones, we can utilize the concept of similar triangles to find the volume. The formula for the volume of a cone is:
Volume = (1⁄3) × π × Radius² × Height
By using similar triangles, we can derive this formula from the basic volume formula for pyramids.
| Variables | Definitions |
|---|---|
| Volume (V) | The volume of the cone |
| π (pi) | A mathematical constant representing the ratio of a circle's circumference to its diameter |
| Radius (r) | The radius of the cone's base |
| Height (h) | The perpendicular height of the cone |
Method 3: Using the Disk Method for Cones
The disk method is an alternative approach to finding the volume of a cone. This method involves integrating the area of infinitesimally thin disks to find the total volume.
Volume = π × ∫a, b² dx
Where:
- f(x) is the function describing the cone’s shape
- a and b are the limits of integration
📝 Note: This method requires a basic understanding of calculus and integration.
Method 4: Using the Shell Method for Pyramids
The shell method is another technique for finding the volume of a pyramid. This method involves integrating the area of infinitesimally thin shells to find the total volume.
Volume = 2π × ∫[a, b] x × f(x) dx
Where:
- f(x) is the function describing the pyramid’s shape
- a and b are the limits of integration
📝 Note: This method requires a basic understanding of calculus and integration.
Method 5: Using Geometric Proofs for Pyramids and Cones
Geometric proofs involve using visual representations and logical reasoning to derive the volume formulas for pyramids and cones.
For example, by dissecting a pyramid into smaller pyramids and cones, we can prove the basic volume formula for pyramids:
Volume = (1⁄3) × Base Area × Height
Similarly, by dissecting a cone into smaller cones and cylinders, we can prove the formula for the volume of a cone:
Volume = (1⁄3) × π × Radius² × Height
In conclusion, calculating the volume of pyramids and cones is an essential aspect of mathematics and engineering. By mastering these five methods, you will gain a deeper understanding of the underlying principles and be able to tackle complex problems with confidence.
What is the main difference between the volume formulas for pyramids and cones?
+The main difference is the shape of the base. Pyramids have a polygonal base, while cones have a circular base.
Which method is more accurate for finding the volume of a cone?
+The disk method is generally more accurate, as it involves integrating the area of infinitesimally thin disks to find the total volume.
What is the importance of understanding the volume formulas for pyramids and cones?
+Understanding the volume formulas for pyramids and cones is crucial in various fields, including architecture, engineering, and physics, as it allows for accurate calculations and designs.