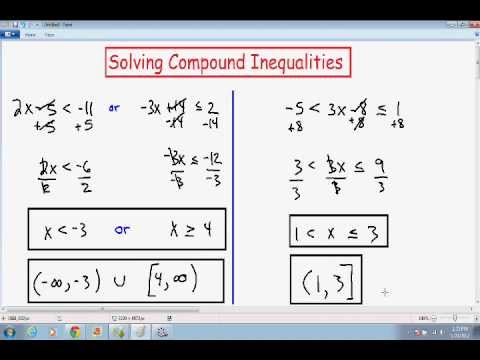
Variables On Both Sides Equations Worksheet

Understanding Variables on Both Sides Equations
Variables on both sides equations are a type of linear equation where the variable appears on both sides of the equation. These equations require careful manipulation to isolate the variable and find the solution. In this article, we will explore the concept of variables on both sides equations, provide examples, and offer a worksheet for practice.
What are Variables on Both Sides Equations?
Variables on both sides equations are linear equations where the variable appears on both sides of the equation. For example:
2x + 3 = x + 5
In this equation, the variable x appears on both sides of the equation. To solve this type of equation, we need to isolate the variable by performing algebraic operations.
How to Solve Variables on Both Sides Equations
To solve variables on both sides equations, we need to follow these steps:
- Add or subtract the same value to both sides of the equation to get all the variable terms on one side.
- Add or subtract the same value to both sides of the equation to get all the constant terms on the other side.
- Divide both sides of the equation by the coefficient of the variable to solve for the variable.
Example 1: Simple Variables on Both Sides Equation
Solve the equation: 2x + 3 = x + 5
Step 1: Subtract x from both sides of the equation to get all the variable terms on one side:
2x - x + 3 = x - x + 5
Step 2: Simplify the equation:
x + 3 = 5
Step 3: Subtract 3 from both sides of the equation to get all the constant terms on the other side:
x + 3 - 3 = 5 - 3
Step 4: Simplify the equation:
x = 2
Example 2: More Complex Variables on Both Sides Equation
Solve the equation: 3x + 2 = 2x + 5
Step 1: Subtract 2x from both sides of the equation to get all the variable terms on one side:
3x - 2x + 2 = 2x - 2x + 5
Step 2: Simplify the equation:
x + 2 = 5
Step 3: Subtract 2 from both sides of the equation to get all the constant terms on the other side:
x + 2 - 2 = 5 - 2
Step 4: Simplify the equation:
x = 3
Variables on Both Sides Equations Worksheet
Now it's your turn to practice solving variables on both sides equations. Here are five equations for you to solve:

| Equation | Solution |
|---|---|
| 2x + 4 = x + 7 | |
| x + 2 = 3x - 1 | |
| 4x - 2 = 2x + 6 | |
| 3x + 1 = 2x + 4 | |
| x - 3 = 2x + 2 |
📝 Note: Remember to follow the steps outlined above to solve each equation. Check your work by plugging your solution back into the original equation.
In conclusion, variables on both sides equations require careful manipulation to isolate the variable and find the solution. By following the steps outlined above and practicing with the worksheet, you’ll become more confident in solving these types of equations.
What is the main goal when solving variables on both sides equations?
+The main goal is to isolate the variable by performing algebraic operations.
What is the first step in solving variables on both sides equations?
+The first step is to add or subtract the same value to both sides of the equation to get all the variable terms on one side.
Why is it important to check your work when solving variables on both sides equations?
+Checking your work by plugging your solution back into the original equation ensures that your solution is correct.