Solving Two-Step Equations with Integers Made Easy

Unlocking the Secrets of Two-Step Equations with Integers

Two-step equations with integers can seem daunting, but with the right approach, they can be solved with ease. In this article, we’ll delve into the world of two-step equations, explore the key concepts, and provide step-by-step guidance on how to solve them.

What are Two-Step Equations?

Two-step equations are algebraic equations that require two operations to solve. They involve a combination of addition, subtraction, multiplication, and division, and can include integers, fractions, or decimals. The goal is to isolate the variable (usually represented by a letter) and find its value.

Key Concepts to Master

Before diving into the solution process, it’s essential to grasp the following concepts:

• Inverse Operations: Understanding the relationship between addition and subtraction, as well as multiplication and division, is crucial. Inverse operations “undo” each other, allowing us to isolate the variable.
• Order of Operations: Following the correct order of operations (PEMDAS/BODMAS) ensures that calculations are performed correctly.
• Integer Properties: Familiarity with integer properties, such as the commutative and associative properties, helps simplify equations.

Step-by-Step Solution Process

Now that we’ve covered the essential concepts, let’s walk through a step-by-step solution process for two-step equations with integers:

Step 1: Simplify the Equation (if necessary)

• Combine like terms
• Remove any parentheses or brackets
• Simplify any complex fractions or decimals

Step 2: Identify the Inverse Operations

• Determine the operations required to isolate the variable
• Identify the inverse operations needed to “undo” each operation

Step 3: Perform the Inverse Operations

• Apply the inverse operations to both sides of the equation
• Simplify the resulting equation

Step 4: Check Your Solution

• Plug the solution back into the original equation to ensure it’s true
• Verify that the solution is reasonable and makes sense in the context of the problem

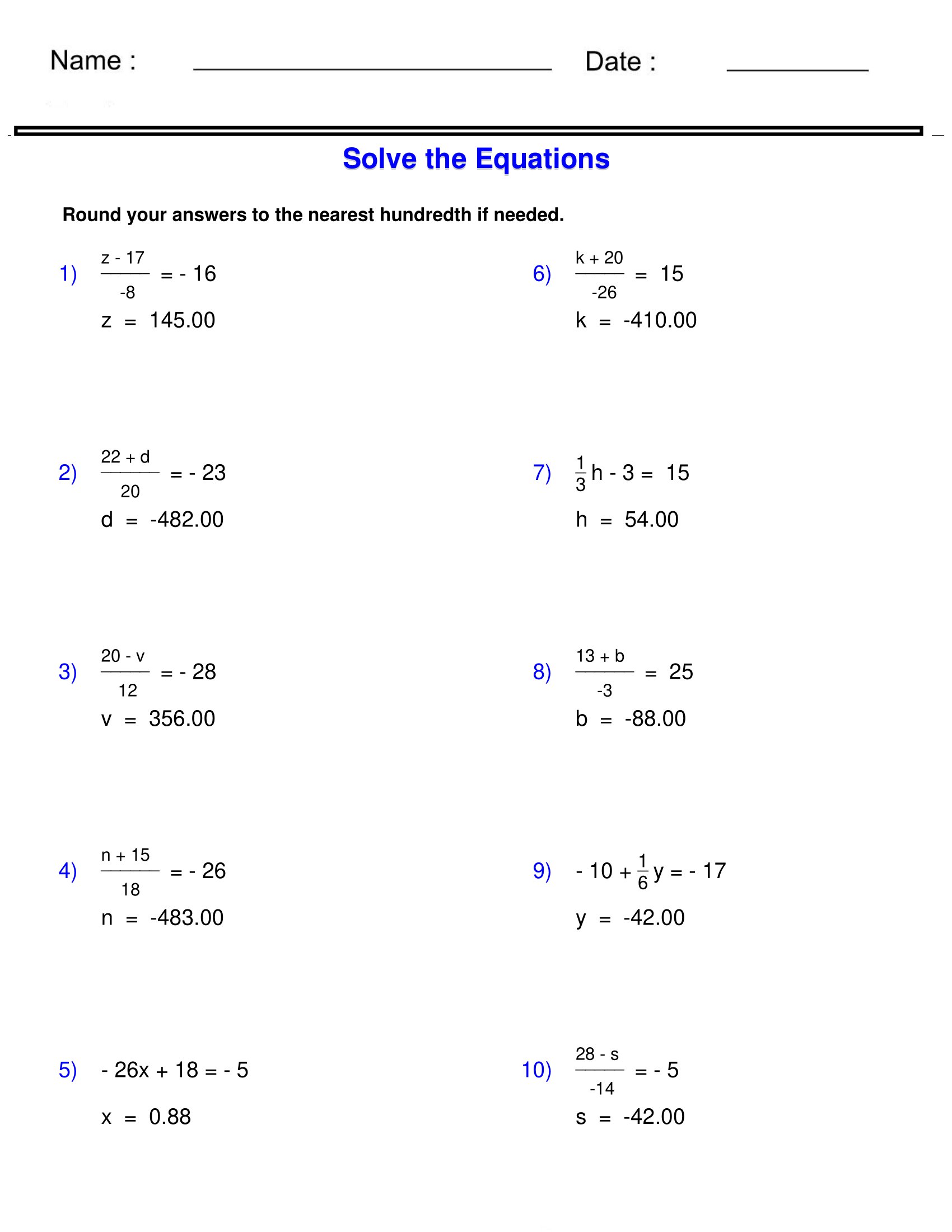
Example 1: Solving a Two-Step Equation with Integers

Let’s solve the equation: 2x + 5 = 11

Step 1: Simplify the Equation

The equation is already simplified.

Step 2: Identify the Inverse Operations

We need to isolate the variable x. To do this, we’ll use the inverse operations of addition and multiplication.

• Inverse operation of addition: subtraction
• Inverse operation of multiplication: division

Step 3: Perform the Inverse Operations

• Subtract 5 from both sides of the equation: 2x = 11 - 5
• Simplify: 2x = 6
• Divide both sides of the equation by 2: x = 6 ÷ 2
• Simplify: x = 3

Step 4: Check Your Solution

• Plug x = 3 back into the original equation: 2(3) + 5 = 11
• Verify that the solution is true: 6 + 5 = 11

Example 2: Solving a Two-Step Equation with Integers

Let’s solve the equation: x/4 - 2 = 7

Step 1: Simplify the Equation

The equation is already simplified.

Step 2: Identify the Inverse Operations

We need to isolate the variable x. To do this, we’ll use the inverse operations of division and subtraction.

• Inverse operation of division: multiplication
• Inverse operation of subtraction: addition

Step 3: Perform the Inverse Operations

• Add 2 to both sides of the equation: x/4 = 7 + 2
• Simplify: x/4 = 9
• Multiply both sides of the equation by 4: x = 9 × 4
• Simplify: x = 36

Step 4: Check Your Solution

• Plug x = 36 back into the original equation: ³⁶⁄₄ - 2 = 7
• Verify that the solution is true: 9 - 2 = 7

📝 Note: When solving two-step equations, it's essential to perform the inverse operations in the correct order. Failure to do so may result in incorrect solutions.

Tips and Tricks

• Read the equation carefully: Understand the problem and identify the variable and constants.
• Use inverse operations wisely: Apply the correct inverse operations to isolate the variable.
• Check your work: Verify that your solution is true by plugging it back into the original equation.
• Practice, practice, practice: The more you practice, the more comfortable you’ll become with solving two-step equations.

Conclusion

Solving two-step equations with integers may seem daunting, but with the right approach, it can be a breeze. By mastering the key concepts, following the step-by-step solution process, and practicing regularly, you’ll become a pro at solving two-step equations in no time.

Related Terms:

• Two step Equations Worksheet
• two-step equations worksheets pdf
• Two-Step Equations Integers sheet 1
• Two step equations with fractions
• Two-step equations with integers calculator